Induced emf and current due to a changing uniform B field
Figure 30-8 shows a conducting loop consisting of a half-circle of radius r = 0.20 m and three straight sections. The halfcircle lies in a uniform magnetic field →B that is directed out of the page, the field magnitude is given by B=4.0t2+2.0t+3.0, with B in teslas and tin seconds. An ideal battery with emf Ebat = 2.0 V is connected to the loop. The resistance of the loop is 2.0 Ω.
(a) What are the magnitude and direction of the emf Eind induced around the loop by field →B at t = 10 s?
(b) What is the current in the loop at t = 10 s?
To solve the problem step by step, we will break it down into two parts as specified in the question.
Part (a): Finding the Induced EMF Eind
1. Identify the expression for the magnetic field: The magnetic field is given by B(t)=4.0t2+2.0t+3.0 (in teslas).
2. Calculate the magnetic field at t=10 seconds:
B(10)=4.0(10)2+2.0(10)+3.0=4.0×100+20+3=400+20+3=423T
3. Determine the area of the semicircular loop: The radius r is given as 0.20m.
A=12πr2=12π(0.20)2=12π(0.04)=0.02πm2
4. Calculate the rate of change of the magnetic field: We need to differentiate B(t) with respect to time t:
dBdt=ddt(4.0t2+2.0t+3.0)=8.0t+2.0
At t=10:
dBdt=8.0(10)+2.0=80+2=82T/s
5. Use Faraday's law of electromagnetic induction to find the induced EMF:
Eind=−AdBdt
Substituting the values:
Eind=−0.02π×82
Approximating π≈3.14:
Eind≈−0.02×3.14×82≈−5.2V
6. Determine the direction of the induced EMF: Since the magnetic field is increasing out of the page, by Lenz's law, the induced EMF will act in a direction to oppose this increase, which means the induced current will flow in a direction that creates a magnetic field into the page.
Part (b): Finding the Current in the Loop
1. Identify the battery EMF and resistance: The battery EMF Ebat=2.0V and the resistance R=2.0Ω.
2. Calculate the net EMF in the loop:
Enet=Ebat−|Eind|=2.0−5.2=−3.2V
Since the induced EMF opposes the battery, we take its absolute value.
3. Calculate the current using Ohm's law:
I=EnetR=−3.22.0=−1.6A
The negative sign indicates that the current is flowing in the direction opposite to what we assumed when applying the battery EMF.
Final Results
- Induced EMF Eind: 5.2V (out of the page)
- Current I: 1.6A (in the direction opposite to the battery EMF)
B(10)=4.0(10)2+2.0(10)+3.0=4.0×100+20+3=400+20+3=423T
A=12πr2=12π(0.20)2=12π(0.04)=0.02πm2
dBdt=ddt(4.0t2+2.0t+3.0)=8.0t+2.0
At t=10:
dBdt=8.0(10)+2.0=80+2=82T/s
Eind=−AdBdt
Substituting the values:
Eind=−0.02π×82
Approximating π≈3.14:
Eind≈−0.02×3.14×82≈−5.2V
Enet=Ebat−|Eind|=2.0−5.2=−3.2V
Since the induced EMF opposes the battery, we take its absolute value.
I=EnetR=−3.22.0=−1.6A
The negative sign indicates that the current is flowing in the direction opposite to what we assumed when applying the battery EMF.
- Induced EMF Eind: 5.2V (out of the page)
- Current I: 1.6A (in the direction opposite to the battery EMF)
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - CHECKPOINTS9videos
ELECTROMAGNETIC INDUCTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - PROBLEMS67videos
ELECTROMAGNETIC INDUCTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - (PRACTICE QUESTIONS) Integer Type1videos
ELECTRIC POTENTIAL
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC OSCILLATIONS AND ALTERNATING CURRENT EXERCISE - Practice Questions (Integer)7videos
ELECTROMAGNETIC OSCILLATIONS AND ALTERNATING CURRENT
Similar Questions
Induced emf due to a changing nonuniform B field
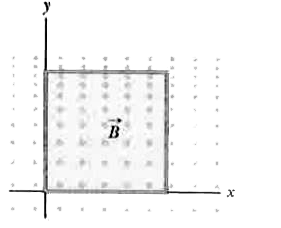
Figure 30-9 shows a rectangular loop of wire immersed in a nonuniform and varying magnetic field →B that is perpendicular to and directed into the page. The field's magnitude is given by B=4t2x2, with B in teslas, tin seconds, and x in meters. (Note that the function depends on both time and position.) The loop has width W = 3.0 m and height H = 2.0 m. What are the magnitude and direction of the induced emf E around the loop at t = 0.10 s?View SolutionAs seen in Fig. 30-47, a square loop of wire has sides of length 3.0 cm. A magnetic field is directed out of the page, its magnitude is given by B=5.0t2y , where B is in teslas, t is in seconds, and y is in meters. At t = 2.5 s, what are the (a) magnitude and (b) direction of the emf induced in the loop?
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A conducting circular loop of radiius r carries a constant current i. It is placed in a uniform magnetic field →B0 such that →B0 is perpendicular to the plane of the loop . The magnetic force acting on the loop is
AirB0B2πrB0CzeroDπirB0 - Question 2 - Select One
A conducting circular loop of radiius r carries a constant current i. It is placed in a uniform magnetic field →B0 such that →B0 is perpendicular to the plane of the loop . The magnetic force acting on the loop is
AirB0B2πirB0CzeroDπirB0 - Question 3 - Select One
A copper disc of radius 0.1m rotates about its centre with 10 revolutuion per second in a uniform magnetic field of 0.1 tesla. The emf induced across the radius of the disc is
Aπ10VB2π10VC10πmVD20πmV
If plane of coil and uniform magnetic field B(4T0 is same then torque on the current carrying coil is
 View Solution
View SolutionA copper disc of radius 0.1 m rotates about its centre with 10 revolution per second in 'a uniform magnetic field of 0.1 T. The emf induced across the radius of the disc is -
View SolutionA square loop of side 22cm is converted into circular loop in 0.4s. A uniform magnetic field of 0.2Tdirected normal to the loop, then the induced in the loop is
View SolutionA loop shown in the figure is immersed in the varying magnetic field B=B0t, directed into the page. If the total resistance of the loop is R, then the direction and magnitude of induced current in the inner circle is
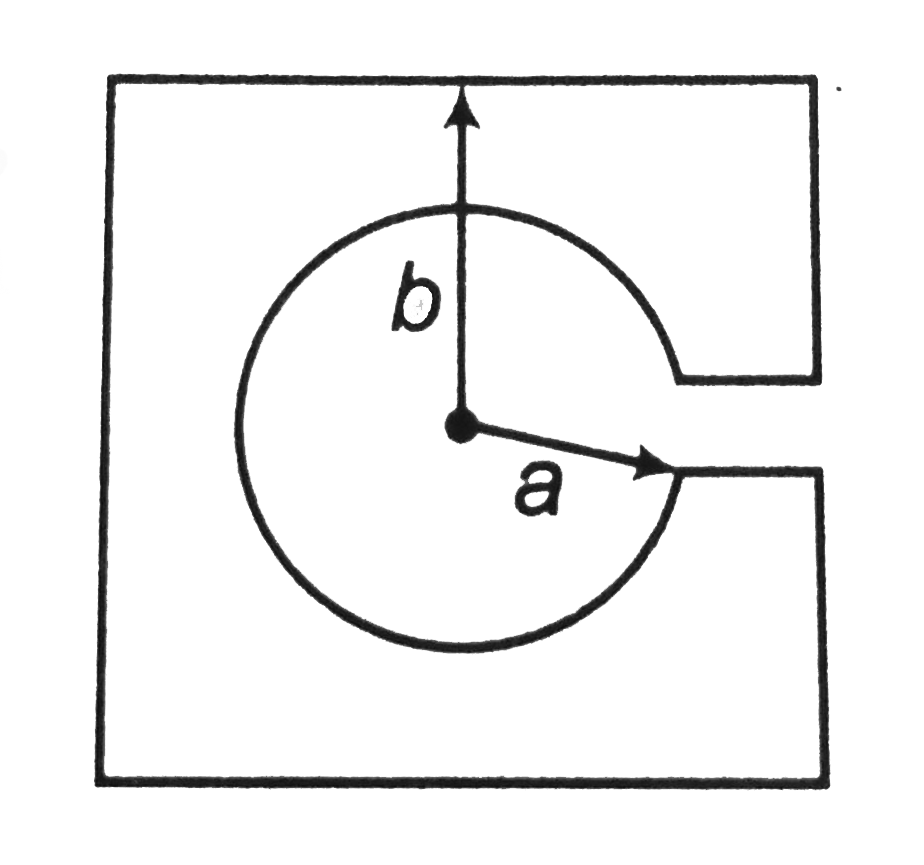 View Solution
View SolutionIn the figure shown a semicircular wire loop is placed in a uniform magnetic field B=1.0T. The plane of the loop is perpendicular to the magnetic field. Current i=2A flows in the loop in the directions shown. Find the magnitude of the magnetic force in newton in both the cases a and b. The radius of the loop is 1.0 m
 View Solution
View Solution

