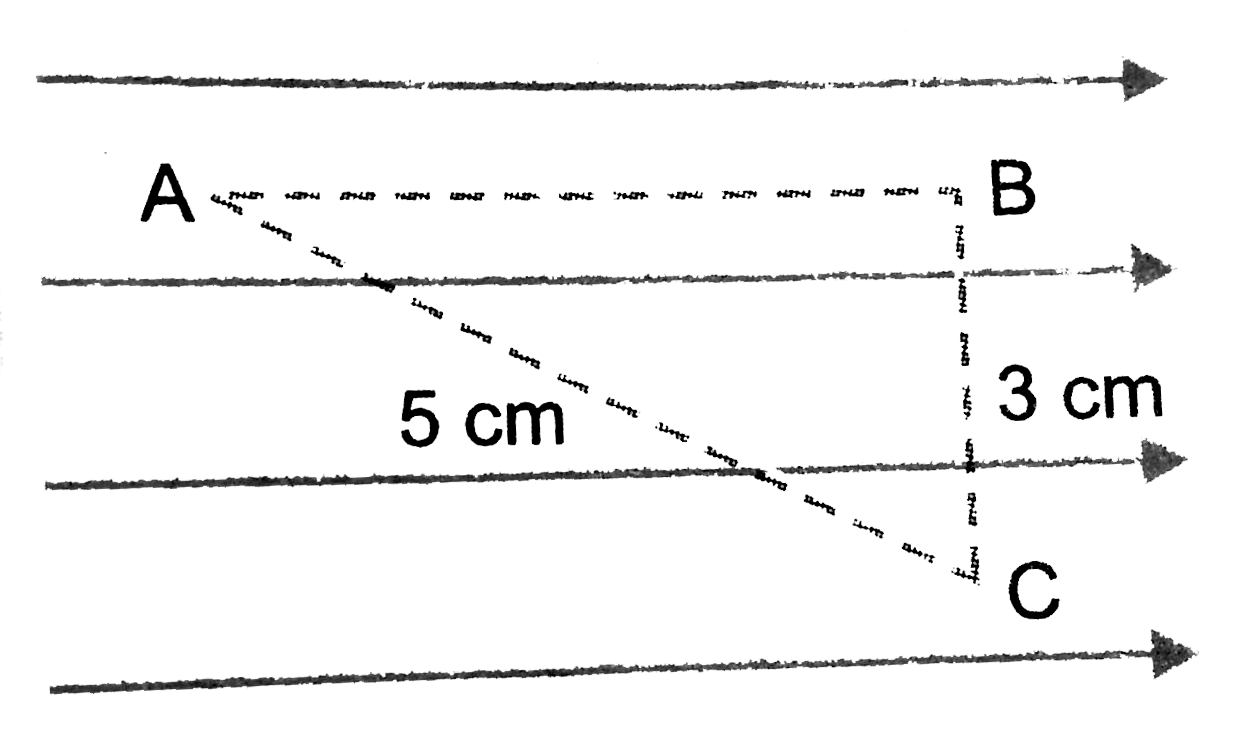
In Fig. 36-10, Sally (at point A) and Sam's spaceship (of proper length $L_{0} = 230 m$ ) pass each other with constant relative speed v. Sally measures a time interval of 3.57 $μ s$ for the ship to pass her (from the passage of point B in Fig. 36-10a to the passage of point C in Fig. 36-10b). In terms of c, what is the relative speed v between Sally and the ship?

Text Solution

Verified by Experts

KEY IDEAS
Let.s assume that speed v is near c. Then:
1. This problem involves measurements made from two (inertial) reference frames, one attached to Sally and the other attached to Sam and his spaceship.
2. This problem also involves two events: the first is the passage of point B pass Sally (Fig. 36-10a) and the second is the passage of point C past her (Fig. 36-10b).
4. From either reference frame, the other reference frame passes at speed v and moves a certain distance in the time interval between the two events:
$v = \frac{distance}{time interval}$ (36-17)
Because speed v is assumed to be near the speed of light, We must be careful that the distance and the time interval in Eq. 36-17 are measured in the same reference frame.
Calculations: We free to use either frame for the measurements. Because we know that the time interval $Δ t$ between the two events measured from Sally.s frame is 3.57 $μ s$ , let us also use the distance L between the two events measured from her frame. Equation 36-17 then becomes
$v = \frac{L}{Δ t} (36 - 18)$
We do not know L, but we can relate it to the given $L_{0}$ . The distance between the two events as measured from Sam.s frame is the ship.s proper length $L_{0}$ . Thus, the distance L measured from Scally.s frame must be less than $L_{0}$ , as given by Eq. 36-13 $(L = L_{0} / γ)$ for length contraction. Substituting $L_{0} / γ$ for L in Eq. 36-18 and then substituting Eq. 36-8 for $γ$ , we find
$v = \frac{L_{0} / γ}{Δ t} = \frac{L_{0} \sqrt{(1 - {(v / c)}^{2})}}{Δ t}$
Solving this equation for v (notice that it is on the left and also buried in the Lorentz factor) leads us to
$v = \frac{L_{0} c}{\sqrt{{(c Δ t)}^{2} + L_{0}^{2}}}$
$= \frac{(230 m) c}{\sqrt{{(299792458 m / s)}^{2} {(3.57 \times 10^{- 6} s)}^{2} + {(230 m)}^{2}}}$
$= 0.210 c$ .
Note that only the relative motion of Sally and Sam matters here, whether either is stationary relative to, say, a space station is irrelevant. In Figs. 36-10a and b we took Sally to be stationary, but we could instead have taken the ship to be stationary, with Sally moving to the left past it. Event 1 is again when Sally and point B are aligned (Fig. 36-10c), and event 2 is again when Sally and point C are aligned (Fig 36-10d). However, we are now using Sam.s measurements. So the length between the two events in his frame is the proper length $L_{0}$ of the ship and the time interval between them is not Sally.s measurement $Δ t$ but a dilated time interval $γ Δ t$ .
Substituting Sam.s measurements into Eq. 36-17, we have
$v = \frac{L_{0}}{γ Δ t}$ ,
which is exactly what we found using Sally.s measurements. Thus, we get the same result of v = 0.210c with either set of measurements, but we must be careful not to mix the measurements from the two frames.

Updated on:21/07/2023