A förce of6 N and another of 8 N can be applied together to produce the effect of asingle force oF (A) I N (B) 11 N (C) 15 N (D) 20 N
Step by step video & image solution for A förce of6 N and another of 8 N can be applied together to produce the effect of asingle force oF (A) I N (B) 11 N (C) 15 N (D) 20 N by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Similar Questions
Knowledge Check
- Question 1 - Select One
A force of 60 N acting perpendicular to a force of 80 N can produce
A20 NB70 NC100 ND140 N - Question 2 - Select One
Forces are applied on a wheel of radius 20 cm as shown in the figure. The torque produced by the forces 4 N at A, 8N at B, 6 N at C and 9N at D at angles indicated is
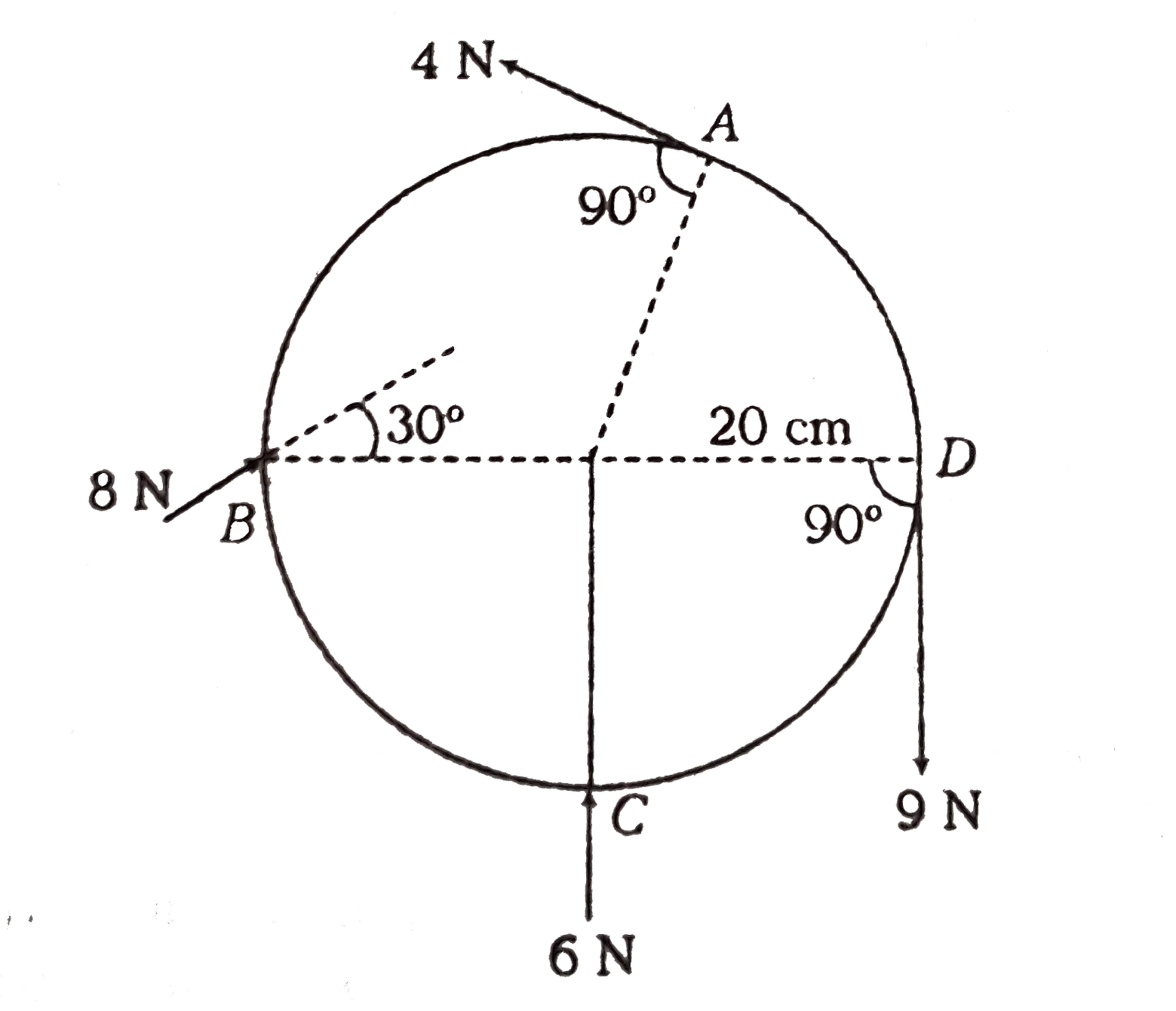 A5.4 N-m anti-clockwiseB1.80 N-m clockwiseC2.0 N-m clockwiseD3.6 N-m clockwise
A5.4 N-m anti-clockwiseB1.80 N-m clockwiseC2.0 N-m clockwiseD3.6 N-m clockwise - Question 3 - Select One
A wheel of radius 20 cm has forces applied to it as shown in the figure. The torque produced by the forces 4N at A, 8N at B, 6N at C, and 9N at D at angles indicated is :
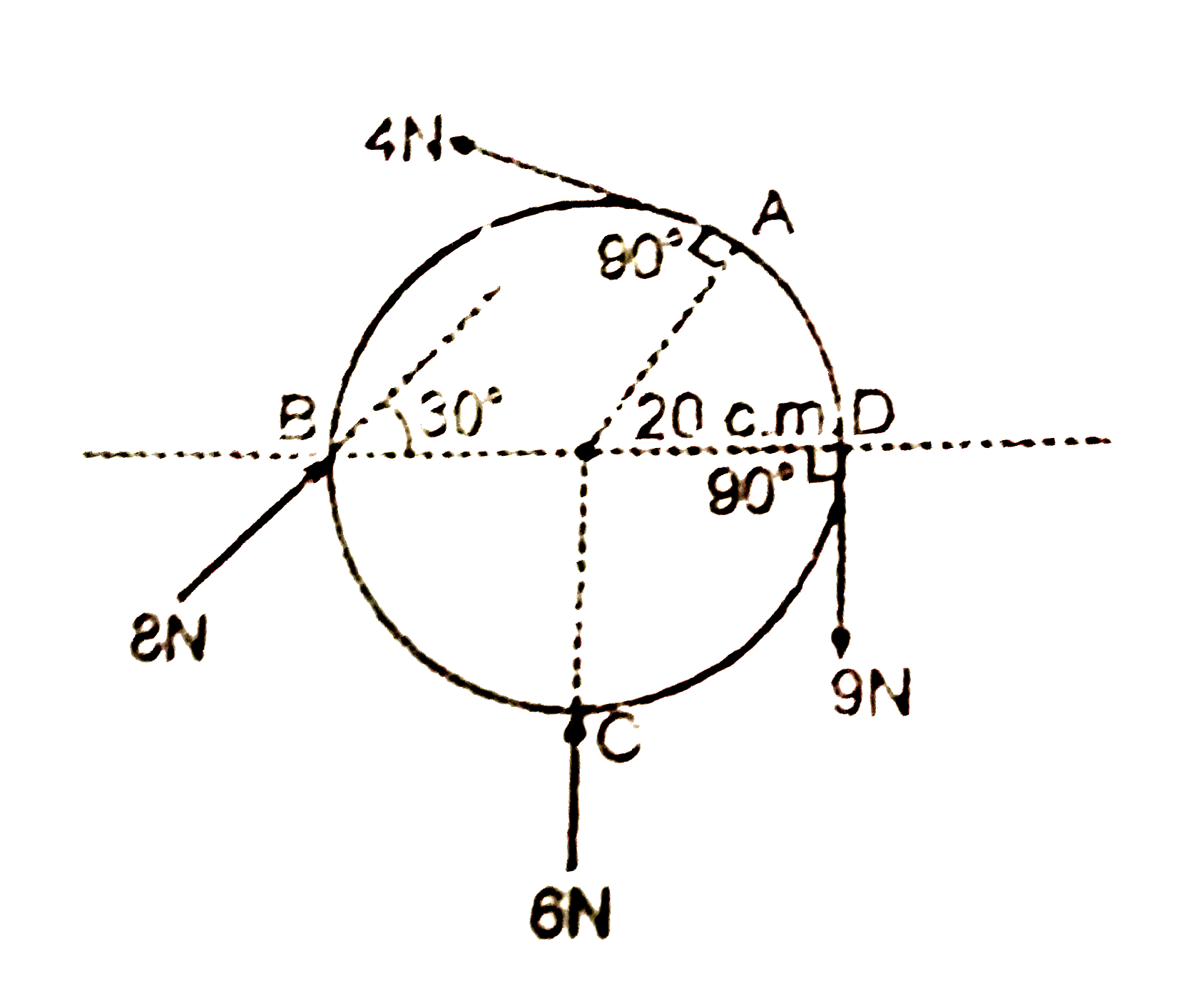 A5.4 N-m AnticlockwiseB1.8 N-m ClockwiseC2.0 N-m ClockwiseD5.4 N-m Clockwise
A5.4 N-m AnticlockwiseB1.8 N-m ClockwiseC2.0 N-m ClockwiseD5.4 N-m Clockwise
Forces of magnitudes 6N and 4N are acting on the body . Which of the following of the following can be the resultant of the two?
(a) 11N (b) 2N (c) 10N (d) 1N (e) 8N (f) 0N (g) 7NView SolutionThe sum of the magnitudes of two forces acting at a point is 16 N. The resultant of these forces is perpendicular to the smaller force has a magnitude of 8 N. If the smaller force is magnitude x, then the value of x is (A) 2N (B) 4N (C) 6N (D) 7N
View SolutionForces are applied on a wheel of radius 20 cm as shown in the figure. The torque produced by the forces 4 N at A, 8N at B, 6 N at C and 9N at D at angles indicated is
 View Solution
View SolutionTo make two bodies A and B experience an equal acceleration, forces 6 N and 4 N are applied, respectively. If the bodies are combined, then to produce the same acceleration, the force applied should be _______ N.
View SolutionIf n(A)=6 and n(B)=8 and n(A∩B)=4, then find n(AΔB).
The following are the step involved in solving the above problem . Arrange them in sequnential order.
(a) n(A∪B)=n(A)+n(B)−n(A∩B)
(b) n(A∪B)=10
(c )n(A∪B)=6+8−4
(d) n(AΔB)=10−4=6
(e) n(AΔB)=n(A∪B)−n(A∩B).View Solution

