Match the unit cell (column I) with number of atoms per unit cell (column II)

A
(i) - (D), (ii) - (C) , (iii) - (B), (iv) - (A)
B
(i) - (C), (ii) - (D) , (iii) - (B), (iv) - (A)
C
(i) - (C), (ii) - (D) , (iii) - (A), (iv) - (B)
D
(i) - (B), (ii) - (D) , (iii) - (C), (iv) - (A)
Video Solution
Text Solution
The correct Answer is:C
|
Answer
Step by step video, text & image solution for Match the unit cell (column I) with number of atoms per unit cell (column II) by Chemistry experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - SOLID STATEEXERCISE - REVISION EXERCISES (OBJECTIVE QUESTIONS) (PASSAGE BASED QUESTIONS)10videos
SOLID STATE
- BOOK - MODERN PUBLICATIONCHAPTER - SOLID STATEEXERCISE - REVISION EXERCISES (OBJECTIVE QUESTIONS) (ASSERTION REASON QUESTIONS)10videos
SOLID STATE
- BOOK - MODERN PUBLICATIONCHAPTER - SOLID STATEEXERCISE - REVISION EXERCISES (OBJECTIVE QUESTIONS) (MULTIPLE CHOICE QUESTIONS)39videos
SOLID STATE
- BOOK - MODERN PUBLICATIONCHAPTER - POLYMERSEXERCISE - Competition file (OBJECTIVE TYPE QUESTIONS) (C. MULTIPLE CHOICE QUESTIONS)(Integer Type Questions)6videos
POLYMERS
- BOOK - MODERN PUBLICATIONCHAPTER - SOLUTIONSEXERCISE - UNIT PRACTICE TEST14videos
SOLUTIONS
Similar Questions
Knowledge Check
- Question 1 - Select One
What is the number of atoms per unit cell in a body centred cubic structure ?
A9B4C2D1 - Question 2 - Select One
Match the unit cell (column I) with radius of its atoms in terms of 'a' (column II)
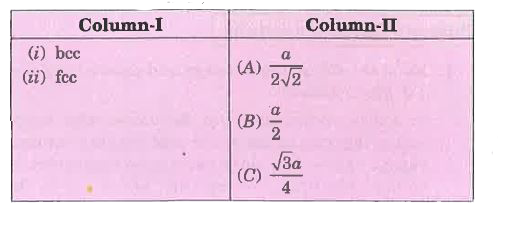 A(i) - (C) - (ii) - (A)B(i) - (C) , (ii) - (B)C(i) - (B) , (ii) - (A)D(i) - (A), (ii) - (B)
A(i) - (C) - (ii) - (A)B(i) - (C) , (ii) - (B)C(i) - (B) , (ii) - (A)D(i) - (A), (ii) - (B) - Question 3 - Select One
The number of atoms per unit cell in bcc lattice is
A1B2C4D9
match the type of unit cell given column I with the features iven in Column II .
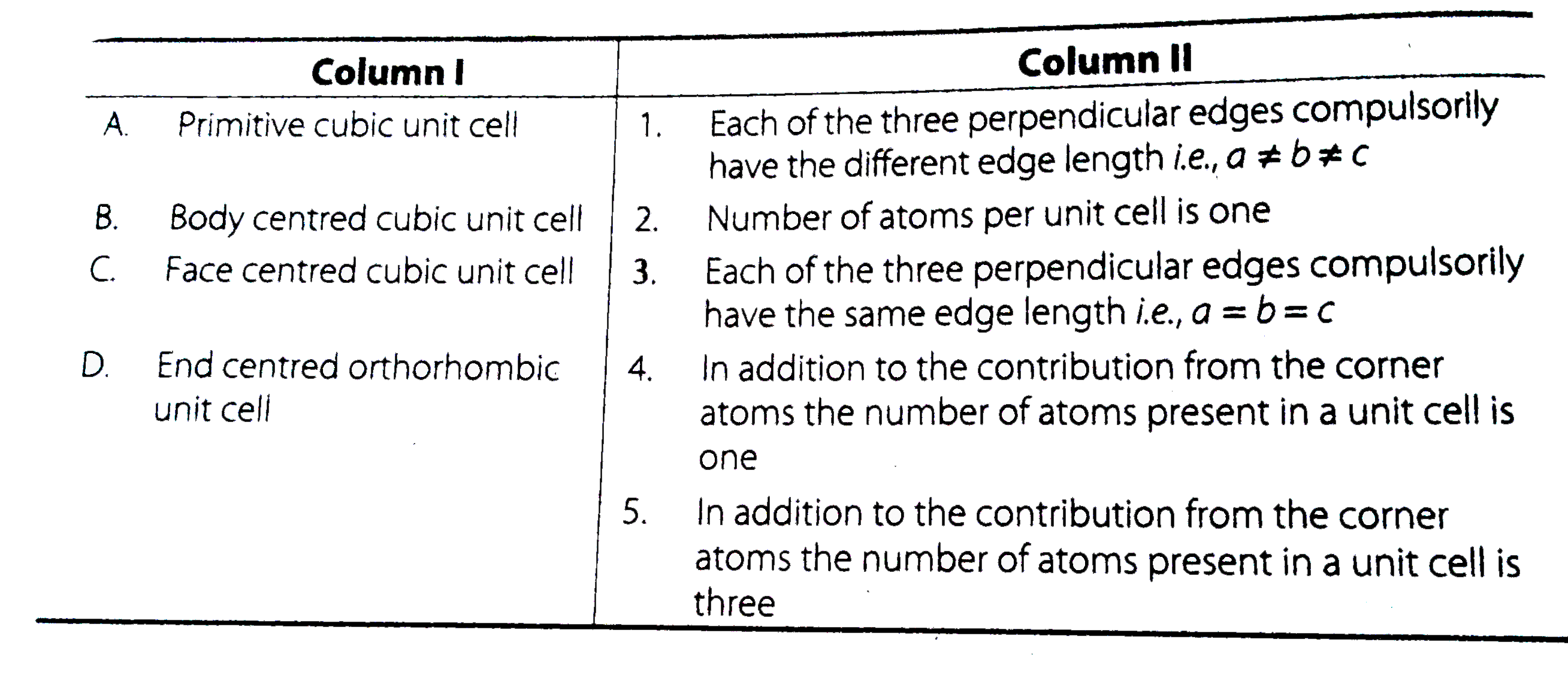 View Solution
View SolutionMatch the column I and column II.
 View Solution
View SolutionMatch column I with column II
 View Solution
View SolutionThe number of atoms per unit cell in bcc lattice is
View SolutionThe number of atoms per unit cell in a face-centred cube is:
View Solution


