On a straight road, two cars X and Y are moving in the same direction, Velocity of car X is 20m/s and that of car Y is15 m/s. Find the velocity of X relative to Y and vice versa.
To solve the problem of finding the velocity of car X relative to car Y and vice versa, we can follow these steps:
Step 1: Understand the concept of relative velocity
Relative velocity is defined as the velocity of one object as observed from another object. If we have two objects A and B, the relative velocity of A with respect to B (denoted as VA/B) can be calculated using the formula:
VA/B=VA−VB
where VA is the velocity of object A and VB is the velocity of object B.
Step 2: Identify the velocities of the cars
From the problem statement, we know:
- Velocity of car X, VX=20m/s
- Velocity of car Y, VY=15m/s
Step 3: Calculate the velocity of car X relative to car Y
Using the formula for relative velocity:
VX/Y=VX−VY
Substituting the known values:
VX/Y=20m/s−15m/s
VX/Y=5m/s
Step 4: Calculate the velocity of car Y relative to car X
Now, we calculate the velocity of car Y relative to car X using the same formula:
VY/X=VY−VX
Substituting the known values:
VY/X=15m/s−20m/s
VY/X=−5m/s
Step 5: Interpret the results
- The velocity of car X relative to car Y is 5m/s in the direction of motion (positive x-direction).
- The velocity of car Y relative to car X is −5m/s, which indicates that car Y is moving at 5m/s in the opposite direction relative to car X.
Final Answers:
- Velocity of X relative to Y: 5m/s
- Velocity of Y relative to X: −5m/s
---
Relative velocity is defined as the velocity of one object as observed from another object. If we have two objects A and B, the relative velocity of A with respect to B (denoted as VA/B) can be calculated using the formula:
VA/B=VA−VB
where VA is the velocity of object A and VB is the velocity of object B.
From the problem statement, we know:
- Velocity of car X, VX=20m/s
- Velocity of car Y, VY=15m/s
Using the formula for relative velocity:
VX/Y=VX−VY
Substituting the known values:
VX/Y=20m/s−15m/s
VX/Y=5m/s
Now, we calculate the velocity of car Y relative to car X using the same formula:
VY/X=VY−VX
Substituting the known values:
VY/X=15m/s−20m/s
VY/X=−5m/s
- The velocity of car X relative to car Y is 5m/s in the direction of motion (positive x-direction).
- The velocity of car Y relative to car X is −5m/s, which indicates that car Y is moving at 5m/s in the opposite direction relative to car X.
- Velocity of X relative to Y: 5m/s
- Velocity of Y relative to X: −5m/s
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - PRACTICE PROBLEMS28videos
MOTION IN A STRAIGHT LINE
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - CONCEPTUAL QUESTIONS14videos
MOTION IN A STRAIGHT LINE
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A PLANE EXERCISE - Chapter Practice Test15videos
MOTION IN A PLANE
- BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Practice Test (For Board Examination)12videos
OSCILLATIONS
Similar Questions
A car (A) is moving at 60kmh−1 on a straight road, is ahead of car (B) moving in the same direction at 10ms−1. Find the velocity of (A) relative to (B) and vice versa.
View SolutionFour cars are moving along a straight road in the same direction. Velocity of car 1 is 10m/s. It was found that distance between car 1 and 2 is decreasing at a rate of 2m/s, whereas driver in car 4 observed that he was nearing car 2 at a speed of 8m/s. The gap between car 2 and 3 is decreasing at a rate of 3m/s.
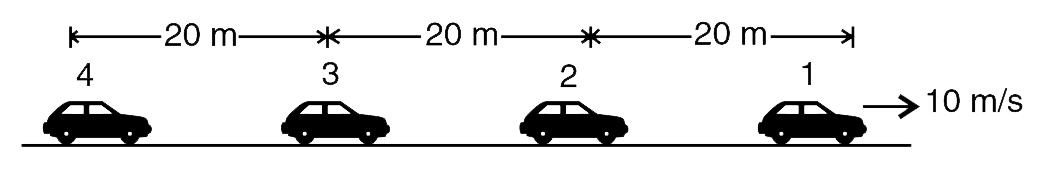
(a) t=0, after how much time t0 will the driver of car 2 see for the first time, that another car overtakes him?
(b) Which car will be first to overtake car 1?View Solution
Knowledge Check
- Question 1 - Select One
Two cars A and B are moving in same direction with velocities 30 m/s and 20 m/s. When car A is at a distance d behind the car B, the driver of the car A applies brakes producing uniform retardation of 2m/s2. There will be no collision when :-
Ad<2.5mBd<125mCd>25mDd>125m - Question 2 - Select One
Out of the two cars A and B car A is moving towards east with velocity of 10 m/s whereas B is moving towards north with a velocity 20 m/s, then velocity of A w.r.t B is ( nearly )
A30 m/sB10 m/sC22 m/sD42 m/s
Two cars are moving in same direction with speed of 40kmh−1 Find the relative velocity of first car with another.
View SolutionThe car A of mass 1000 kg travelling at 25m/s collides with another car B of mass 1500 kg moving with a speed of 20 m/s in the same direction, the velocity of car A becomes 15 m/s, what would be the velocity of car B ?
View SolutionA car of mass one metric ton travelling at 32m/sc dashes into the rear of a truck of mass 8000kg moving in the same direction with a velocity of 4m/s After the collision the car bounces back wards with a velocity of 8m/s What is the velocity of the truck after the impact ?
View SolutionA car travelling with a velocity of 50kmh−1 on a straight road is ahead of a motor-cycle travelling with a speed of 75kmh−1. How would the relative velocity be altered if motor cycle is ahead of car ?
View SolutionThe car A of mass 1500kg, travelling at 25m/s collides with another car B of mass 1000kg travelling at 15m/s in the same direction. After collision, the velocity of car A becomes 20m/s. Calcualte the velocity of car B afrer collison.
View SolutionThe car A of mass 1500 kg, travelling at 25 m/s collides with another car B of mass 1000 kg travelling at 15 m/s in the same direction. After collision the velocity of car A becomes 20 m/s. Calculate the velocity of car B after the collision.
 View Solution
View Solution

