The pilot landing or taking off the aero plane is an inertial observer or non - inertial frame of refrence.
To determine whether the pilot landing or taking off an airplane is an inertial observer or in a non-inertial frame of reference, we can analyze the situation step by step.
1. Understanding Frames of Reference:
- A frame of reference is considered inertial if it is either at rest or moving at a constant velocity. In such frames, Newton's laws of motion hold true without any modifications.
- A non-inertial frame is one that is accelerating. In these frames, fictitious forces (pseudo forces) must be introduced to apply Newton's laws.
2. Analyzing the Pilot's Situation:
- When a pilot is landing or taking off, the airplane undergoes a change in velocity. This change in velocity indicates that the airplane is accelerating.
- During landing, the airplane decelerates, while during takeoff, it accelerates. In both cases, there is a change in speed.
3. Acceleration and Frame of Reference:
- Since the airplane is accelerating (either speeding up or slowing down), the frame of reference of the pilot inside the airplane is not inertial.
- An observer inside an accelerating frame experiences forces that are not due to any physical interaction but rather due to the acceleration of the frame itself.
4. Conclusion:
- The pilot, being in an accelerating airplane (whether during takeoff or landing), is in a non-inertial frame of reference.
- Therefore, the pilot is classified as a non-inertial observer.
Final Statement:
The pilot landing or taking off the airplane is in a non-inertial frame of reference due to the acceleration of the airplane.
---
- A frame of reference is considered inertial if it is either at rest or moving at a constant velocity. In such frames, Newton's laws of motion hold true without any modifications.
- A non-inertial frame is one that is accelerating. In these frames, fictitious forces (pseudo forces) must be introduced to apply Newton's laws.
- When a pilot is landing or taking off, the airplane undergoes a change in velocity. This change in velocity indicates that the airplane is accelerating.
- During landing, the airplane decelerates, while during takeoff, it accelerates. In both cases, there is a change in speed.
- Since the airplane is accelerating (either speeding up or slowing down), the frame of reference of the pilot inside the airplane is not inertial.
- An observer inside an accelerating frame experiences forces that are not due to any physical interaction but rather due to the acceleration of the frame itself.
- The pilot, being in an accelerating airplane (whether during takeoff or landing), is in a non-inertial frame of reference.
- Therefore, the pilot is classified as a non-inertial observer.
The pilot landing or taking off the airplane is in a non-inertial frame of reference due to the acceleration of the airplane.
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - TOUGH & TRICKY ( PROBLEMS)
10 Videos
- MOTION IN A STRAIGHT LINE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - NCERT (TEXTBOOK EXERCISES )
22 Videos
- MOTION IN A STRAIGHT LINE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - PRACTICE PROBLEMS
28 Videos
- MOTION IN A PLANE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A PLANE EXERCISE - Chapter Practice Test
15 Videos
- OSCILLATIONS BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Practice Test (For Board Examination)
12 Videos
Similar Questions
Knowledge Check
Non-inertial frame of reference have
Azero acceleration.Bsome acceleration with respect to inertial frame of reference.Czero velocity.Dconstant velocity with respect to inertial frame of reference.A collision of two objects occurs in an inertial frame of reference A. It is also observed by an observed in another inertial frame of reference B moving uniformly with respect to the frame A along the z-axis. Which one of the following is correct?
AThe momentum is conserved in both the frames but the energy may not.BThe energy is conserved in both the frames but the momentum may not.CMomentum along the z-axis only is conserved in the two frames.DBoth energy and momentum are conserved in both the frames.Which law of Newton defines an ‘inertial frame of reference’ ?
AFirst law of motionBSecond law of motionCThird law of motionDLaw of gravitation
Define inertial frame and non-inertial frame. Is earth an inertial frame of reference?
View SolutionFind the expression for the density of a body in an arbitrary inertial frame of reference.
View SolutionWhich law of Newton defines an 'inertial frame of reference' ? .
View SolutionStatement-I : Time period of spring block system is the same whether in an accelerated or in an inertial frame of reference.
Statement-II : Mass of the block of spring block system and spring constant of the spring are independent of the acceleration of the frame of reference.
View SolutionA frame of reference that is accelerated with respect to an inertial frame of reference is called a non-inertial frame of reference. A coordinate system fixed on a circular disc rotating about a fixed axis with a constant angular velocity ω is an example of non=inertial frame of reference. The relationship between the force →Frot experienced by a particle of mass m moving on the rotating disc and the force →F∈ experienced by the particle in an inertial frame of reference is
→Frot=→Fin+2m(→vrot×→ω)+m(→ω×→r)×→ω.
where →vrot is the velocity of the particle in the rotating frame of reference and →r is the position vector of the particle with respect to the centre of the disc.
Now consider a smooth slot along a diameter fo a disc of radius R rotating counter-clockwise with a constant angular speed ω about its vertical axis through its center. We assign a coordinate system with the origin at the center of the disc, the x-axis along the slot, the y-axis perpendicular to the slot and the z-axis along the rotation axis (→ω=ωˆk). A small block of mass m is gently placed in the slot at →r(R/2)ˆi at t=0 and is constrained to move only along the slot.
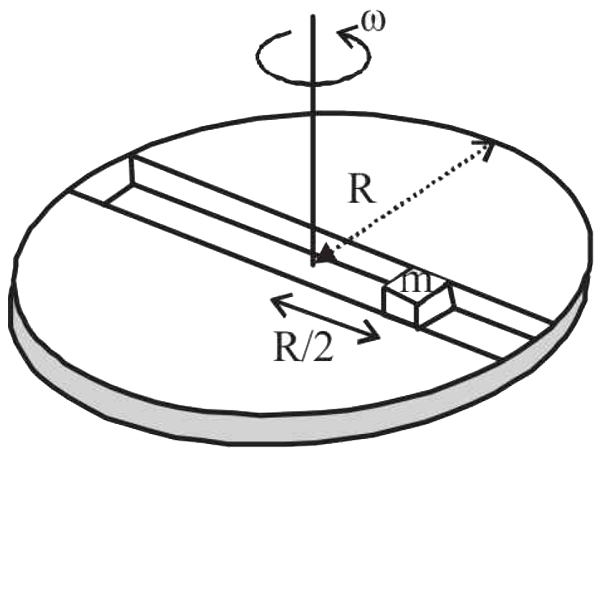
The distance r of the block at time is
View Solution
