A football kicker can give the ball an initial speed of 25 m/s. What are the (a) least and (b) greatest elevation angles at which he can kick the ball to score a field goal from a point 50 m in front of goalposts whose horizontal bar is 3.44 m above the ground?
To solve the problem, we need to determine the least and greatest elevation angles at which a football kicker can kick the ball to score a field goal. We will use the equations of motion in two dimensions, particularly focusing on projectile motion.
1. Identify Given Values:
- Initial speed of the ball, u=25m/s
- Horizontal distance to the goalposts, x=50m
- Height of the goalpost, h=3.44m
- Acceleration due to gravity, g=10m/s2
2. Equation of Motion:
The trajectory of a projectile can be described by the equation:
y=xtan(θ)−gx22u2cos2(θ)
where y is the height of the projectile at horizontal distance x, and θ is the angle of projection.
3. Substituting Known Values:
We want the ball to reach a height h at distance x:
3.44=50tan(θ)−10⋅5022⋅252cos2(θ)
4. Simplifying the Equation:
First, calculate 10⋅5022⋅252:
=10⋅25002⋅625=250001250=20
Thus, the equation simplifies to:
3.44=50tan(θ)−20sec2(θ)
5. Rearranging the Equation:
Rearranging gives:
20sec2(θ)−50tan(θ)+3.44=0
Using the identity sec2(θ)=1+tan2(θ), we can substitute t=tan(θ):
20(1+t2)−50t+3.44=0
This expands to:
20t2−50t+23.44=0
6. Using the Quadratic Formula:
The quadratic formula is given by:
t=−b±√b2−4ac2a
Here, a=20, b=−50, and c=23.44:
t=50±√(−50)2−4⋅20⋅23.442⋅20
Calculate the discriminant:
=2500−1875.2=624.8
Now, substituting back:
t=50±√624.840
7. Calculating the Values:
Calculate √624.8≈24.99:
t1=50+24.9940≈1.87475andt2=50−24.9940≈0.62525
8. Finding Angles:
Now convert t back to angles:
θ1=tan−1(1.875)≈62∘andθ2=tan−1(0.625)≈32∘
Final Answers:
- (a) Least elevation angle: θ2≈32∘
- (b) Greatest elevation angle: θ1≈62∘
- Initial speed of the ball, u=25m/s
- Horizontal distance to the goalposts, x=50m
- Height of the goalpost, h=3.44m
- Acceleration due to gravity, g=10m/s2
The trajectory of a projectile can be described by the equation:
y=xtan(θ)−gx22u2cos2(θ)
where y is the height of the projectile at horizontal distance x, and θ is the angle of projection.
We want the ball to reach a height h at distance x:
3.44=50tan(θ)−10⋅5022⋅252cos2(θ)
First, calculate 10⋅5022⋅252:
=10⋅25002⋅625=250001250=20
Thus, the equation simplifies to:
3.44=50tan(θ)−20sec2(θ)
Rearranging gives:
20sec2(θ)−50tan(θ)+3.44=0
Using the identity sec2(θ)=1+tan2(θ), we can substitute t=tan(θ):
20(1+t2)−50t+3.44=0
This expands to:
20t2−50t+23.44=0
The quadratic formula is given by:
t=−b±√b2−4ac2a
Here, a=20, b=−50, and c=23.44:
t=50±√(−50)2−4⋅20⋅23.442⋅20
Calculate the discriminant:
=2500−1875.2=624.8
Now, substituting back:
t=50±√624.840
Calculate √624.8≈24.99:
t1=50+24.9940≈1.87475andt2=50−24.9940≈0.62525
Now convert t back to angles:
θ1=tan−1(1.875)≈62∘andθ2=tan−1(0.625)≈32∘
- (a) Least elevation angle: θ2≈32∘
- (b) Greatest elevation angle: θ1≈62∘
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - MOTION IN TWO AND THREE DIMENSIONSEXERCISE - CHECKPOINT3videos
MOTION IN TWO AND THREE DIMENSIONS
- BOOK - RESNICK AND HALLIDAYCHAPTER - MOTION IN TWO AND THREE DIMENSIONSEXERCISE - PROBLEMS50videos
MOTION IN TWO AND THREE DIMENSIONS
- BOOK - RESNICK AND HALLIDAYCHAPTER - MOTION ALONG A STRAIGHT LINEEXERCISE - PRACTICE QUESTIONS ( Integer Type )3videos
MOTION ALONG A STRAIGHT LINE
- BOOK - RESNICK AND HALLIDAYCHAPTER - OSCILLATIONSEXERCISE - Practice Questions57videos
OSCILLATIONS
Similar Questions
A cricketer can throw a ball to a maximum horizontal distance of 150 m. With the same speed how high above the ground can the cricketer throw the ball?
View SolutionA footballer throws a ball from a height of 2.00m above the ground with an initial velocity of 20.0m/s at an angle of 30∘ above the horizontal (a) How long does the ball take to cross the goal line 32.0m from the point of release?(b) What is the ball's height above the ground as it crosses the goal line?
View Solution
Knowledge Check
- Question 1 - Select One
A ball is thrown horizontally with a speed of 20m/s from the top of a tower of height 100m
velocity with which the ball strikes the ground is .A20√6m/s at angle tan−10(√7) below the horizontalB20√6m/s at an angle tan−1(√6) below the horizontalC20√6m/s at an angle tan−1(√5) below the horizontalD10√6m/s at an angle tan−1(√5) below the horizontal - Question 2 - Select One
A cricketer can throw a ball to a maximum horizontal distance of 200 m. With the same speed how much high above the ground can the cricketer throw the same ball?
A50 mB100 mC150 mD200 m - Question 3 - Select One
A football player throws a ball with a velocity of 50 metre/sec at an angle 30 degrees from the horizontal. The ball remains in the air for (g=10m/s2)
A2.5secB1.25secC5secD0.625sec
A football player kicks a ball at ball at an angle of 300 with the horizontal with an initial speed of 20m/s. Assuming that the ball travels in a vertical plane, calculate (a) the time at which the ball reaches the highest point (b) maximum height reached (c ) the horizontal range of the ball (d) the time for which the ball is in air. g=10m/s2.
View SolutionThere is a tall cylindrical building standing in a field. Radius of the cylinder is R=8m. A boy standing at A (at a distance of 10 m from the centre of the cylindrical base of the building) knows that his friend is standing at B behind the building. The line joining A and B passes through the centre of the base of the building. Distance between A and B is 50 m. A wants to throw a ball to B but he realizes that the building is too tall and he cannot throw the ball over it. He throws the ball at a speed of 20m/s such that his friend at B has to move minimum distance to catch it.
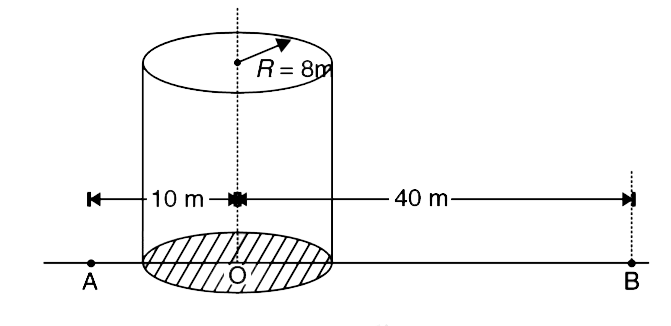
(a) What is the minimum distance that boy at B will have to move to catch the ball?
(b) At what angle to the horizontal does the boy at A throws the ball? Assume that the ball is released and caught at same height above the ground.
[Take g=10m/s2 and sin−1(0.75)≅48.6∘View SolutionA football is kicked at a speed of 20m/s a projection angle of 45∘. A receiver on the goal line 25 metres away in the direction to the kink runs the same instant to meet the ball. Befrom it hits the ground?
View SolutionA cricketer can throw a ball to a maximum horizontal distance of 100 m. How high above the ground can the cricketer throw the ball, with the same speed ?
View SolutionA foot ball is kicked off with an initial speed of 19.6m/s to have maximum range. Goal keeper standing on the goal line 67.4m away in the direction of the kick starts running opposite to the direction of kick to meet the ball at that instant. What must his speed be if he is to catch the ball before it hits the ground?
View Solution

