In Fig., sound waves A and B, both of wavelength λ , are initially in phase and traveling rightward, as indicated by the two rays. Wave A is reflected from four surfaces but ends up traveling in its original direction. Wave B ends in that direction after reflecting from two surfaces. Let distance L in the figure be expressed as a multiple q of λ:L=qλ. What are the (a) smallest and (b) third smallest values of q that put A and B exactly out of phase with each other after the reflections?
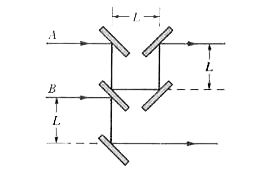
(a) 0.5 , (b) 2.5
Topper's Solved these Questions
- WAVE - IIBOOK - RESNICK AND HALLIDAYCHAPTER - WAVE - IIEXERCISE - PRACTICE QUESTIONS (SINGLE CORRECT CHOICE TYPE)
35 Videos
- WAVE - IIBOOK - RESNICK AND HALLIDAYCHAPTER - WAVE - IIEXERCISE - PRACTICE QUESTIONS (MORE THAN ONE CORRECT CHOICE TYPE)
6 Videos
- WAVE - IIBOOK - RESNICK AND HALLIDAYCHAPTER - WAVE - IIEXERCISE - CHECKPOINTS
4 Videos
- VECTORS BOOK - RESNICK AND HALLIDAYCHAPTER - VECTORS EXERCISE - PRACTICE QUESTIONS
39 Videos
- WAVES-IBOOK - RESNICK AND HALLIDAYCHAPTER - WAVES-IEXERCISE - Practice Questions (Integer Type)
4 Videos
Similar Questions
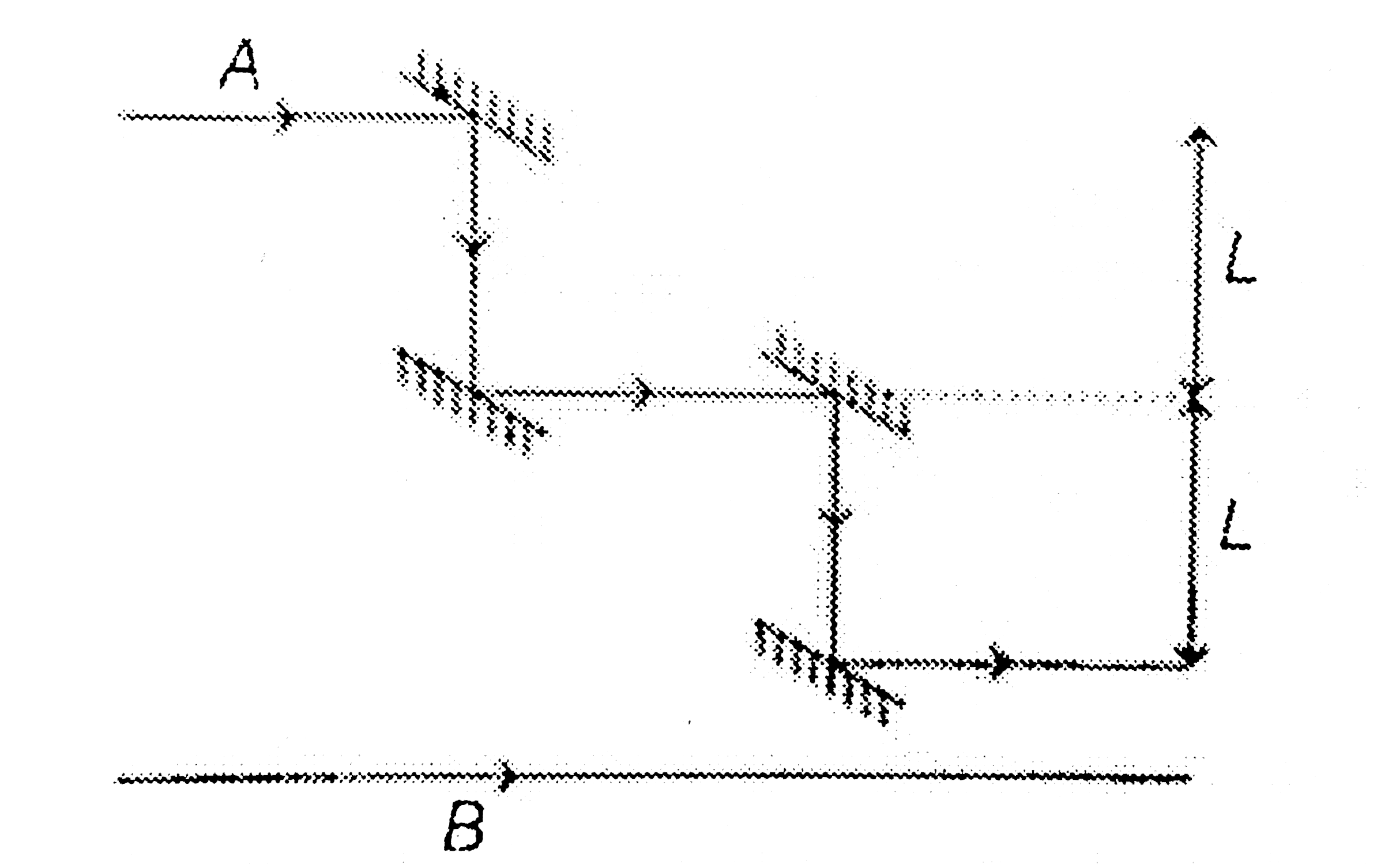
In the figure shown, light waves A and B both of wavelength λ are initially in phase and travelling rightward as incident by the two rays. Wave A is reflected from four parallel surfaces but ends up travelling in original direction. The possible value(s) of distance L in terms of wavelength λ in figure which put A and B exactly out of phase with each other after all the reflected is/are
View SolutionThe change in phase if a wave is reflected from a rigid surface is
View Solution
Knowledge Check
In the figure shown, light waves A and B both of wavelength λ are initially in phase and travelling rightward as incident by the two rays. Wave A is reflected from four parallel surfaces but ends up travelling in original direction. The possible value(s) of distance L in terms of wavelength λ in figure which put A and B exactly out of phase with each other after all the reflected is/are
 Aλ/2B3λ/2C3λ/4Dλ
Aλ/2B3λ/2C3λ/4DλThe change in phase if a wave is reflected from a rigid surface is
AzeroBπ radianC2π radianDπ2 radianThe wave reaching the receiving antenna will have maximum intensity if the direct wave & wave after reflection from earth's surface
Aare out of phase by 90∘Bare out of phase by 270∘Care in phaseDNone of these
Waves of wavelength λ are generated on the water surface. The phase difference between two points at a distance of λ is
View SolutionA sound wave having wavelength λ forms stationary waves after reflection from a surface. The distance between two consecutive nodes is
View SolutionA sound wave having wavelength λ froms stationary waves after reflection from a surface . The distace between two consecutive nodes is
View SolutionWaves of wavelength λ are generated on the water surface. The phase difference between two points at a distance of λ is
View SolutionA sine wave of wavelength λ is travelling in a medium. The minimum distance between the two particles always having same speed is
View Solution
