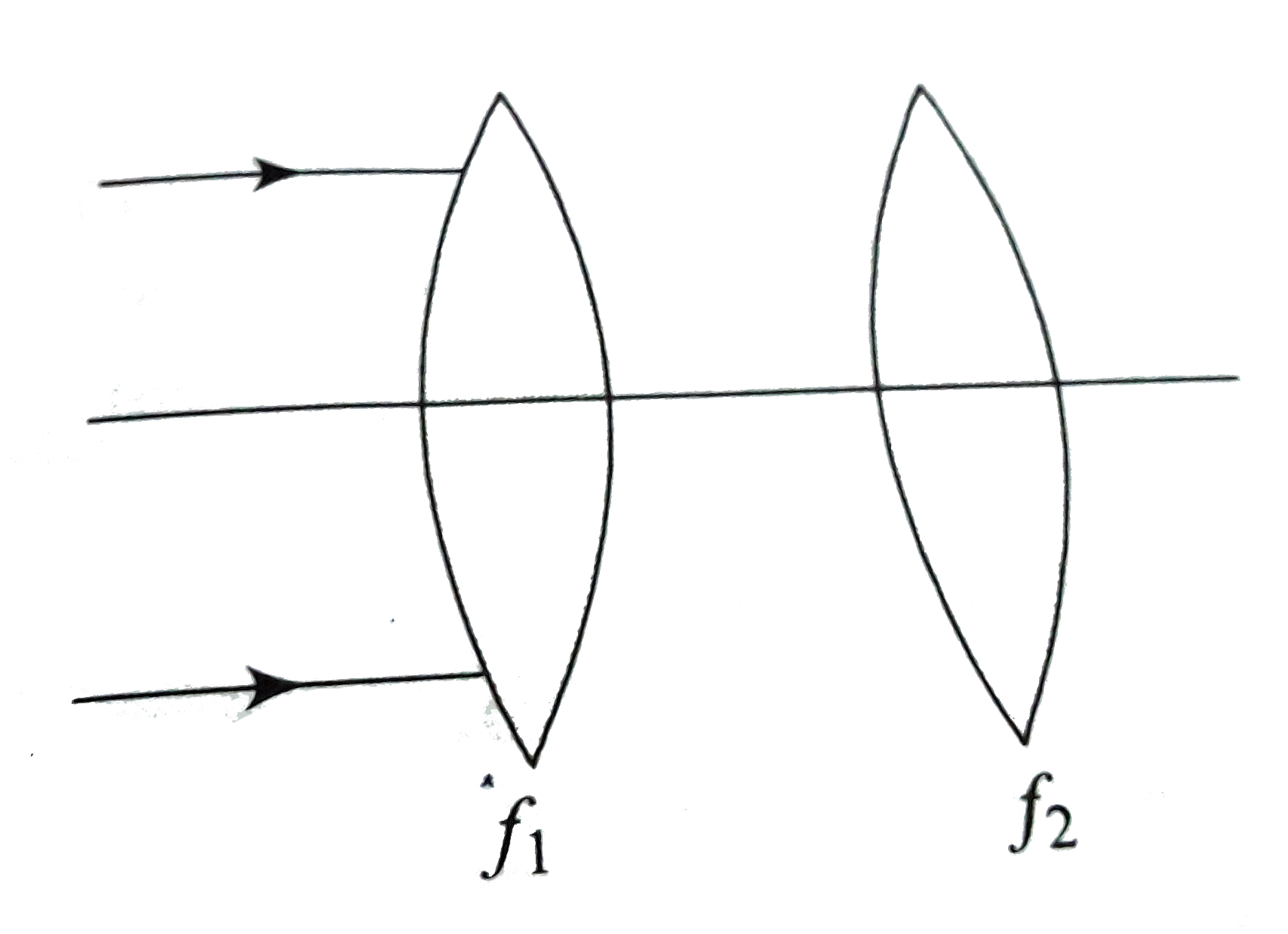
Beam expander
Assume that a parallel beam is incident on two convex lens of focal length 20cm and 40cm. The beam has a diameter of 2cm. What should be the distance between them so that the final beam emerges parallel to the principal axis( Fig. 34-46) ?
Here, again we can see that the image of one will act as an image of another. However , we can also see that for the final beam to be parallel the object for it should be at its first focus. The image of first lens is formed at its second focus. So, we can say that the distance between them should be equal to sum of their focal length.
Calculation : From Fig. 34-46, it is clear that
d=f1+f2=60cm
Learning : If a parallel beam passes through a series of lens, under suitable arrangement, the width of the beam can be expanded. As can be seen from Fig. 34-46, the radius of the beam is changed. This new radius can be found with the help of similar triangles. Here, we can say that
RiRf=fiff

Topper's Solved these Questions
- GEOMETRICAL OPTICS : REFRACTIONBOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFRACTIONEXERCISE - Check point
6 Videos
- GEOMETRICAL OPTICS : REFRACTIONBOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFRACTIONEXERCISE - Problems
44 Videos
- GEOMETRICAL OPTICS : REFLECTION BOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFLECTION EXERCISE - PRACTICE QUESTIONS (Integer Type)
3 Videos
- GRAVITATIONBOOK - RESNICK AND HALLIDAYCHAPTER - GRAVITATIONEXERCISE - PRACTICE QUESTIONS (INTEGER TYPE)
4 Videos
Similar Questions
A parallel beam of light is incident on the system of two convex lenses of focal length f1=20cm and f2=10cm . What should be the distance between the two lenses so that rays after refraction from both the lenses pass undeviated?
View SolutionA convex lens A of focal length 20cm and a concave lens B of focal length 10 cm are kept along the same axis with a distance d between them. If a parallel beam, then d is
View Solution
Knowledge Check
A parallel beam of light is incident on the system of two convex lenses of focal length f1=20cm and f2=10cm . What should be the distance between the two lenses so that rays after refraction from both the lenses pass undeviated?
 A60cmB30cmC90cmD40cm
A60cmB30cmC90cmD40cmA paralel beam of light incident on a concave lens of focal length 10 cm emerges as a parallel beam from a convex lens placed coaxially, the distance between the lenses being 10 cm. The focal length of the convex lens in cm is
A10B15C20D30A parallel beam of monochromatic light falls on a combination of a convex lens and a concave lens of focal lengths 15 cm and 5 cm respectively. What is the distance between the two lenses to obtain a parallel beam of light from the concave lens ?
A20cmB3 cmC10 cmD45 cm
A convex lens A of focal length 20cm and a concave lens B of focal length 10 cm are kept along the same axis with a distance d between them. If a parallel beam, then d is
View SolutionA parallel beam of monochromatic light falls on a combination of a convex lens and a concave lens of focal lengths 15 cm and 5 cm respectively. What is the distance between the two lenses to obtain a parallel beam of light from the concave lens ?
View SolutionA parallel beam of light is incident on a lens of focal length 10 cm. A parallel slab of refractive index 1.5 and thickness 3 cm is placed on the other side of the lens. Find the distance of the final image from the lens.
 .
.
View SolutionA parallel beam of light is incident on a lens of focal length 10 cm. A parallel slab of refractive index 1.5 and thickness 3 cm is placed on the other side of the lens. Find the distance of the final image from the lens.
 .
.
View SolutionA convex lens of focal length 20cm and another plano convex lens of focal length 40cm are placed co-axially . The plano convex lens is silvered on plane surface. What should be the distance (in cm) so that final image of the object 'O' is formed on O itself.
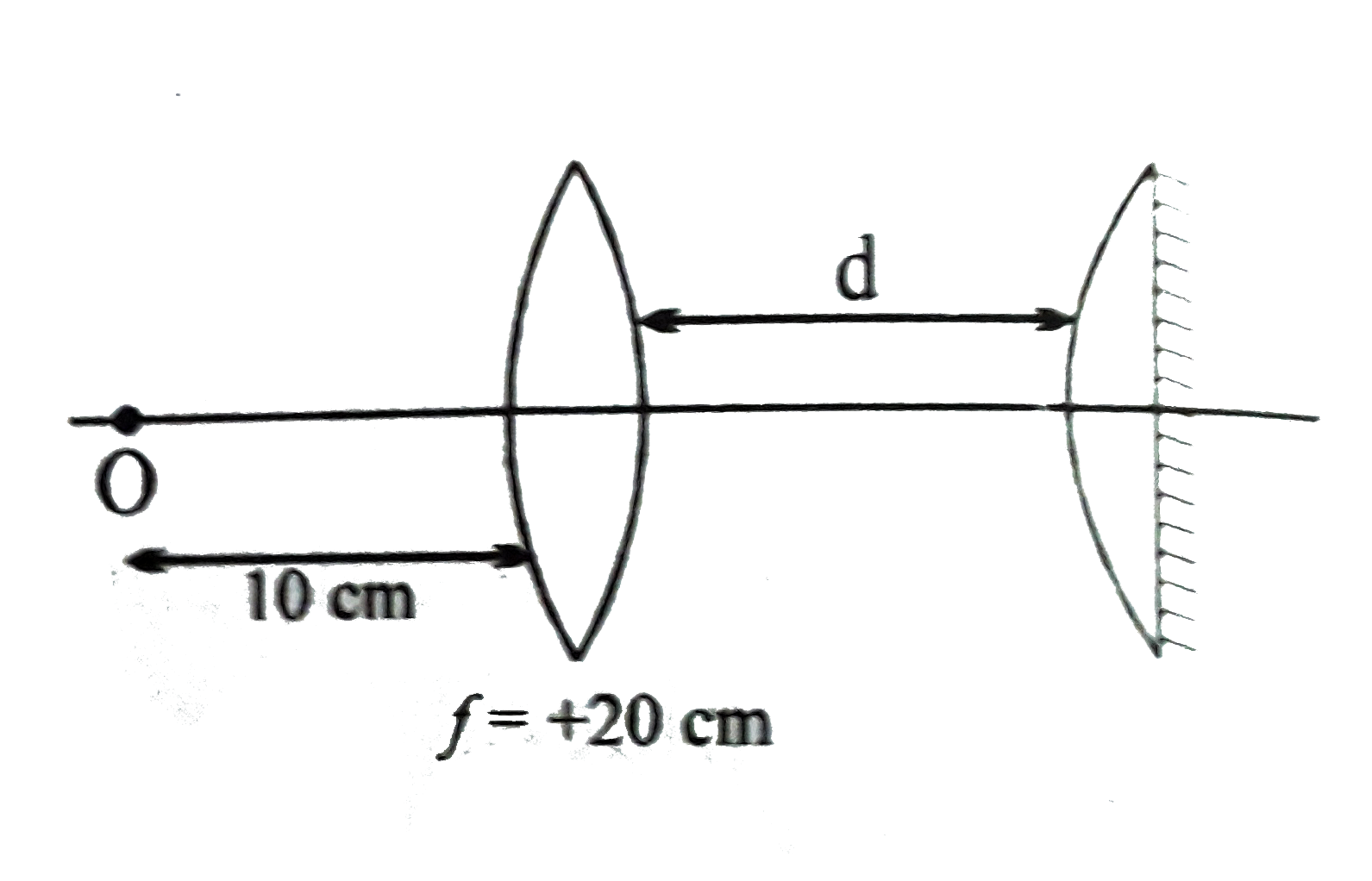
View Solution
