A convex lens focuses an object 40cm from it on a screen placed 10cm away from it. A glass plate (μ=1.5) and of thickness 3cm is inserted between the lens and the screen. Where should the object be placed so that its image is again focused on the screen ?
The correct Answer is:72cm away from the lens
To solve the problem step by step, we will follow the process outlined in the video transcript.
Step 1: Understand the initial setup
We have a convex lens that focuses an object placed 40 cm away from it onto a screen that is 10 cm away from the lens.
Step 2: Identify the given values
- Object distance (u) = -40 cm (the negative sign indicates that the object is on the opposite side of the light direction)
- Image distance (v) = 10 cm (the positive sign indicates that the image is on the opposite side of the lens)
Step 3: Use the lens formula to find the focal length (f)
The lens formula is given by:
1f=1v−1u
Substituting the values:
1f=110−1−40
Calculating:
1f=110+140
To add these fractions, find a common denominator (40):
1f=440+140=540=18
Thus, the focal length f=8cm.
Step 4: Calculate the shift caused by the glass plate
The glass plate has a refractive index μ=1.5 and a thickness of 3 cm. The shift (d) caused by the glass plate can be calculated using the formula:
d=t(1−1μ)
Substituting the values:
d=3(1−11.5)=3(1−23)=3(13)=1cm
Step 5: Adjust the image distance due to the shift
The original image distance was 10 cm. With the shift of 1 cm, the new image distance (v') becomes:
v′=10cm−1cm=9cm
Step 6: Use the lens formula again to find the new object distance (u')
Now we need to find the new object distance (u') such that the image is still focused on the screen. Using the lens formula again:
1f=1v′−1u′
Substituting the values:
18=19−1u′
Rearranging gives:
1u′=19−18
Finding a common denominator (72):
1u′=872−972=−172
Thus, u′=−72cm.
Final Answer
The object should be placed at a distance of 72 cm from the lens (on the same side as the original object).
---
We have a convex lens that focuses an object placed 40 cm away from it onto a screen that is 10 cm away from the lens.
- Object distance (u) = -40 cm (the negative sign indicates that the object is on the opposite side of the light direction)
- Image distance (v) = 10 cm (the positive sign indicates that the image is on the opposite side of the lens)
The lens formula is given by:
1f=1v−1u
Substituting the values:
1f=110−1−40
Calculating:
1f=110+140
To add these fractions, find a common denominator (40):
1f=440+140=540=18
Thus, the focal length f=8cm.
The glass plate has a refractive index μ=1.5 and a thickness of 3 cm. The shift (d) caused by the glass plate can be calculated using the formula:
d=t(1−1μ)
Substituting the values:
d=3(1−11.5)=3(1−23)=3(13)=1cm
The original image distance was 10 cm. With the shift of 1 cm, the new image distance (v') becomes:
v′=10cm−1cm=9cm
Now we need to find the new object distance (u') such that the image is still focused on the screen. Using the lens formula again:
1f=1v′−1u′
Substituting the values:
18=19−1u′
Rearranging gives:
1u′=19−18
Finding a common denominator (72):
1u′=872−972=−172
Thus, u′=−72cm.
The object should be placed at a distance of 72 cm from the lens (on the same side as the original object).
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFRACTIONEXERCISE - Practice questions(single correct choice type)49videos
GEOMETRICAL OPTICS : REFRACTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFRACTIONEXERCISE - Practice questions(More than one correct choice type)14videos
GEOMETRICAL OPTICS : REFRACTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFRACTIONEXERCISE - Check point6videos
GEOMETRICAL OPTICS : REFRACTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFLECTION EXERCISE - PRACTICE QUESTIONS (Integer Type)3videos
GEOMETRICAL OPTICS : REFLECTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - GRAVITATIONEXERCISE - PRACTICE QUESTIONS (INTEGER TYPE)4videos
GRAVITATION
Similar Questions
A convex lens focusses ab object 10 cm from it on a screen paced 10 cm away from it. A goes plate (μ=1.5) of thickenss 1.5 cm is inserted between tehlens and the screen. Where should the object be placed so that its image is again focussed on the screen?
View SolutionA lens is made of three thin different mediums. Radius of curvature and refractive index of each medium is shown iin Figure., Surface AB is straight. An object is placed at some distance from the lens by which a real image is formed on the screen placed at a distance of 10cm from the lens. Find
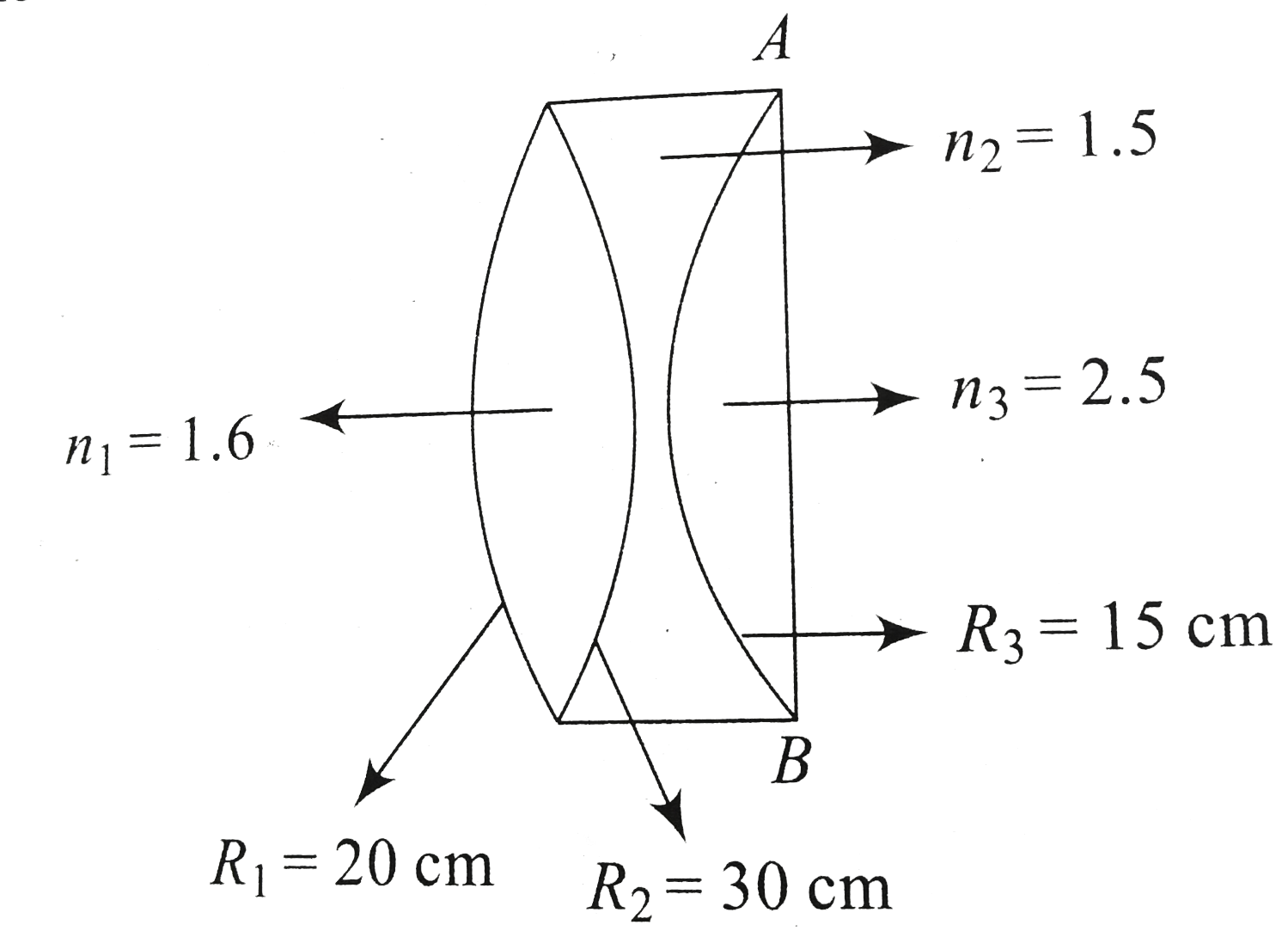
a. The distance of the object from the lens.
b. A slab of thiskness 1.5cm and refractive index 1.5 is introduced between the image and the lens. Find the new position of the object so that image is again formed on the screen.
c. Find the position of the image when same slabe is placed on the other side of the lens {as in part (b)}View Solution
Knowledge Check
- Question 1 - Select One
A convex lens forms an image of an object on a screen 30 cm from the lens. When the lens is moved 90 cm towards the object, then the image is again formed on the screen. Then, the focal length of the lens is
A13 cmB24 cmC33 cmD40 cm - Question 2 - Select One
A thin biconvex lens of focal length f=25cm forms a real image of a real object an a screen. The object is separated by 5cm from the lens. The screen is drawn closer to the lens by a distance 18cm. Through what distance (approximately) must the object be shifted so that its image is again formed on the screen ?
A0.5mm toward leftB0.25mm toward leftC2.5mm toward leftD1.4mm toward left - Question 3 - Select One
A lens forms a virtual image 4cm away from it when an object is placed 10cm away from it. The lens is a ….. Lens of focal length ……………
Aconcave, 6.67cmBconcave, 2.86cmCconvex, 2.86cmDmay be concave or convex, 6.67cm.
A lens with a focal length of f=30cm produces on a screen a sharp image of an object that is at a distance of a=40cm from the lens. A plane parallel plate with thickness of d=9cm is placed between the lens and the object perpendicular to the optical axis of the lens. Through what distance should the screen be shifted for the image of the object to remain distinct? The refractive index of the glass of the plate is μ=1.8.
View SolutionA convex lens of focal length 20 cm is placed at a distance 5 cm from a glass plate (μ=32) of thickness 3 cm. an object is placed at a distance 30 cm from lens on the other side of glass plate. Locate the final image produced by this optical setup.
View SolutionA converging lens of focal length 5 cm is placed at a distance of 20 cm from a screen. How far from the lens should an object be placed so as to form its real image on the screen?
View SolutionA convex lens forms a real image on a screen placed at a distance 60 cm from the object. When the lens is shifted towards the screen by 20 cm , another image of the object is formed on the screen. The focal length of the lens is :
View SolutionA thin converging lens of focal length f = 25 cm forms the image of an object on a screen placed at a distance of 75 cm from the lens. Now the screen in moved closer to the lens by a distance of 25 cm. The distance through which the object has to be shifted so that its image on the screen is sharp again is
View Solution

