A man having height 169 cm is standing near a pole. He casts a shadow 130 cm long. What is the length of the pole if it gives a shadow 420 cm long?
550 cm
589 cm
232 cm
546 cm
The correct Answer is:D
To find the length of the pole based on the given information, we can use the concept of similar triangles. The height of the man and the length of his shadow form one triangle, while the height of the pole and the length of its shadow form another triangle.
1. Identify the given values:
- Height of the man (H_m) = 169 cm
- Length of the man's shadow (S_m) = 130 cm
- Length of the pole's shadow (S_p) = 420 cm
- Height of the pole (H_p) = ?
2. Set up the proportion using similar triangles:
The triangles formed by the man and his shadow and the pole and its shadow are similar. Therefore, we can set up the following proportion:
HmSm=HpSp
3. Substitute the known values into the proportion:
169130=Hp420
4. Cross-multiply to solve for the height of the pole (H_p):
169×420=130×Hp
5. Calculate the left side:
169×420=70980
6. Now, solve for H_p:
Hp=70980130
7. Perform the division:
Hp=546
8. Conclusion:
The height of the pole is 546 cm.
- Height of the man (H_m) = 169 cm
- Length of the man's shadow (S_m) = 130 cm
- Length of the pole's shadow (S_p) = 420 cm
- Height of the pole (H_p) = ?
The triangles formed by the man and his shadow and the pole and its shadow are similar. Therefore, we can set up the following proportion:
HmSm=HpSp
169130=Hp420
169×420=130×Hp
169×420=70980
Hp=70980130
Hp=546
The height of the pole is 546 cm.
Similar Questions
A pole 6 m high casts a shadow 2 3 m long on the ground, then find the angle oi elevation of the sun.
View Solution
Vijay is trying to find the average height of a tower near his house. He is using the properties of similar triangles.The height of Vijay’s house if 20m when Vijay’s house casts a shadow 10m long on the ground. At the same time, the tower casts a shadow 50m long on the ground and the house of Ajay casts 20m shadow on the ground.
What will be the length of the shadow of the tower when Vijay’s house casts a shadow of 12m?View Solution
Knowledge Check
- Question 1 - Select One
A vertical pole 40 m long casts a shadow 20 m long on the ground. At the same time a……….. Tower casts a shadow 50 m long on the ground.
A100mB50mC25 mD150 m - Question 2 - Select One
A flag pole 18 m high casts a shadow 9.6 m long. Find the distance of the top of the pole from the far end of the shadow.
A21.4mB20.4mC30.4mD40.4m - Question 3 - Select One
If a pole 12 m high casts a shadow 4√3m long on the ground then the sun's elevation is
A60∘B45∘C30∘D90∘
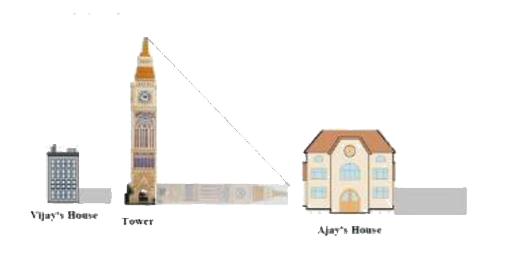
Vijay is trying to find the average height of a tower near his house. He is using the properties of similar triangles.The height of Vijay’s house if 20m when Vijay’s house casts a shadow 10m long on the ground. At the same time, the tower casts a shadow 50m long on the ground and the house of Ajay casts 20m shadow on the ground.
When the tower casts a shadow of 40m, same time what will be the length of the shadow of Ajay’s house?View Solution
Vijay is trying to find the average height of a tower near his house. He is using the properties of similar triangles.The height of Vijay’s house if 20m when Vijay’s house casts a shadow 10m long on the ground. At the same time, the tower casts a shadow 50m long on the ground and the house of Ajay casts 20m shadow on the ground.
When the tower casts a shadow of 40m, same time what will be the length of the shadow of Vijay’s house?View SolutionAt a particular time of a day a 7-m high flagstaff casts a shadow which is 8.2 m long.What is the height of the building which casts a shadow 20.5 metres length at the same moment?
View SolutionAt a particular time of a day a 7-m high flagstaff casts a shadow which is 8.2 m long.What is the height of the building which casts a shadow 20.5 metres length at the same moment?
View Solution
Vijay is trying to find the average height of a tower near his house. He is using the properties of similar triangles.The height of Vijay’s house if 20m when Vijay’s house casts a shadow 10m long on the ground. At the same time, the tower casts a shadow 50m long on the ground and the house of Ajay casts 20m shadow on the ground.
What is the height of Ajay’s house?View Solution

