If the lengths of the sides AB, BC and CA of a triangle ABC are 10 cm, 8 cm and 6 cm respectively and if M is the mid-point of BC and MN || AB to cut AC at N, then the area of the trapezium ABMN is equal to
18 sq. cm
20 sq. cm
12 sq. cm
16 sq.cm
The correct Answer is:A
Step by step video, text & image solution for If the lengths of the sides AB, BC and CA of a triangle ABC are 10 cm, 8 cm and 6 cm respectively and if M is the mid-point of BC and MN || AB to cut AC at N, then the area of the trapezium ABMN is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Similar Questions
Length of two sides AB and BC of a triangle ABC are 28cm and 38 cm respectively . find the length of its third side AC if the perimeter of ABC is 100 cm
View SolutionThe side AB, BC and CA of triangle ABC touch a circle at P,Q and R respectively. If PA =4 cm, BP=3 cm and AC=11 cm, then length of BC is
View Solution
Knowledge Check
- Question 1 - Select One
If the lengths of the sides AB, BC and CA of a triangle ABC are 10 cm, 8 cm and 6 cm respectively and if M is the mid-point of BC and MN || AB to cut AC at N, then the area of the trapezium ABMN is equal to
A18 sq. cmB20 sq. cmC12 sq. cmD16 sq.cm - Question 2 - Select One
The mid points of the sides AB and AC of a triangle aBC are (2,−1) and (−4,7) respectively, then the length of BC is
A10B20C25D30 - Question 3 - Select One
G is the centroid of the triangle ABC, where AB, BC and CA are 7 cm, 24 cm and 25 cm respectively, then BG is:
G त्रिभुज ABC का केन्द्रक है जिसमें AB, BC और CA क्रमशः 7 सेमी, 24 सेमी और 25 सेमी हैं | BG का मान ज्ञात करें |A613cmB813cmC512cmD416cm
Find the length of the altitude AL of an isoceles triangle ABC, where AB = AC = 5 cm and BC = 8 cm.
View SolutionIn ΔABC, AB = 5cm , BC = 8 cm and CA = 7cm. If D and E are respectively the midd-points of AB and BC, determine the length of DE.
View SolutionIn figure , the sides AB, BC and CA of triangle ABC, touch a circle at P,Q and R, respectively . If PA = 4 cm , BP = 3 cm and AC = 11 cm , then length of BC is
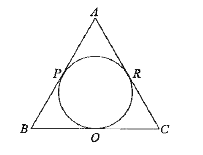View SolutionThe sides AB, BC and AC of a ΔABC are 12 cm, 8 cm and 10 cm respectively. A circle is inscribed in the triangle touching AB, BC and AC at D, E and F, respectively. The difference between the lengths of AD and CE is:
View SolutionArea of ΔABC=30cm2. D and E are the mid - points of BC and AB respectively. Find A(ΔBDE) :
View Solution