Let $f_{1} : R \to R, f_{2} : (- \frac{π}{2}, \frac{π}{2}) \to R, f_{3} : (- 1, e^{π / 2} - 2) \to R and f_{4} : R \to R$ be functions defined by
(i) $f_{1} (x) = \sin (\sqrt{1 - e^{- x^{2}}})$ ,
(ii) $f_{2} (x) = {\begin{matrix} \frac{| \sin x |}{\tan^{- 1} x} if x \neq 0 \\ , where the inverse \\ 1 if x = 0 \end{matrix}$
trigonometric function $\tan^{- 1}$ x assumes values in $(- \frac{π}{2}, \frac{π}{2})$ ,
(iii) $f_{3} (x) = [\sin (\log_{e} (x + 2))]$ , where for $t \in R$ , [t] denotes the greatest integer less than or equal to t,
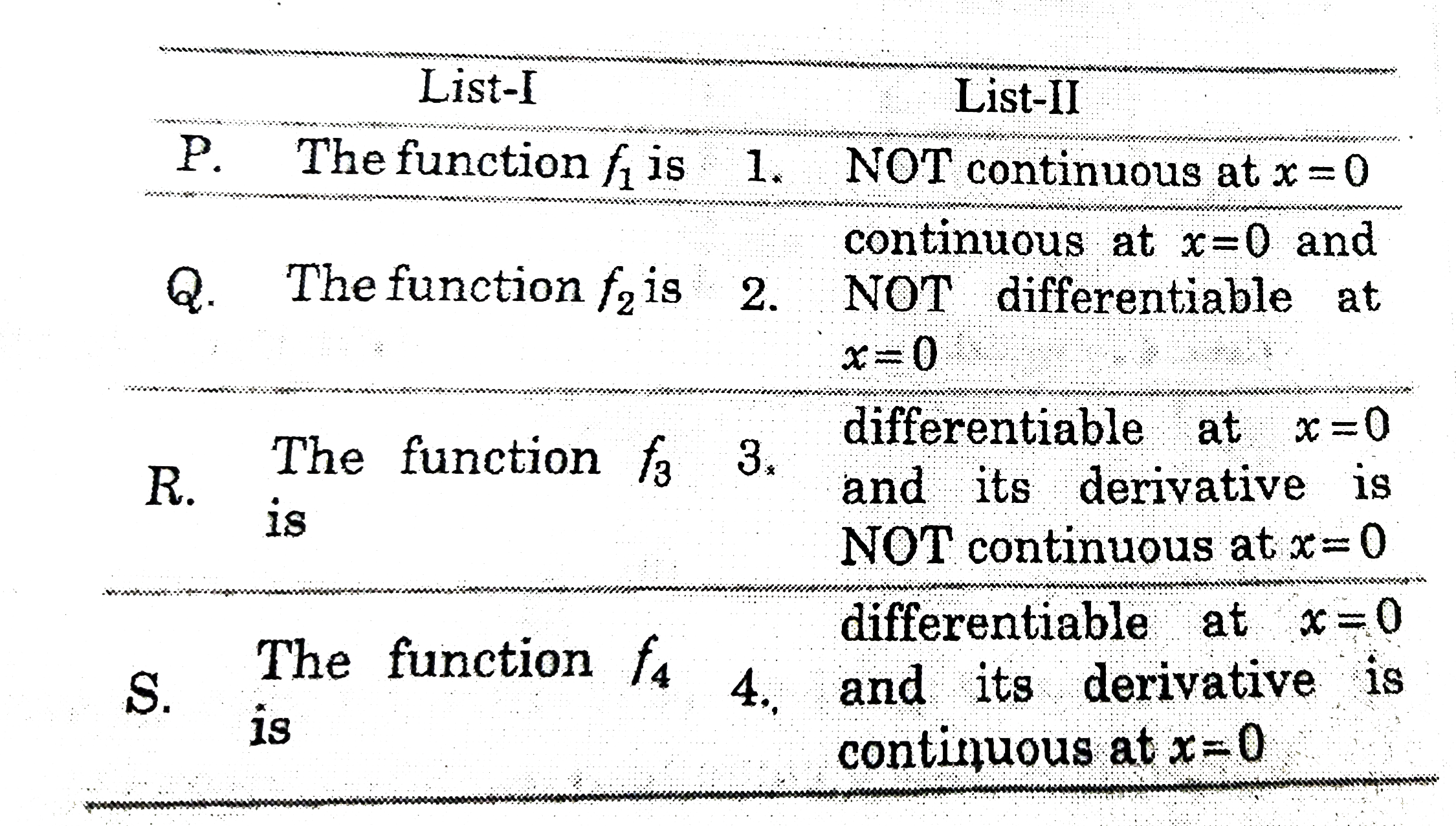
(iv) $f_{4} (x) = {\begin{matrix} x^{2} \sin (\frac{1}{x}) if x \neq 0 \\ 0 if x = 0 \end{matrix}$
$Let f(1) : R to R, f(2) : (-pi/2,pi/2) to R, f(3) : (-1,e^(pi//2)-2) to R and f(4) : R to R be functions defined by (i) f(1) (x) = sin (sqrt(1-e^(-x^(2)))), (ii) f(2)(x) = {{:(|sin x|/(tan^(-1) x)$

A

$P \to 2, Q \to 3, R \to 1, S \to 4$

B

$P \to 4, Q \to 1, R \to 2, S \to 3$

C

$P \to 4, Q \to 2, R \to 1, S \to 3$

D

$P \to 2, Q \to 1, R \to 4, S \to 3$

Text Solution

Verified by Experts

The correct Answer is:D

(i) Given, $f_{1} : R \to R and f_{1} (x) = \sin (\sqrt{1 - e^{- x^{2}}})$
$∴ f_{1} (x)$ is continuous at x = 0
Now, $f_{1}' (x) = \cos \sqrt{1 - e^{- x^{2}}} \cdot \frac{1}{2 \sqrt{1 - e^{- x^{2}}}} (2 x e^{- x^{2}})$
At x = 0
$f_{1}' (x)$ does not exists.
$∴ f_{1} (x)$ is not differential at x = 0
Hence, option (2) for P.
(ii) Given, $f_{2} (x) = {\begin{matrix} \frac{| \sin x |}{\tan^{- 1} x}, if x \neq 0 \\ 1, if x = 0 \end{matrix}$
$\Rightarrow f_{2} (x) = {\begin{matrix} \frac{- \sin x}{\tan^{- 1} x} x < 0 \\ \frac{\sin x}{\tan^{- 1} x} x > 0 \\ 1 x = 0 \end{matrix}$
Clearly, $f_{2} (x)$ is not continuous at x = 0 .
$∴$ Option (1) for Q.
(iii) Given, $f_{3} (x) = [\sin (\log_{e} (x + 2)]$ , where [] is G.I.F. and $f_{3} : (- 1, e^{π / 2} - 2) \to R$
It is given $- 1 < x < e^{π / 2} - 2$
$\Rightarrow - 1 + 2 < x + 2 < e^{π / 2} - 2 + 2$
$\Rightarrow 1 < x + 2 < e^{π / 2}$
$\Rightarrow \log_{e} 1 < \log_{e} (x + 2) < \log_{e} e^{π / 2}$
$\Rightarrow 0 < \log_{e} (x + 2) < \frac{π}{2}$
$\Rightarrow \sin 0 < {\sin \log}_{e} (x + 2) < \sin . \frac{π}{2}$
$\Rightarrow 0 < {\sin \log}_{e} (x + 2) < 1$
$∴ [{\sin \log}_{e} (x + 2)] = 0$
$∴ f_{3} (x) = 0, f'_{3} (x) = f_{3}'' (x) = 0$
It is differentiable and continuous at x = 0 .
$∴$ Option (4) for R
(iv) Given, $f_{4} (x) = {\begin{matrix} x^{2} \sin (\frac{1}{x}), if x \neq 0 \\ 0, if x = 0 \end{matrix}$
Now, $lim_{x \to 0} f_{4} (x) = lim_{x \to 0} x^{2} \sin (\frac{1}{x}) = 0$
$f_{4}' (x) = 2 x \sin (\frac{1}{x}) - \cos (\frac{1}{x})$
For $x = 0, f_{94})' (x) = lim_{h \to 0} \frac{f (0 + h) - f (0)}{h}$
$\Rightarrow f_{4}' (x) = lim_{x \to 0} \frac{h^{2} \sin (\frac{1}{h}) - 0}{h}$
$\Rightarrow f_{4}' (x) = lim_{h \to 0} h \sin (\frac{1}{h}) = 0$
Thus, $f_{4}' (x) = {\begin{matrix} 2 x \sin (\frac{1}{x}) - \cos (\frac{1}{x}), x \neq 0 \\ 0, x = 0 \end{matrix}$
Again, $lim_{x \to 0} (2 x \sin (\frac{1}{x}) - \cos (\frac{1}{x}))$
does not exists.
Since, $lim_{x \to 0} \cos (\frac{1}{x})$ does not exists.
Hence, f'(x) is not continuous at x = 0.
$∴$ Option (3) for S.

|

Updated on:21/07/2023

Class 12 MATHS LIMITS AND DERIVATIVES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One or More
A function $f : R \to R$ is given by $f (x) = {\begin{matrix} x^{4} (2 + sin \frac{1}{x}) & x \neq 0 0 & x = 0 \end{matrix},$ then
Af has a continous derivative $\forall x \in R$
Bf is a bounded function
Cf has an global minimum at $x = 0$
Df" is continous $\forall x \in R$
Question 2 - Select One or More
A function $f : R \to R$ is given by $f (x) = {\begin{matrix} x^{4} (2 + sin \frac{1}{x}) & x \neq 0 0 & x = 0 \end{matrix},$ then
Af has a continous derivative $\forall x \in R$
Bf is a bounded function
Cf has an global minimum at $x = 0$
Df" is continous $\forall x \in R$

IIT JEE PREVIOUS YEAR-LIMIT,CONTINUITY AND DIFFERENTIABILITY -(Match the Columns)

Let f(1) : R to R, f(2) : (-pi/2,pi/2) to R, f(3) : (-1,e^(pi//2)-2) ...
Text Solution

Home
Ask Doubt
Profile