The orthocenter of the triangle formed by lines x+y=1,2x+3y=6and4x−y+4=0 lines in quadrant number
Video Solution
Text Solution
Let H(h,k) be orthocentre
⇒(slope ofAH)⋅(Slope of BC)=−1
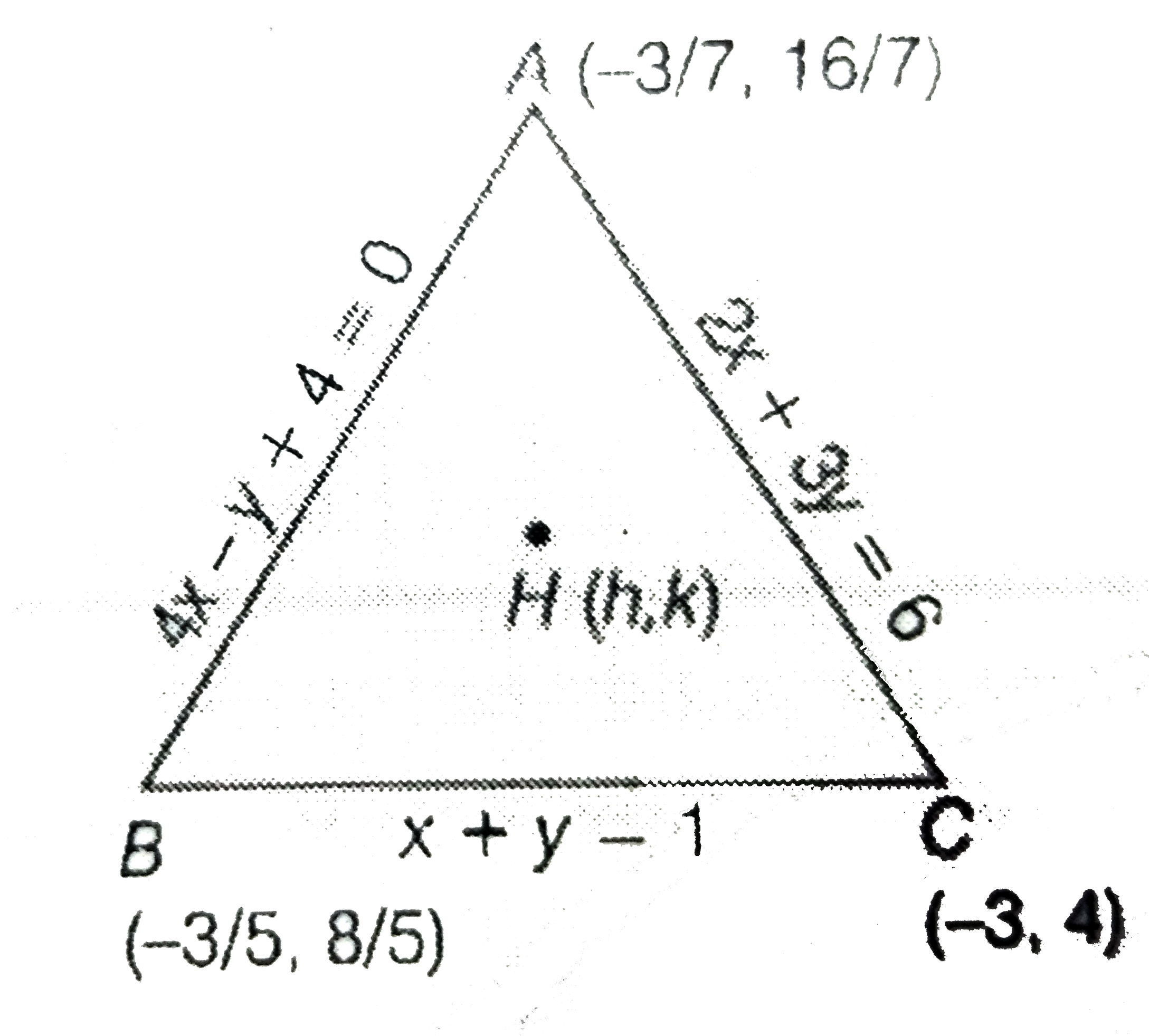
⇒⎛⎝k−167h+37⎞⎠⋅(−1)=−1
⇒k−167=h+37
⇒h−k=−197……(i)
Also, (slope ofCH)⋅(slope ofAB)=−1
⇒k−4h+3⋅(4)=−1
⇒4h−16=−h−3
⇒h+4k=13.......(ii)
On solving Eqs. (i) and (ii), we get h=37, k=227
∴Orthocentre(37,227)
Hence, this coordinate lies in the first quadrant.
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - STRAIGHT LINE AND PAIR OF STRAIGHT LINESEXERCISE - VARIOUS FORMS OF STRAIGHT LINE (True/False)3videos
STRAIGHT LINE AND PAIR OF STRAIGHT LINES
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - STRAIGHT LINE AND PAIR OF STRAIGHT LINESEXERCISE - VARIOUS FORMS OF STRAIGHT LINE (Analytical & Desriptive Questions)19videos
STRAIGHT LINE AND PAIR OF STRAIGHT LINES
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - STRAIGHT LINE AND PAIR OF STRAIGHT LINESEXERCISE - VARIOUS FORMS OF STRAIGHT LINE (Objective Questions II) (Only or more than one correct option)3videos
STRAIGHT LINE AND PAIR OF STRAIGHT LINES
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - SOLVED PAPER 2019EXERCISE - Paper -2 section-34videos
SOLVED PAPER 2019
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - THEORY OF EQUATIONSEXERCISE - SOME SPECIAL FORMS18videos
THEORY OF EQUATIONS
Similar Questions
Knowledge Check
- Question 1 - Select One
The orthocenter of the triangle formed by the lines xy=0andx+y=1 is
A(1/2,1/2)B(1/3,1/3)C(0,0)D(1/4,1/4) - Question 2 - Select One
Area of triangle formed by straight lines x+y=4,2x−y=2andx−2=0 is
A4 sq. unitB9 sq. unitC72 sq. unitDNone of these - Question 3 - Select One
The incentre of triangle formed by lines x=0,y=0 and 3x+4y=12 is
A(3,1)B(1,2)C(2,1)D(1,1)
The orthocentre of the triangle formed by the lines x−7y+6=0,2x−5y−6=0and7x+y−8=0 is
View SolutionThe circumcentre of the triangle formed by the lines, xy+2x+2y+4=0andx+y+2=0 is-
View SolutionThe orthocenter of the triangle formed by the lines x+3y-10=0 and 6x2+xy−y2=0 is
View SolutionThe orthocenter of the triangle formed by the lines x+y=1 and 2y2−xy−6x2=0 is
View SolutionThe incentre of triangle formed by lines x=0,y=0 and 3x+4y=12 is
View Solution

