Doubtnut Promotions Sticky

A ray of light along x+√3y=√3 gets reflected upon reaching x-axis, the equation of the reflected ray is

A

y=x+√3

B

√3y=x−√3

C

y=√3x−√3

D

√3y=x−1

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

Take any point B(0,1) on given line.
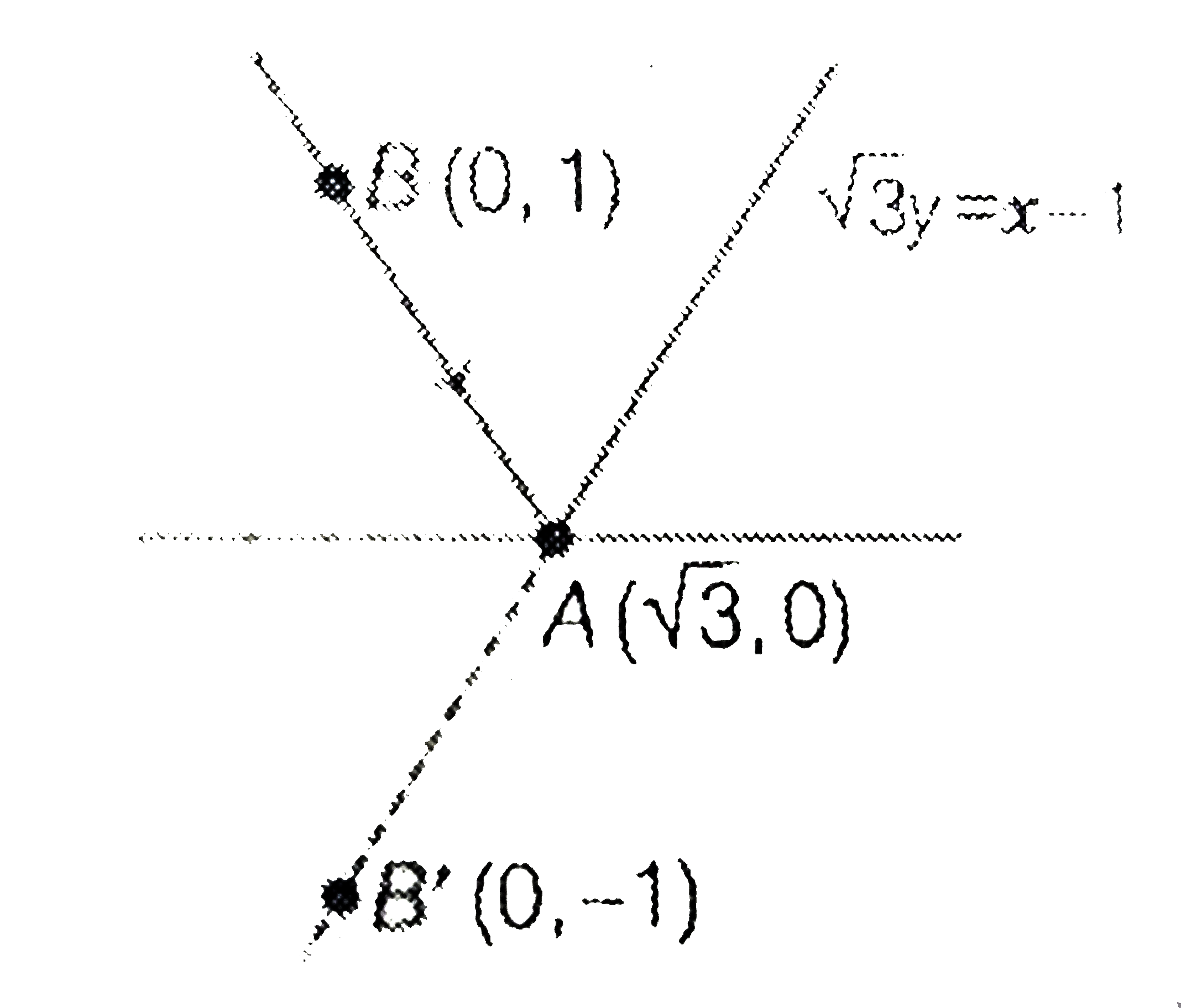
ocr_image
Equation of AB' is
y−0=−1−00−√3(x−√3)
⇒−√3y=−x+√3
⇒x−√3y=√3
⇒√3y=x−√3

|
Updated on:21/07/2023
Class 12MATHSSTRAIGHT LINE AND PAIR OF STRAIGHT LINES

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One

    Statement I A ray of light incident at the point (-3,-1) gets reflected from the tangent at (0,-1) to the circle x2+y2=1. If the reflected ray touches the circle, then equation of the reflected ray is 4y-3x=5
    Statement II The angle of incidence = angle of reflection i.e. ∠i=∠r,

    AStatement I is true, Statement II is true, Statement II is a correct explanation for Statement I
    BStatement I is true, Statement II is true, Statement II is not a correct explanation for Statement I
    CStatement I is true, Statement II is false
    DStatement I is false, Statement II is true
  • Question 2 - Select One

    A ray of light is sent through the point P(1,2,3) and is reflected on the XY plane. If the reflected ray passes through the point Q(3,2,5) then the equation of the reflected ray is

    Ax−31=y−20=z−51
    Bx−31=y−20=z−5−4
    Cx−31=y−20=z−54
    Dx−11=y−20=z−34