Thre block A, B and C are lying on a smooth horizontal surface, as shwon in the figure. A and B have equal masses m while C has mass M. Block A is given an initial seed v towards B due to which it collides with B perfectly inelastically. The combined mass collides with C, also perfectly inelastically and 56th of the initial kinetic energy is lost whole process. If the value of M/m is 4x. FInd the value of x.

Kinetic energy of block A
k1=12mv20
∴ From principle of linear momentum conservation
mv0=(2m+M)vfΦvf=mv02m+M
According to question of 56 th the initial kinetic energy is lost in whole process.
∴kjkf=6⇒12mv2012(2m+M)(mv02m+M)2=6
Φ2m+Mm=6∴Mm=4
Topper's Solved these Questions
- CHAPTERWISE NUMERIC /INTEGER ANSWER QUESTIONSBOOK - DISHA PUBLICATIONCHAPTER - CHAPTERWISE NUMERIC /INTEGER ANSWER QUESTIONSEXERCISE - CHAPTER 6 (SYSTEM OF PARTICLES AND ROTATIONAL MOTION)
14 Videos
- CHAPTERWISE NUMERIC /INTEGER ANSWER QUESTIONSBOOK - DISHA PUBLICATIONCHAPTER - CHAPTERWISE NUMERIC /INTEGER ANSWER QUESTIONSEXERCISE - CHAPTER -7 (GRAVITATION)
15 Videos
- CHAPTERWISE NUMERIC /INTEGER ANSWER QUESTIONSBOOK - DISHA PUBLICATIONCHAPTER - CHAPTERWISE NUMERIC /INTEGER ANSWER QUESTIONSEXERCISE - CHAPTER -4 (LAWS OF MOTION)
15 Videos
- ATOMSBOOK - DISHA PUBLICATIONCHAPTER - ATOMSEXERCISE - EXERCISE-2: CONCEPT APPLICATOR
30 Videos
- COMMUNICATION SYSTEM BOOK - DISHA PUBLICATIONCHAPTER - COMMUNICATION SYSTEM EXERCISE - EXERCISE-2 : Concept Applicator
30 Videos
Similar Questions
Three blocks A, B and C are lying on a smooth horizontal surface, as shown in the figure. A and B have equal masses, m while C has mass M. Block A is given an inita speed v towards B due to which it collides with B perfectly inelastically. The combined mass collides with C, also perfectly inelastically 56th of the initial kinetic energy is lost in whole process. What is balue of M/m?
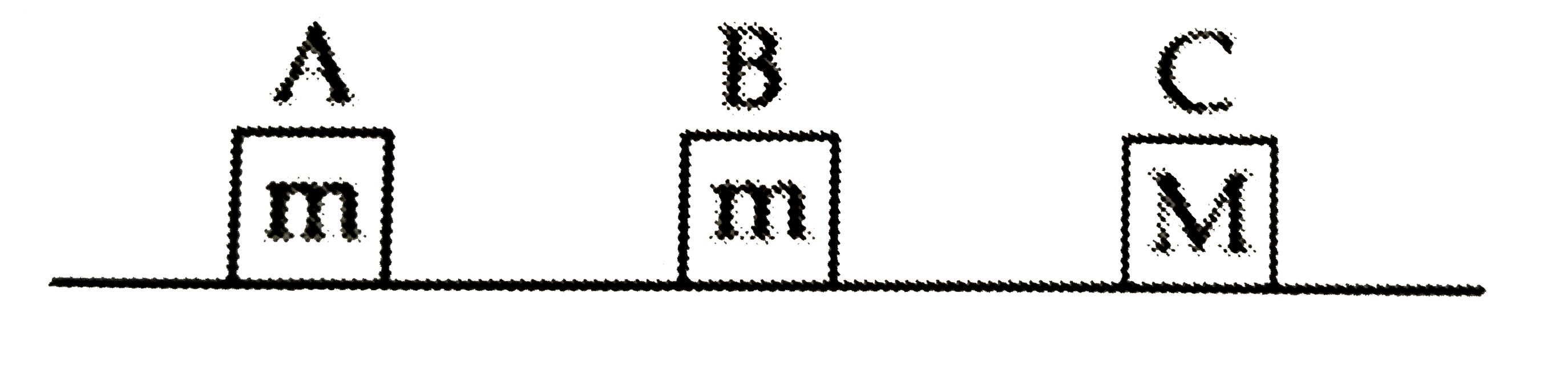
View SolutionThree blocks A, B and C are lying on a smooth horizontal surface, as shown in the figure. A and B have equal masses, m while C has mass M. Block A is given an inita speed v towards B due to which it collides with B perfectly inelastically. The combined mass collides with C, also perfectly inelastically 56th of the initial kinetic energy is lost in whole process. What is balue of M//M?
View Solution
Knowledge Check
Two identical mass M moving with velocity u1andu2 collide perfectly inelastically. The loss in kinetic energy is
AM2(u2−u1)2BM2(u21−u22)CM4(u2−u1)2DM4(u21−u22)Three blocks A, B and C each of mass m are placed on a surface as shown in the figure. Blocks B and C are initialyy at rest. Block A is moving to the right with speed v. It collides with block B and stricks to it. The A-B combination collides elastically with block C. Which of the following statement is (are) true about the velocity, of block B and C.
 AVelocity of the block C after collision is 2/3v towards rightBVelocity of the A−B combination after collision is v3 towards leftCVelocity of the A−B combination after collision is 23v towards leftDVelocity of the block C after collision is v3 towards right.
AVelocity of the block C after collision is 2/3v towards rightBVelocity of the A−B combination after collision is v3 towards leftCVelocity of the A−B combination after collision is 23v towards leftDVelocity of the block C after collision is v3 towards right.Three blocks A,B and C are suspended as shown in the figure. Mass of each block A and C is m. if the system is in equilibrium and mass of B is M, then
 AM=2mBM<2mCM>2mDM=m.
AM=2mBM<2mCM>2mDM=m.
Is whole of the kinetic energy lost in a perfectly inelastic collision ?
View SolutionThree blocks A, B and C each of mass m are placed on a surface as shown in the figure. Blocks B and C are initialyy at rest. Block A is moving to the right with speed v. It collides with block B and stricks to it. The A-B combination collides elastically with block C. Which of the following statement is (are) true about the velocity, of block B and C.

View SolutionThree blocks A,B and C are suspended as shown in the figure. Mass of each block A and C is m. if the system is in equilibrium and mass of B is M, then

View SolutionA ball of mass 5 kg moving with speed 8 m/s collides head on with another stationary ball of mass 15 kg. If collision is perfectly inelastic, then loss in kinetic energ is
View SolutionA ball of mass 5 kg moving with speed 8 m/s collides head on with another stationary ball of mass 15 kg. If collision is perfectly inelastic, then loss in kinetic energ is
View Solution
