Show that the coefficient of area expansion , (ΔA/A)/ΔT , of a rectangular sheet of the solid is twice its linear expansivity , α1.Solution in Telugu
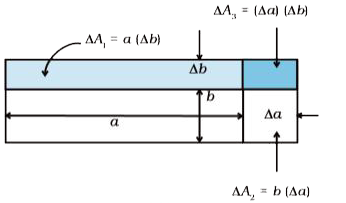
Consider a rectangular sheet of the solid material of length a and breadth b (Fig. 11.8). When the temperature increases by ΔT,a Increases by Δa=α1aΔT and b increases by Δb=α1,bDetlaT. From Fig. 11.8, the increase in area
ΔA=ΔA1+ΔA2+ΔA3
ΔA=aΔb+bΔa+(Δa)(Δb)
=aα1bΔT+bα1aΔT+(α1)2ab(ΔT)2
=α1abΔT(2+α1ΔT)=α1AΔT(2+α1ΔT)
Since α1≈10−5K−1, from Table 11.1 , the product α1ΔT for fractional temperature is small in comparision with 2 and may be neglected.
Hence,
(ΔAA)1ΔT≈2αl
Topper's Solved these Questions
- BOOK - NCERT TELUGUCHAPTER - THERMAL PROPERTIME OF MATTEREXERCISE - EXERCISES30videos
THERMAL PROPERTIME OF MATTER
- BOOK - NCERT TELUGUCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - EXERCISES (TRUE OR FALSE)5videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - NCERT TELUGUCHAPTER - THERMODYNAMICS EXERCISE - EXERCISES10videos
THERMODYNAMICS
Similar Questions
The triple points of neon and carbon dioxide are 24.57K and 216.55K respectively. Express these temperatures on the Celsius and Fahrenheit scales.
06:19View SolutionDuring rolling, the force of friction acts in the same direction as the direction of motion of the CM of the body.
03:21View SolutionA geyser heats water flowing at the rate of 3.0 litres per minute from 27°C to 77°C. If the geyser operates on a gas burner, what is the rate of consumption of the fuel if its heat of combustion is 4.0×104J/g ?
06:07View SolutionThe coefficient of linear expansion of crystal in one direction is alpha_(1) and that in every direction perpendicular to it is alpha_(2) . The coefficient of cubical expansion is
02:48View SolutionShow that the coefficeint of area expansions (Delta A//A)//Delta T of a rantangular sheet of the solid is twice its linear expansitivity alpha .
04:12View Solutionयह दर्शाइए कि किसी ठोस की आयताकार शीट का क्षेत्र प्रसार गुणांक, ΔA/AΔT, इसके रैखिक प्रसार गुणांक αl का दो गुना होता है |
03:46View Solution" The relation between coefficient of linear expansion "(alpha_(1))" and coefficient of volume expansion ( "alpha_(5)" ) is "
03:54View SolutionA solid rectangular sheet has two different coefficients of linear expansion alpha_(1) " and " alpha_(2) along its length and breadth respectively. The coefficient of surface expansion is ("for " alpha_(1)t "<<"1, alpha_(2)t"<<"1)
07:48View SolutionShow that the coefficient of superficial expansion of a rectangular sheet of the solid is twice its coefficient of linear expansion.
01:43View SolutionShow that the coefficient of area expansion , (ΔA/A)/ΔT , of a rectangular sheet of the solid is twice its linear expansivity , α1.
03:00View SolutionShow that the coefficient of area expansion , (Delta A//A)//Delta T , of a rectangular sheet of the solid is twice its linear expansivity , alpha_(1) .
02:49View SolutionA solid rectangular sheet has two different coefficients of linear expansion alpha_1 and alpha_2 along its length and breadth respectively. The coefficient of surface expansion is (for alpha_1 t ltlt 1, alpha_2 lt lt 1 )
01:27View SolutionShow that the coefficient of area expansions (ΔA/A)/ΔT of a rectangular sheet of the solid is twice its linear expansivity α.
05:02View SolutionShow that the coefficient of area expansions, (DeltaA//A)//DeltaT , of a rectangular sheet of the solid is twice its linear expansively, alpha_(l).(alpha_(l)=10^(-5)K^(-1))
05:50View SolutionThe coefficient of linear expansion of crystal in one direction is α1 and that in every direction perpendicular to it α2. The coefficient of cubical expansion is :
04:42View Solution

