Explain square root function with graph
Video Solution
Text Solution
Let f be a real-valued funcation which associates to each non-negative real number x, its non-negative square root.
Then, f:R+0→R+0:f(x)=√x is called the suare-root funcation.
Domain f(x)=R+0and range f(x)=R+0
Some of the graph are (0,0)], (1,1) ,(2,1.4), (3,1.7), ( 4,2) , ( 5, 2.2), etc. joining these point, we get the required graph.
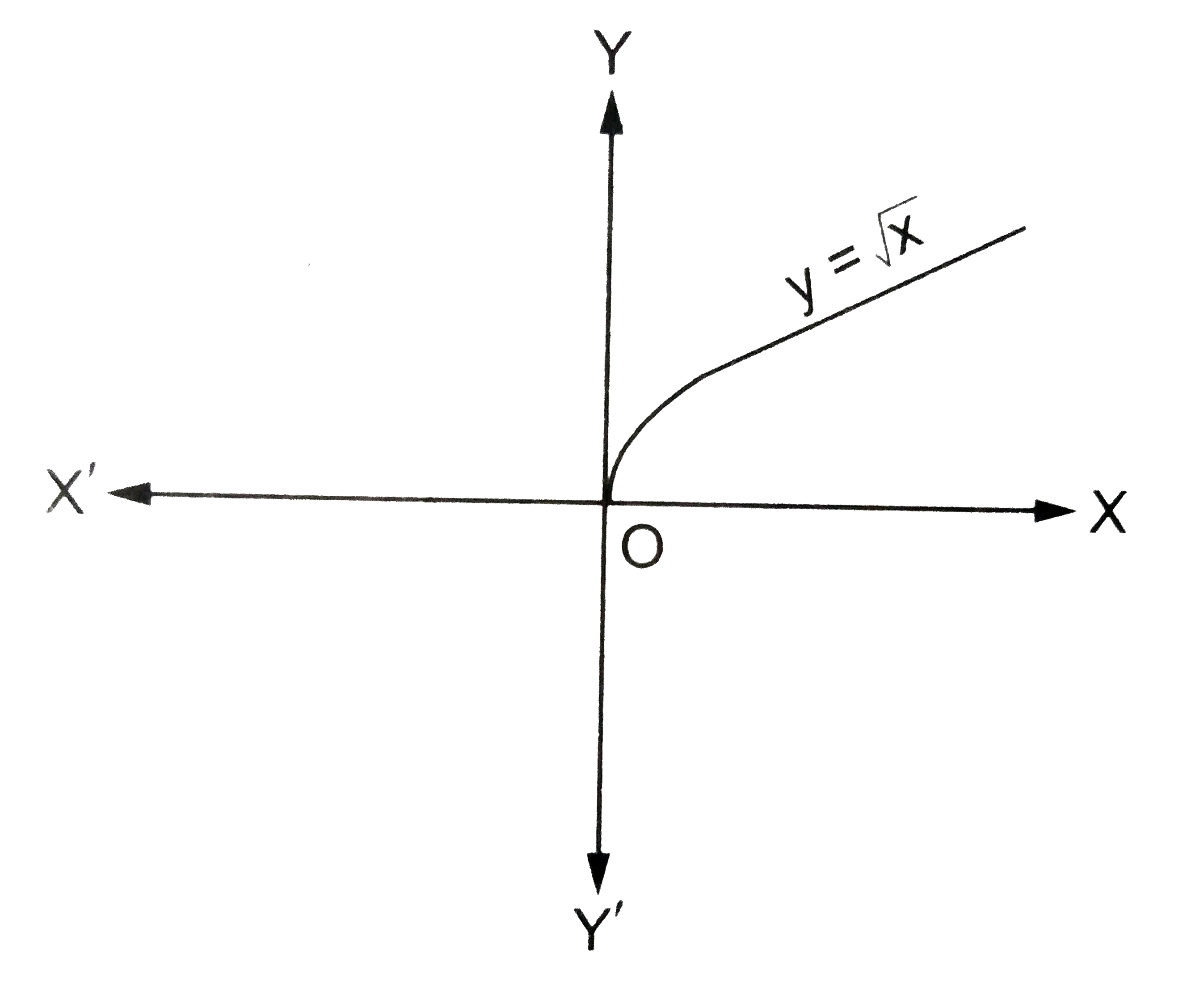
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - RS AGGARWALCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Exercise 9A27videos
CONTINUITY AND DIFFERENTIABILITY
- BOOK - RS AGGARWALCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Exercise 9B11videos
CONTINUITY AND DIFFERENTIABILITY
- BOOK - RS AGGARWALCHAPTER - BINARY OPERATIONSEXERCISE - Exercise 3B8videos
BINARY OPERATIONS
- BOOK - RS AGGARWALCHAPTER - CROSS,OR VECTOR, PRODUCT OF VECTORSEXERCISE - Exercise 2426videos
CROSS,OR VECTOR, PRODUCT OF VECTORS
Similar Questions
Explain cube root function with graph
View SolutionExplain quadratic expression with graph
View SolutionExplain Reciprocal functions with graph
View SolutionExplain Constant function with graph
View SolutionExplain Exponential function with graph (a^x)
View SolutionExplain Identity function with graph
View SolutionExplain signum function with graph
View SolutionFunction and Graph
View SolutionExplain cubic expression with graph
View SolutionExplain Modulus Function with graph
View Solution

