Show that the cone of greatest volume which can be inscribed in a given sphere is such that three times its altitude is twice the diameter of the sphere. Find the volume of the largest come inscribed ina sphere of radius R.

Video Solution

Text Solution

Verified by Experts

Let R be the radius of the given sphere with centre O, and let V be the volume of the inscribed cone, h be its height and r be the radius of the base.
In the given figure, we have $O D = A D - A O = (h - R)$
$∴ R^{2} = {(h - R)}^{2} + r^{2} or r^{2} = h (2 R - h)$
Now, $V = \frac{1}{3} π r^{2} h = \frac{1}{3} π h^{2} (2 R - h)$ [using (i)]
$∴ \frac{d V}{d h} = \frac{1}{3} π h (4 R - 3 h), and \frac{d^{2} V}{d h^{2}} = (\frac{4}{3} π R - 2 π h)$
For a maxima or minima, we have $\frac{d V}{d h} = 0$
Now, $\frac{d V}{d h} = 0 \Rightarrow \frac{1}{3} π h (4 R - 3 h) 0$
$\Rightarrow h = 0 or (4 R - 3 h) = 0 \Rightarrow h = \frac{4}{3} R [∵ h \neq 0]$
And, ${[\frac{d^{2} V}{d h^{2}}]}_{h = (4 / 3) R} = - \frac{4 π R}{3} < 0$
So, V is maximum when $h = \frac{4}{3} R$ , i.e., when $3 h = 2 (2 R)$
i.e., 3 times the height = 2 times the diameter
Volume of the largest cone $= \frac{1}{3} π \times \frac{16 R^{2}}{9} \times (2 R - \frac{4 R}{3}) = \frac{32 π R^{3}}{81}$

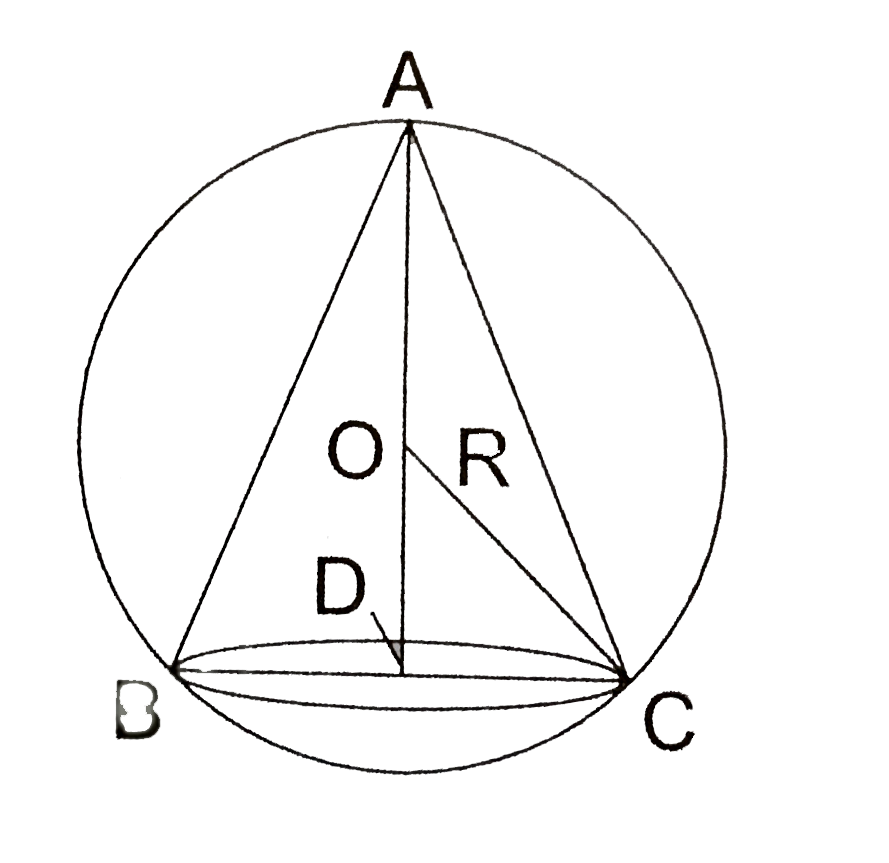
Updated on:21/07/2023