i53+i72+i93+i102=2i.
To solve the equation i53+i72+i93+i102=2i, we will first simplify each term using the properties of the imaginary unit i.
1. Understanding the Powers of i:
The powers of i cycle every 4:
- i1=i
- i2=−1
- i3=−i
- i4=1
- Then it repeats: i5=i, i6=−1, etc.
2. Finding i53:
To find i53, we calculate 53mod4:
53÷4=13(remainder 1)
Thus, i53=i1=i.
3. Finding i72:
Now, for i72:
72÷4=18(remainder 0)
Thus, i72=i0=1.
4. Finding i93:
Next, for i93:
93÷4=23(remainder 1)
Thus, i93=i1=i.
5. Finding i102:
Finally, for i102:
102÷4=25(remainder 2)
Thus, i102=i2=−1.
6. Combining the Results:
Now, we can combine all the results:
i53+i72+i93+i102=i+1+i−1
Simplifying this gives:
i+i+1−1=2i
7. Conclusion:
Therefore, we have shown that:
i53+i72+i93+i102=2i
This confirms the original statement.
The powers of i cycle every 4:
- i1=i
- i2=−1
- i3=−i
- i4=1
- Then it repeats: i5=i, i6=−1, etc.
To find i53, we calculate 53mod4:
53÷4=13(remainder 1)
Thus, i53=i1=i.
Now, for i72:
72÷4=18(remainder 0)
Thus, i72=i0=1.
Next, for i93:
93÷4=23(remainder 1)
Thus, i93=i1=i.
Finally, for i102:
102÷4=25(remainder 2)
Thus, i102=i2=−1.
Now, we can combine all the results:
i53+i72+i93+i102=i+1+i−1
Simplifying this gives:
i+i+1−1=2i
Therefore, we have shown that:
i53+i72+i93+i102=2i
This confirms the original statement.
Topper's Solved these Questions
- BOOK - RS AGGARWALCHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - EXERCISE 5 B28videos
COMPLEX NUMBERS AND QUADRATIC EQUATIONS
- BOOK - RS AGGARWALCHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - EXERCISE 5 C12videos
COMPLEX NUMBERS AND QUADRATIC EQUATIONS
- BOOK - RS AGGARWALCHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - EXERCISE 5 G28videos
COMPLEX NUMBERS AND QUADRATIC EQUATIONS
- BOOK - RS AGGARWALCHAPTER - COMBINATIONSEXERCISE - Exercise 9D18videos
COMBINATIONS
- BOOK - RS AGGARWALCHAPTER - CONDITIONAL IDENTITIES INVOLVING THE ANGLES OF A TRIANGLE EXERCISE - EXERCISE 1612videos
CONDITIONAL IDENTITIES INVOLVING THE ANGLES OF A TRIANGLE
Similar Questions
Knowledge Check
- Question 1 - Select One
In the box shown current i enters at H and leaves at C. If iAB=i6,iDC=2i3,iHA=i2,iGF=i6,iHE=i6, choose the branch in which current is zero
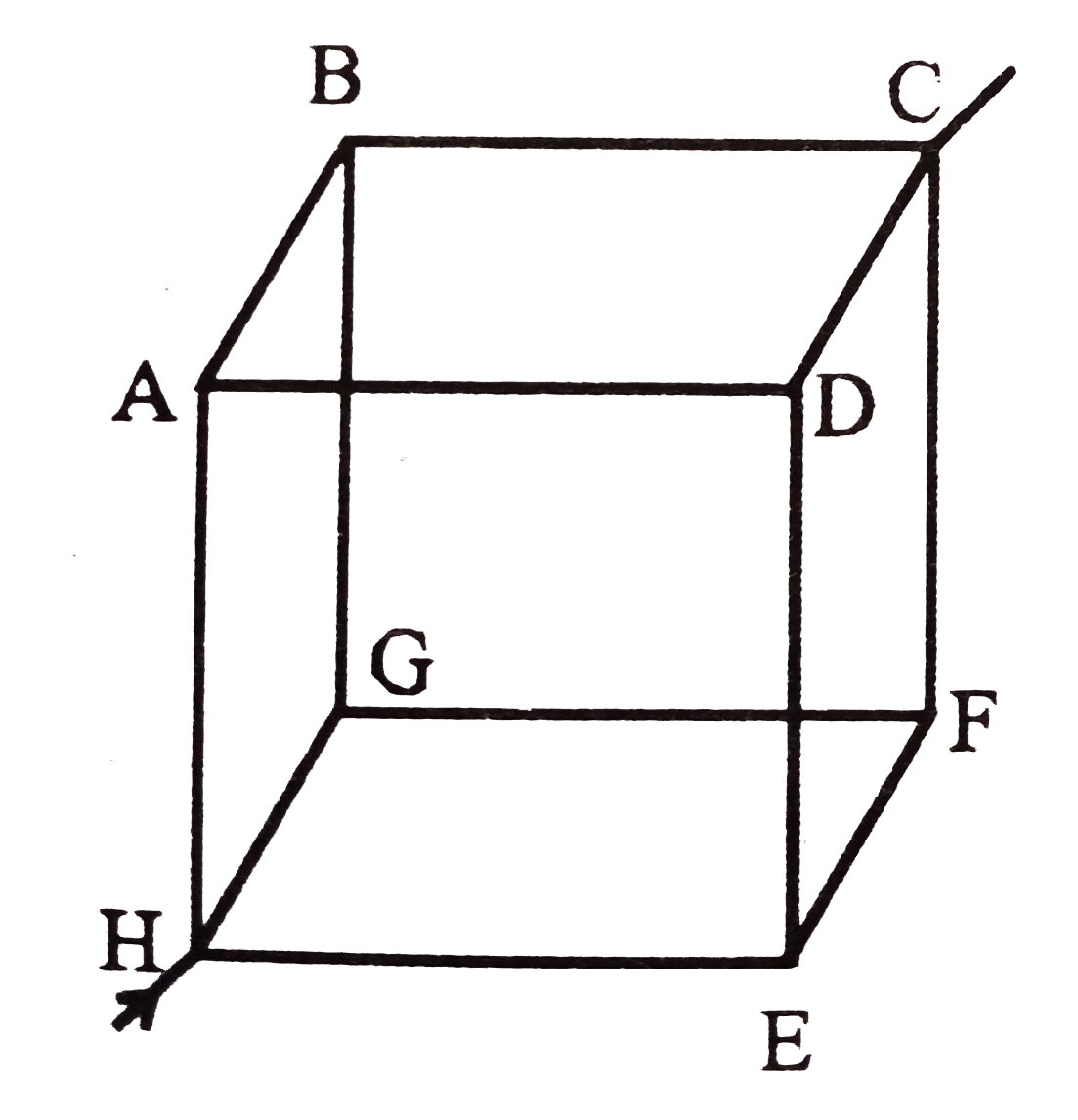 ABGBFCCEDDnone
ABGBFCCEDDnone
Find the value of i73+i74+i75+i76
(A) 0 (B) 2 (C) 2i (D) -2iView SolutionFind the value of i2+i4+i6+i71+i2+i3 is
( a ) 1−i
( b ) 1+i
( c ) 2−i
( d ) 2+iView SolutionProve that:
(i) 1+i2+i4+i6=0
(ii) 1+i10+i100+i1000=2
(iii) i104+i109+i114+i119=0
(iv) 6i54+5i37−2i11+6i68=7i
(v)i592+i590+i588+i586+i584i582+i580+i578+i576+i574=−1View SolutionWrite the following in the form x+iy:
(i) i+i2+i3+i4
(ii) i4+i8+i12+i16
(iii) i+i5+i9+i13
(iv) i9+i10+i11+i12.View SolutionFind the values of following expressions: i49+i68+i89+i110 (ii) i30+i80+i120 (iii) i+i2+i3+i4 (iv) i5+i10+i15 (v) i592+i590+i586+i584i582+i580+i576+i574 (vi) 1+i2+i4+i6+i8+.i20 (vii) (1+i)6+(1−i)3
View SolutionWrite the following in the form x+iy:
(i) (3+2i)(2−i)
(ii) 2i2+6i3+3i16−6i19+4i25.
(iii) (3−2i)(2+3i)(1+2i)(2−i).View SolutionProve that:
(i) (1−i)2=−2i
(ii) (1+i)4×(1+1i)4=16
(iii) {i19+(1i)25}2=−4
(iv) i4n+i4n+1+i4n+2+i4n+3=0
(v) 2i2+6i3+3i16−6i19+4i25=1+4i.View Solution

