Solve the inequation −3≤3−2x<9,x∈R. Represent the solution set on the real line.
Video Solution
Text Solution
We have
−3≤3−2x<9⇒−3≤3−2xand3−2x<9.
Now, −3≤3−2x⇒−3+2x≤3 [adding 2x on both sides]
⇒2x≤6 [adding 3 on both sides]
⇒x≤3 [dividing both sides by 2]
⇒x∈(−∞,3].
And, 3−2x<9⇒−2x<6 [substracting 3 from both sides]
⇒x>−3 [dividing both sides by -2]
⇒x∈(−3,∞).
∴ solution set =(−∞,3]∩(−3,∞)=(−3,3].
We may represent it on the number line as shown below.
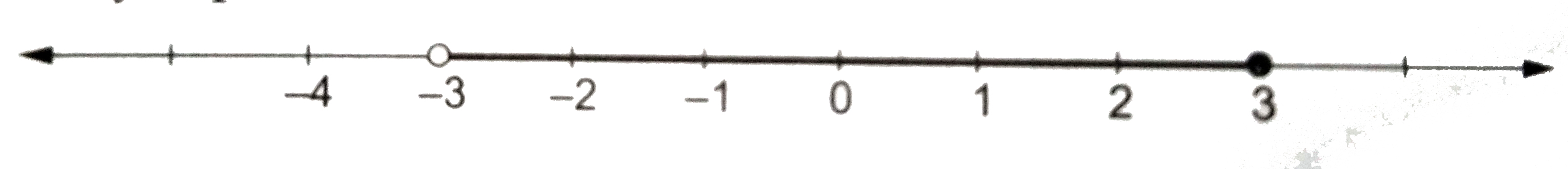
|
Topper's Solved these Questions
- LINEAR INEQUATIONS (IN ONE VARIABLE)BOOK - RS AGGARWALCHAPTER - LINEAR INEQUATIONS (IN ONE VARIABLE)EXERCISE - WORD PROBLEMS (Example)
9 Videos
- LINEAR INEQUATIONS (IN ONE VARIABLE)BOOK - RS AGGARWALCHAPTER - LINEAR INEQUATIONS (IN ONE VARIABLE)EXERCISE - EXERCISE 6A
46 Videos
- LIMITBOOK - RS AGGARWALCHAPTER - LIMITEXERCISE - EXERCISE 27C
15 Videos
- LINEAR INEQUATIONS (IN TWO VARIABLES )BOOK - RS AGGARWALCHAPTER - LINEAR INEQUATIONS (IN TWO VARIABLES )EXERCISE - EXERCISE 7
22 Videos
Similar Questions
Solve the inequation : −2≤6x−1<2
View SolutionSolve the inequation: 3x−2<0
View SolutionSolve the inequation : −7<2x−3<7
View SolutionSolve the inequation 2≤|x−3|≤4
View SolutionSolve the inequation 2x−3<0
View SolutionSolve the system of inequations 2x−1>x+7−x3>2,x∈R.
Represent the solution set on the number line.
View SolutionSolve the inequation : 6≤−3(2x−4)<12
View SolutionSolve the inequation: 3(x−2)≤5x+8
View SolutionSolve the inequation: 3x+17≤2(1−x)
View SolutionSolve the inequation 3(x−1)≤2(x−3) If x ∈ R and represent the solution on the number line
View Solution
