In a high school, a committee has to be formed from a group of 6 boys M1,M2,M3,M4,M5,M6 and 5 girls G1,G2,G3,G4,G5.
(i) Let a1 be the total number of ways in which the committee can be formed such that the committee has 5 members, having exactly 3 boys and 2 girls
(ii) Let a2 be the total number of ways in which the committee can be formed such that the committee has at least 2 members, and having an equal number of boys and girls.
(iii) Let a3 be the total number of ways in which the committee can be formed such that the committee has 5 members, at least 2 of them being girls.
(iv) Let a4 be the total number of ways in which the committee can be formed such that the committee has 4 members, having at least 2 girls such that both M1 and G1 are NOT in the committee together.
The correct option is
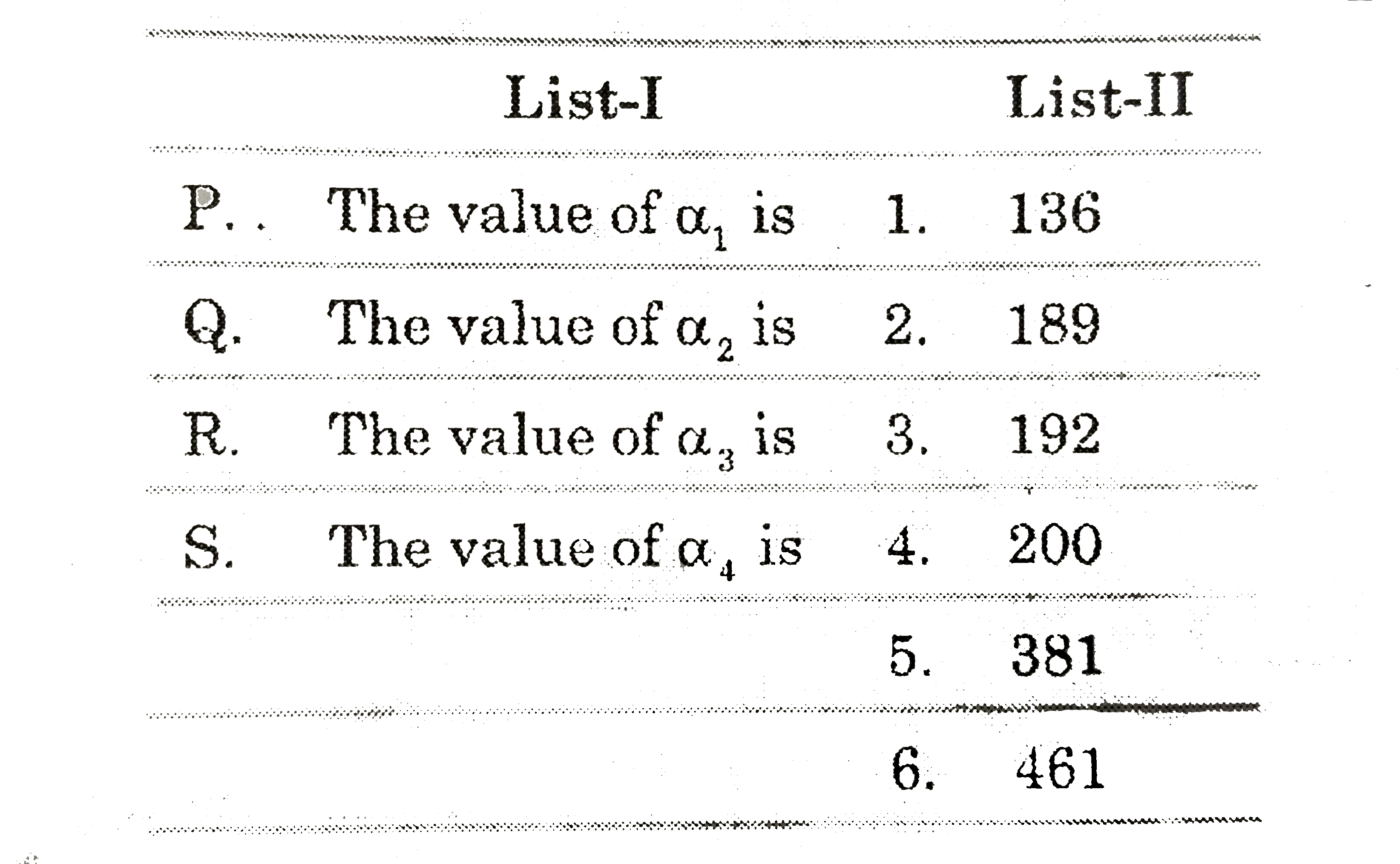
P→4,Q→6,R→2,S→1
P→1,Q→4,R→2,S→3
P→4,Q→6,R→5,S→2
P→4,Q→2,R→3,S→1
The correct Answer is:C
Given 6 boys M1,M2,M3,M4,M5,M6 and 5 girls G1,G2,G3,G4,G5
(i) α1→ Total number of ways of selecting 3 boys and 2 girls from 6 boys and 5 girls.
i.e., 6C3×5C2=20×10=200
∴ α1=200
(ii) α2→ Total number of ways selecting at least 2 member and having equal number of boys and girls
i.e., 6C15C1+6C25C2+6C35C3+6C45C4+6C55C5
= 30 + 150 + 200 + 75 + 6 = 461
⇒ α2=461
(iii) α3→ Total number of ways of selecting 5 members in which at least 2 of them girls
i.e., 5C26C3+5C36C2+5C46C1+5C56C0
= 200 + 150 + 30 + 1 = 381
α3=381
(iv) α4→ Total number of ways of selecting 4 members in which at least two girls such that M1 and G1 are not included together.
G1 is included →4C1⋅5C2+4C2⋅5C1+4C3
= 40 + 30 + 4 = 74
M1 is included →4C2⋅5⋅C1+4C3=30+4=34
G1 and M1 both are not included
4C4+4C3⋅5C1+4C2⋅5C2
1 + 20 + 60 = 81
∴ Total number = 74 + 34 + 81 = 189
α4=189
Now P→4,Q→6,R→5,S→2
Hence, option (c) is correct.
Topper's Solved these Questions
- PERMUTATIONS AND COMBINATIONSBOOK - IIT JEE PREVIOUS YEARCHAPTER - PERMUTATIONS AND COMBINATIONSEXERCISE - Properties of Combinational and General Selections (Integer)
1 Videos
- PERMUTATIONS AND COMBINATIONSBOOK - IIT JEE PREVIOUS YEARCHAPTER - PERMUTATIONS AND COMBINATIONSEXERCISE - Properties of Combinational and General Selections (Fill in the Blanks )
2 Videos
- PERMUTATIONS AND COMBINATIONSBOOK - IIT JEE PREVIOUS YEARCHAPTER - PERMUTATIONS AND COMBINATIONSEXERCISE - Properties of Combinational and General Selections (Objective Questions I Only one correct option )
13 Videos
- PARABOLABOOK - IIT JEE PREVIOUS YEARCHAPTER - PARABOLAEXERCISE - TOPIC 4 DIAMETER, CHORD OF CONTACT, CHORD BISECTED AND PRODUCT OF PAIR OF TANGENTS OBJECTIVES QUESTION II (ANALYTICAL & DESCRIPTIVE )
5 Videos
- PROBABILITYBOOK - IIT JEE PREVIOUS YEARCHAPTER - PROBABILITYEXERCISE - TOPIC 5 PROBABILITY DISTRIBUTION AND BINOMIAL DISTRIBUTTION OBJECTIVE QUESTIONS ONLY ONE CORRECT OPTION (INTEGER )
1 Videos
Similar Questions
In a high school, a committee has to be formed from a group of 6 boys M1, M2, M3, M4, M5, M6 and 5 girls G1, G2, G3, G4, G5 . Let α1 be the total number of ways in which the committee can be formed such that the committee has 5 members, having exactly 3 boys and 2 girls. (ii) Let α2 be the total number of ways in which the committee can be formed such that the committee has at least 2 members, and having an equal number of boys and girls. (iii) Let α3 be the total number of ways in which the committee can be formed such that the committee has 5 members, at least 2 of them being girls. (iv) Let α4 be the total number of ways in which the committee can be formed such that the committee has 4 members, having at least 2 girls and such that both M1 and G1 are NOT in the committee together. LIST-I LIST-II P. The value of α1 is 1. 136 Q. The value of α2 is 2. 189 R. The value of α3 is 3. 192 S. The value of α4 is 4. 200 5. 381 6. 461 The correct option is: P→4;Q→6;R→2;S→1 (b) P→1;Q→4;R→2;S→3 (c) P→4;Q→6;R→5;S→2 (d) P→4;Q→2;R→3;S→1
View SolutionIn a high school, a committee has to be formed from a group of 6 boys M1, M2, M3, M4, M5, M6 and 5 girls G1, G2, G3, G4, G5 . Let α1 be the total number of ways in which the committee can be formed such that the committee has 5 members, having exactly 3 boys and 2 girls. (ii) Let α2 be the total number of ways in which the committee can be formed such that the committee has at least 2 members, and having an equal number of boys and girls. (iii) Let α3 be the total number of ways in which the committee can be formed such that the committee has 5 members, at least 2 of them being girls. (iv) Let α4 be the total number of ways in which the committee can be formed such that the committee has 4 members, having at least 2 girls and such that both M1 and G1 are NOT in the committee together. LIST-I LIST-II P. The value of α1 is 1. 136 Q. The value of α2 is 2. 189 R. The value of α3 is 3. 192 S. The value of α4 is 4. 200 5. 381 6. 461 The correct option is: P→4; Q→6; R→2; S→1 (b) P→1; Q→4; R→2; S→3 (c) P→4; Q→6; R→5; S→2 (d) P→4; Q→2; R→3; S→1
View Solution
Knowledge Check
In a high school, a commintee has to be formed from a group of 6 boys M1,M2,M3,M4,M5,M6 and 5 girls G1,G2,G3,G4,G5.
(i) Let α1 be the total number of ways in which of the commitee can be formed such that the commitee has 5 members, having exactly 3 boys and 2 girls.
(ii) Let α2 be the total number of ways in which the commitee can be formed such that the committee has at least 2 members, and having an equal number of bosy and girls.
(iii) Let α3 be the total number of ways in wihc the committee can be formed such that the commitee has 5 members, at least 2 of them being girls.
(iv) Let α4 be the total number of ways in which the committee can be formed such that the committee has 4 members, having at least 2 girls and such that both M1 and G1 are NOT in the committee together.
List - I List - II
P. The value of α1 is 1. 136
Q. The value of α2 is 2. 189
R. The value ofα3 is 3. 192
S. The value of α4 is 4. 200
5. 381
6. 461AP → 4 , Q → 6, R → 2, S → 1BP → 1 , Q → 4, R → 2, S → 3CP → 4 , Q → 6, R → 5, S → 2DP → 4 , Q → 2, R → 3, S → 1A committee of 5 members is to be selected from among 6 boys and 5 girls. Determine the number of ways of selecting the committee if it is to consist of at least one boy and one girl.
A455B350C305Dnone of theseA committee of at least three members is to be formed from a group of 6 boys and 6 girls such that it always has a boy and a girl. The number of ways to form such a committee is
A211−26−13B212−27−35C211−27−35D212−27−13
A committee of 5 is to be formed from 6 boys and 5 girls. The number of ways that committee can be formed so that the committee contains at least one boy and one girl having the majority of boys is
View SolutionA committee of 5 members is to be selected from among 6 boys and 5 girls. Determine the number of ways of selecting the committee if it is to consist of at least one boy and one girl.
View SolutionFrom 6 boys and 7 girls a committee of 5 is to be formed so as to include at least one girl. Find the number of ways in which this can be done.
View SolutionIn how many ways a committee of 5 is to be formed from 6 boys and 4 girls, where the committee contains at least one girl.
View SolutionA committee consisting of at least three members is to be formed from a group of 6 boys and 6 girls such that it always has a body and a girl. The number of ways to form such committtee is :
View Solution
