A block of mass 2.0 kg is given an initial speed along the floor towards a spring as shown. The coefficient of kinetic friction between the floor and the block is 0.4 and force constant of the spring is 5.6×103 N/m. The block compresses the spring by 10cm before it stops for a moment. What is the initial speed (m/s) of the block?
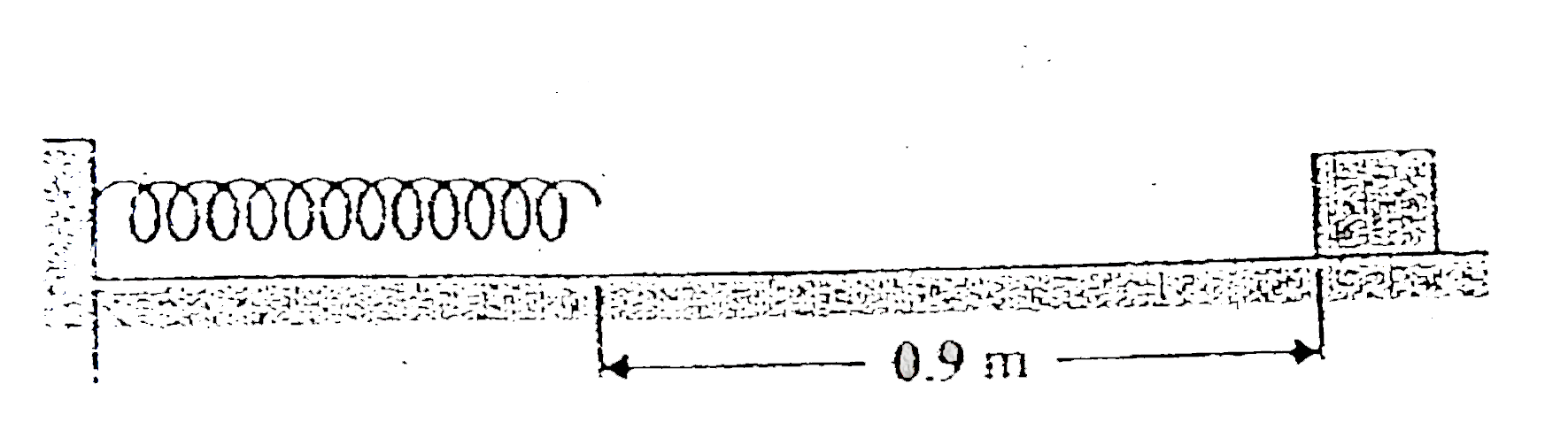
Because the block is moving, a kinetic frictional force acts on it. The magnitude is given by Eq. 6-2 (fk=μkFN, where FN is the normal force). The direction is opposite the motion (the friction opposes the sliding).
Calculating FN: Because we need the magnitude fk of the frictional force, we first must calculate the magnitude FN of the normal force. Figure 6-5b is a free-body diagram showing the forces along the vertical y axis. The normal force is upward, the gravitational force with magnitude mg is downward, and (note) the vertical component Fy of the applied force is upward. That component is shown in Fig. 6-5c, where we can see that Fy=Fsinθ. We can write Newton.s second law (Fnet=m→a) for those forces along the y axis as
FN+Fsinθ−mg=m(0),

Figure 65 (a) A force is applied to a moving block. (b) The vertical forces. (c) The components of the applied force. (d) The horizontal forces and acceleration.
where we substituted zero for the acceleration along the y axis (the block does not even move along that axis). Thus,
FN=mg−Fsinθ.
Calculating acceleration a: Figure 6-5d is a free-body diagram for motion along the x axis. The horizontal component Fx of the applied force is rightward, from Fig. 6-5c, we see that Fx=Fcosθ. The frictional force has magnitude fx(=kFN) and is leftward. Writing Newton.s second law for motion along the x axis gives us
Fcosθ−μkFN=ma
Substituting for FN from Eq. 6-15 and solving for a lead to
a=Fmcosθ−μk(g−Fmsinθ)
Finding a maximum: To find the value of θ that maximizes a, we take the derivative of a with respect to θ and set the result equal to zero:
dadθ=−Fmsinθ+μkFmcosθ=0.
Rearranging and using the identity (sinθ)/(cosθ)=tanθ give us
tanθ=μk.
Solving for θ and substituting the given μk=0.40, we find that the acceleration will be maximum if
θ=tan−1μk
Comment: As we increase θ from 0, more of the applied force →F is upward, relieving the normal force. The decrease in the normal force causes a decrease in the frictional force, which opposes the block.s motion. Thus, the block.s acceleration tends to increase. However, the increase in θ also decreases the horizontal component of →F, and so the block.s acceleration tends to decrease. These opposing tendencies produce a maximum acceleration at θ=22∘
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - FORCE AND MOTION-IIEXERCISE - CHECKPOINTS5videos
FORCE AND MOTION-II
- BOOK - RESNICK AND HALLIDAYCHAPTER - FORCE AND MOTION-IIEXERCISE - PROBLEMS33videos
FORCE AND MOTION-II
- BOOK - RESNICK AND HALLIDAYCHAPTER - FORCE AND MOTION - I EXERCISE - PRACTICE QUESTIONS (Integer Type)3videos
FORCE AND MOTION - I
- BOOK - RESNICK AND HALLIDAYCHAPTER - GAUSS' LAW EXERCISE - PRACTICE QUESTIONS (INTEGER TYPE)3videos
GAUSS' LAW
Similar Questions
A block of mass 2 kg is placed on the floor (μ=0.4). A horizontal force of 7 N is applied on the block. The force of friction between the block & floor is fs. Find the fs.
View SolutionA block of mass 0.18 kg is attached to a spring of force constant 2N/m. The coefficient of friction between the block and the floor is 0.1. Initially, the block is at rest and the spring is unstretched. An impulse is given to the block.
The block slides a distance of 0.06 m and comes to rest for the first time. The initial velocity of the block in m/s is v=N/10. Then, N isView Solution
Knowledge Check
- Question 1 - Select One or More
A block of mass 2 kg, initially at rest on a horizontal floor, is now acted upon by a horizontal force of 10N. The coefficient of friction between the block and the floor is 0.2. If g=10m/s2, then :
AThe work done by the applied force in 4 s is 240 J.BThe work done by the firctional force in 4s is 0,CThe work done by the net forces in 4s is 0.sDThe change in kinetic energy of the block in 4s is 144 J. - Question 2 - Select One
A block of mass 50 kg is projected horizontal on a rough horizontal floor. The coefficient of friction between the block and the floor is 0.1. The block strikes a light spring of stiffness k=100N/m with a velocity 2m/s, the maximum compression of the spring is
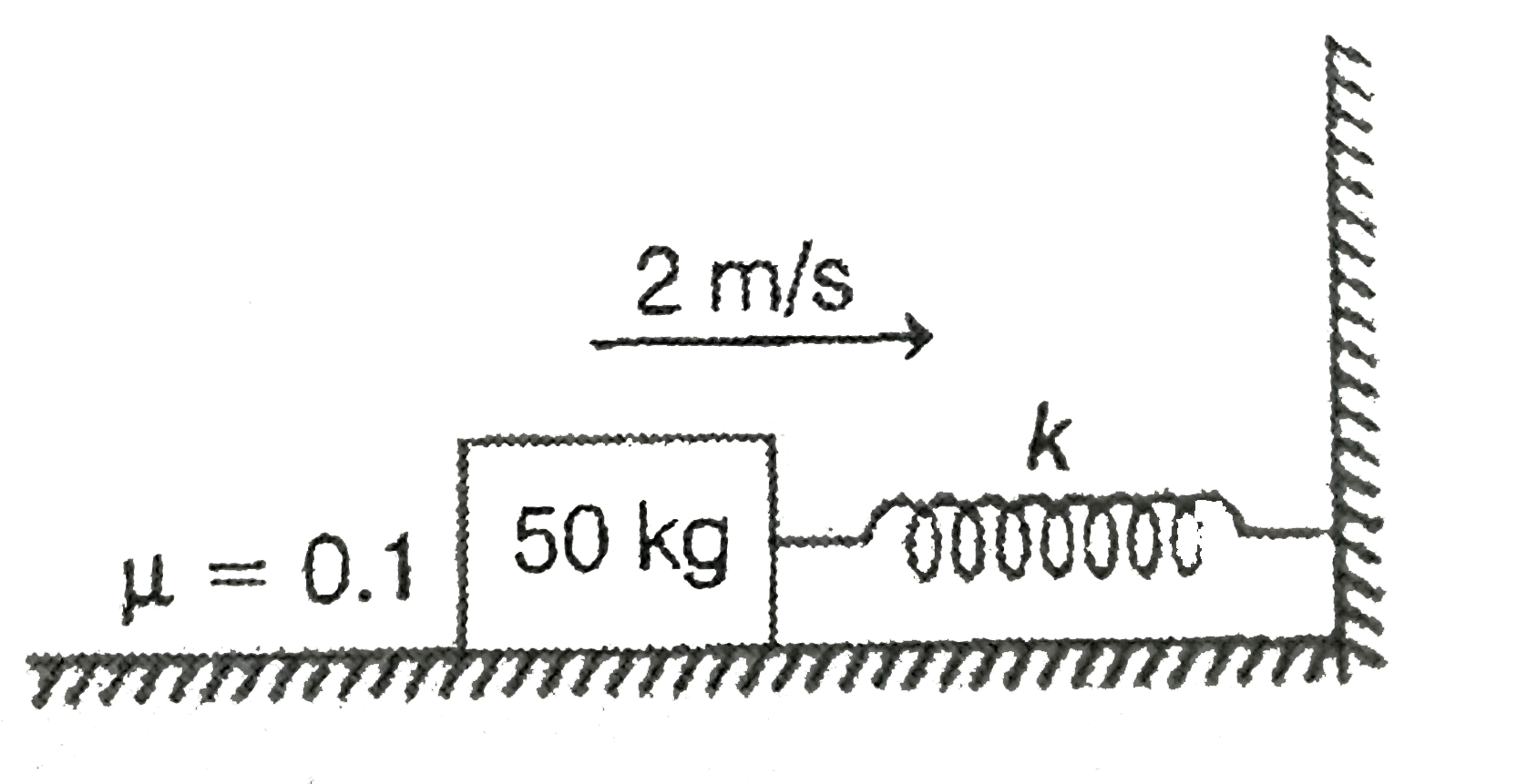 A1 mB2 mC3 mD4 m
A1 mB2 mC3 mD4 m - Question 3 - Select One
A block of mass 2 kg is placed on the floor. The coefficient of static friction is 0.4. A force F of 3 N is applied on the block as shown in figure. The force of friction between the block and the floor is (Take g=10ms−2)
 A1.5 NB3 NC4 ND2.5 N
A1.5 NB3 NC4 ND2.5 N
A block of mass 2 kg is at rest on a floor . The coefficient of static friction between block and the floor is 0.54. A horizonatl force of 2.8 N is applied to the block . What should be the frictional force between the block and the floor ? ( take , g=10m/s2)
View SolutionA block of mass 50 kg is projected horizontal on a rough horizontal floor. The coefficient of friction between the block and the floor is 0.1. The block strikes a light spring of stiffness k=100N/m with a velocity 2m/s, the maximum compression of the spring is
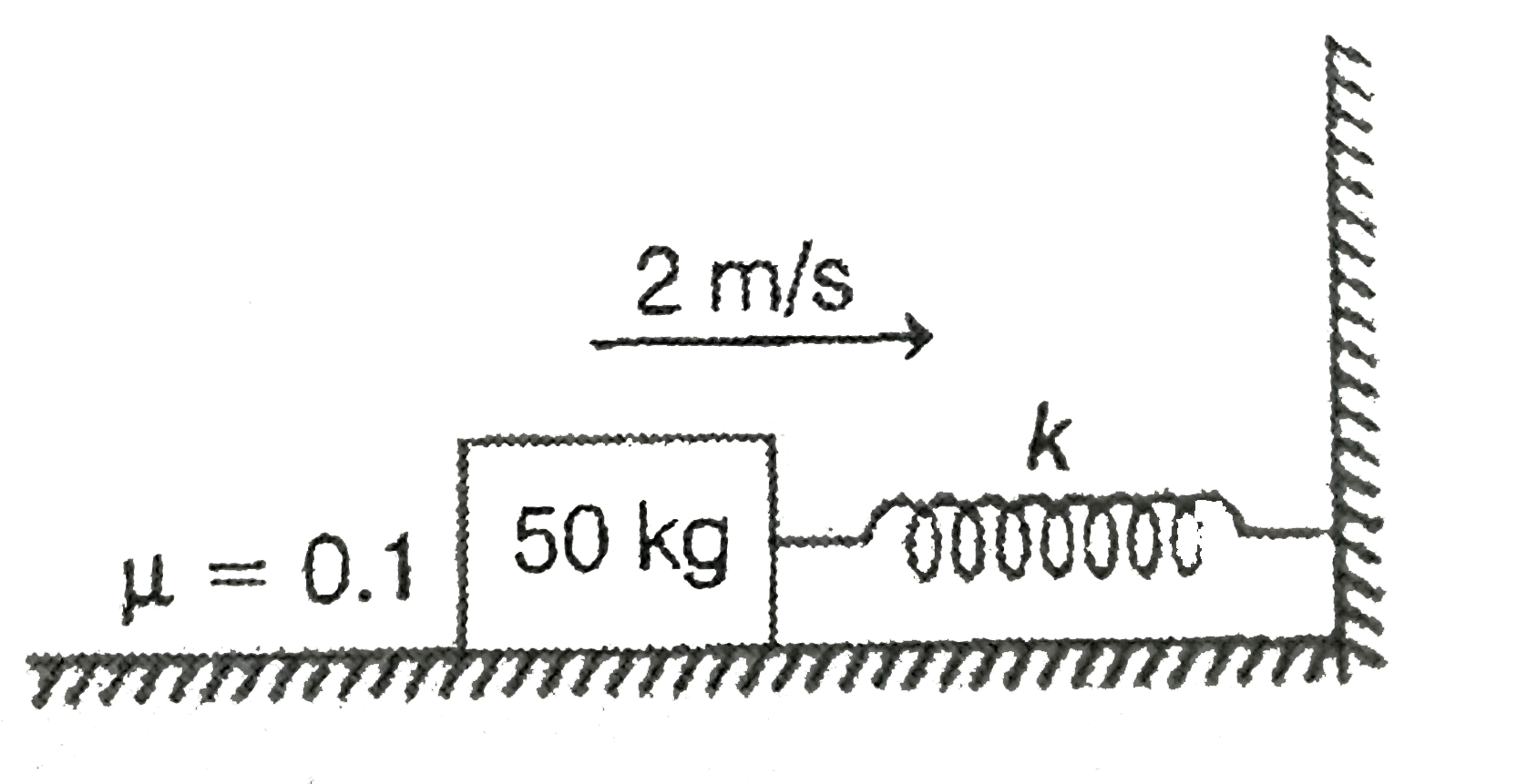 View Solution
View SolutionA block of mass m is moving with a speed v on a horizontal rough surface and collides with a horizontal mounted spring of spring constant k as shown in the figure .The coefficient of friction between the block and the floor is μ The maximum compression of the spring is
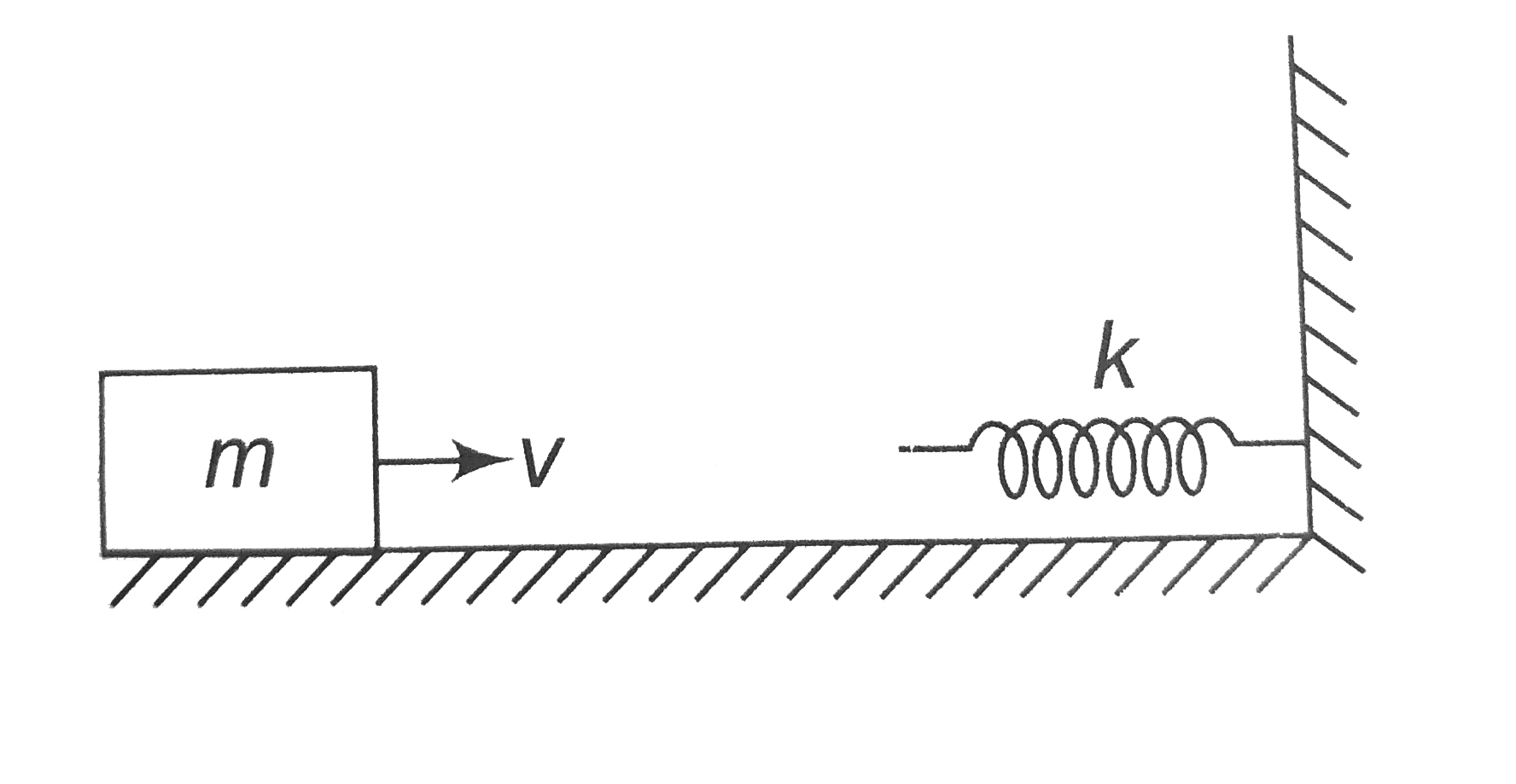 View Solution
View SolutionA block of mass 1kg moves towards a spring of force constant 10N/m. The spring is massless and unstretched. The coeffcient of friction between block and surface is 0.30. After compressing the spring, block does not return back : (g=10m/s).
View SolutionA block of mass 2 kg is at rest on a floor . The coefficient of static friction between block and the floor is 0.54. A horizonatl force of 2.8 N is applied to the block . What should be the frictional force between the block and the floor ? ( take , g=10m/s2)
View Solution

