A compressive force, F is applied at the two ends of a long thin steel rod. It is heated, simultaneously, such that its temeprature increass by ΔT. The net change in its length is zero. Let l be the length of the rod, A its area of cross-section, Y its Young's modulus, and α its coefficient of linear expansion. Then, F is equal to
IAYαΔT
AYαΔT
AYαΔT
I2YαΔT
The correct Answer is:C
Step by step video, text & image solution for A compressive force, F is applied at the two ends of a long thin steel rod. It is heated, simultaneously, such that its temeprature increass by DeltaT. The net change in its length is zero. Let l be the length of the rod, A its area of cross-section, Y its Young's modulus, and alpha its coefficient of linear expansion. Then, F is equal to by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A rod of length L kept on a smooth horizontal surface is pulled along its length by a force F. The area of cross-section is A and Young's modulus is Y. The extension in the rod is

View SolutionA thin uniform rod of mass M and length l is rotating about a frictionless axis passing through one of its ends and perpendicular to the rod. The rod is heated uniformly to increases its temperature by Δθ. Calculate the percentage change in rotational kinetic energy of the rod. Explain why the answer is not zero. Take coefficient of linear expansion of the material of the rod to be α.
View Solution
Knowledge Check
A uniform wire of mass m, length L, area of cross-section A and Young's modulus Y hange from the ceiling. Its elongation under its own weight will be
AzeroBmgL2AYCmgLAYD2mgLAYA metal rod of length 'L', cross-sectional area 'A', Young's modulus 'Y' and coefficient of linear expansion 'α' is heated to 't'∘C. The work that can be perfomred by the rod when heated is
AYAαLt22BYAα2Lt22CYAα2L2t22DYAαLT2A thin rod of negligible mass and area of cross section S is suspended vertically from one end. Length of the rod is L0 at T∘C . A mass m is attached to the lower end of the rod so that when temperature of the rod is reduced to 0∘C its length remains L0 Y is the Young’s modulus of the rod and α is coefficient of linear expansion of rod. Value of m is :
ASYαTgBSYαT2gCSYα2gTDSYαT2gL0
on heating a cylindrical metallic rod, its length increases by 2% . Then area of cross section of rod will
View SolutionA rod PQ of length L revolves in a horizontal plane about the axis YY´. The angular velocity of the rod is w. If A is the area of cross-section of the rod and r be its density, its rotational kinetic energy is
View SolutionA wooden wheel of radius R is made of two semicircular part . The two parts are held together by a ring made of a metal strip of cross sectional area S and length L. L is slightly less than 2πR. To fit the ring on the wheel, it is heated so that its temperature rises by ΔTand it just steps over the wheel. As it cools down to surrounding temperature, it process the semicircle parts together. If the coefficient of linear expansion of the metal is α, and it Young's modulus is Y, the force that one part of the wheel applies on the other part is :
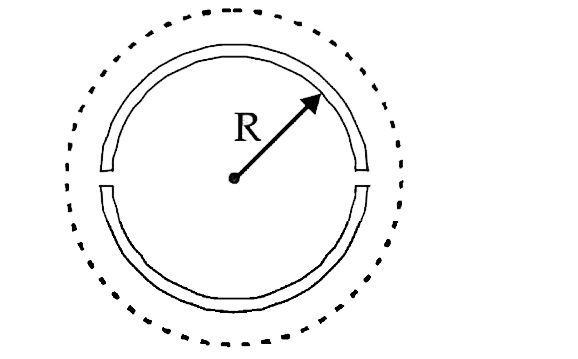
View SolutionYoung's modulus for a wire of length L and area of cross-section A is Y. What will be Young's Modulus for wire of same material, but half its original length and double its area?
View SolutionA metallic rod of length 'L' and cross-section 'A' has Young's modulus 'Y' and coefficent of linear expansion 'α'. If the rod is heated to a temperature. 'T' the energy stored per unit volume is:
View Solution
