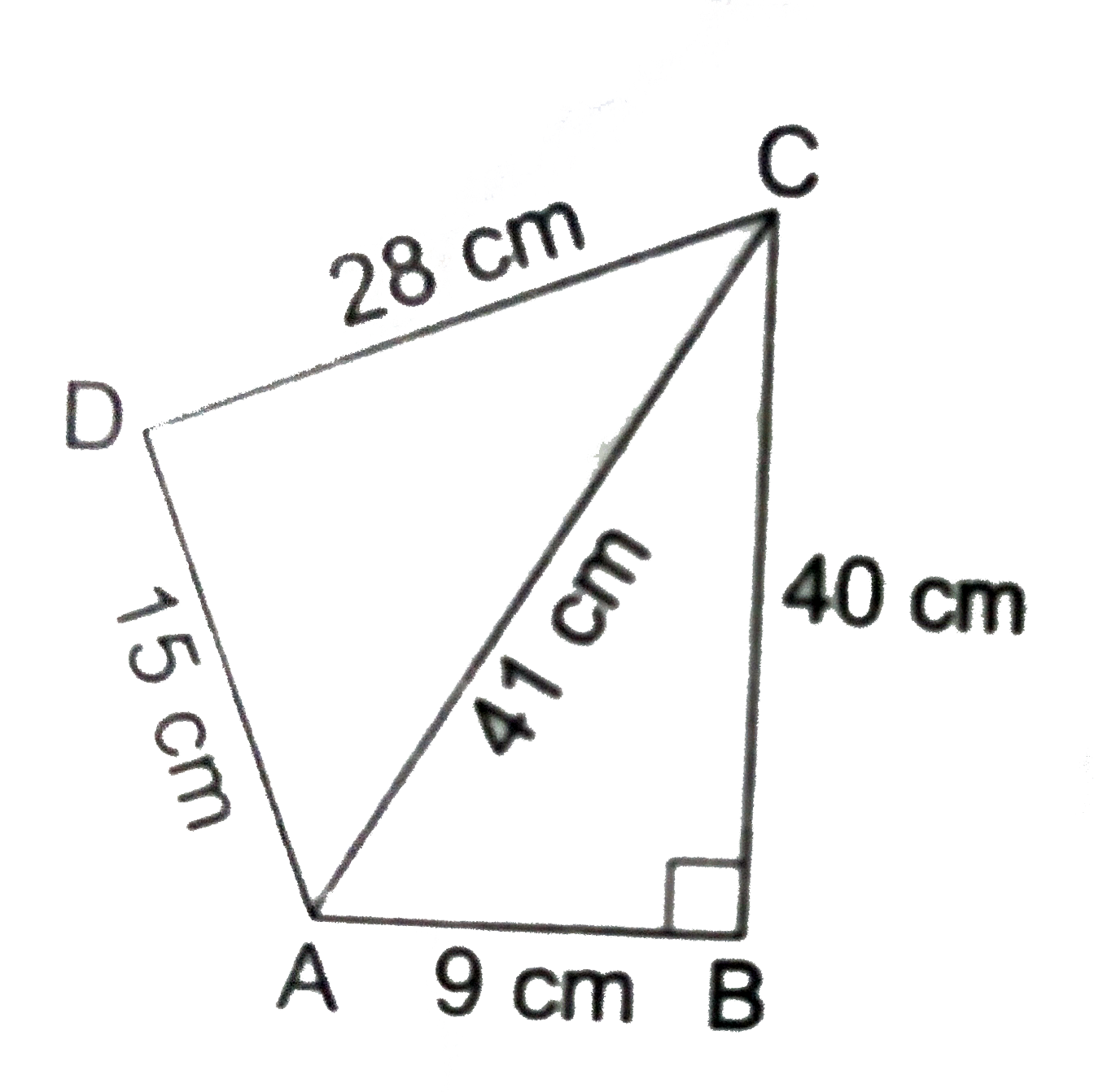
Find the area of the quadrilateral whose sides measure 9 cm , 40 cm, 28 cm and 15 cm , and in which the angle between the first two sides is right angle .

Video Solution

Text Solution

Verified by Experts

Let ABCD be the given quadrilateral in which AB= 9 cm , BC=40 cm ,CD=28 cm DA= 15 cm and $∠ A B C = 90^{\circ}$ .
By pythagoras' theorem ,
$A C = \sqrt{A B^{2} + B C^{2}} units = \sqrt{{(9)}^{2} + {(40)}^{2}} c m$
$= \sqrt{1681} c m = 41 c m .$
$Area of Δ A B C = (\frac{1}{2} \times A B \times B C)$
$= (\frac{1}{2} \times 9 \times 40) c m^{2} = 180 c m^{2} .$
$In Δ A C D,$
Let $a = A C = 41 c m, b = C D = 28 c m and c = D A = 15 c m .$
$∴ s = \frac{1}{2} (41 + 28 + 15) c m = 42 c m .$
$∴ (s - a) = 1 c m, (s - b) = 14 c m and (s - c) = 27 c m .$
Area of $Δ A C D = \sqrt{s (s - a) (s - a) (s - c)} sq units$
$= \sqrt{42 \times 1 \times 14 \times 27} c m^{2}$
$= (14 \times 3 \times 3) c m^{2} = 126 c m^{2}$ .
Area of the quadrilateral ABCD
$= area of Δ A B C + Area of Δ A C D$
$= (180 + 126) c m^{2} = 306 c m^{2}$ .

Updated on:21/07/2023