In a four sider- field , the length of the longer diagonal is 128 m. the lengths of perpendiculars from the opposite vertices upon this diagonal are 22.7 m and 17.3 and Find the area of the field.
The correct Answer is:2560m2
To find the area of the four-sided field given the lengths of the diagonals and the perpendiculars from the opposite vertices, we can break the problem down into a few clear steps.
1. Identify the Given Values:
- Length of the longer diagonal (AC) = 128 m
- Length of the perpendicular from vertex D to diagonal AC (DE) = 22.7 m
- Length of the perpendicular from vertex B to diagonal AC (BE) = 17.3 m
2. Calculate the Area of Triangle ADC:
- The area of triangle ADC can be calculated using the formula:
AreaADC=12×base×height
- Here, the base is the diagonal AC (128 m) and the height is DE (22.7 m):
AreaADC=12×128×22.7
- Calculate:
AreaADC=12×128×22.7=1456.8m2
3. Calculate the Area of Triangle ABC:
- Similarly, the area of triangle ABC can be calculated using the same formula:
AreaABC=12×base×height
- Here, the base is still the diagonal AC (128 m) and the height is BE (17.3 m):
AreaABC=12×128×17.3
- Calculate:
AreaABC=12×128×17.3=1107.2m2
4. Calculate the Total Area of the Field:
- The total area of the four-sided field (ABCD) is the sum of the areas of triangles ABC and ADC:
AreaABCD=AreaADC+AreaABC
- Substitute the calculated areas:
AreaABCD=1456.8+1107.2=2564m2
Final Answer:
The area of the field is 2564 m².
- Length of the longer diagonal (AC) = 128 m
- Length of the perpendicular from vertex D to diagonal AC (DE) = 22.7 m
- Length of the perpendicular from vertex B to diagonal AC (BE) = 17.3 m
- The area of triangle ADC can be calculated using the formula:
AreaADC=12×base×height
- Here, the base is the diagonal AC (128 m) and the height is DE (22.7 m):
AreaADC=12×128×22.7
- Calculate:
AreaADC=12×128×22.7=1456.8m2
- Similarly, the area of triangle ABC can be calculated using the same formula:
AreaABC=12×base×height
- Here, the base is still the diagonal AC (128 m) and the height is BE (17.3 m):
AreaABC=12×128×17.3
- Calculate:
AreaABC=12×128×17.3=1107.2m2
- The total area of the four-sided field (ABCD) is the sum of the areas of triangles ABC and ADC:
AreaABCD=AreaADC+AreaABC
- Substitute the calculated areas:
AreaABCD=1456.8+1107.2=2564m2
The area of the field is 2564 m².
Topper's Solved these Questions
- PERIMETER AND AREA OF PLANE FIGURES BOOK - RS AGGARWALCHAPTER - PERIMETER AND AREA OF PLANE FIGURES EXERCISE - TEST YOURSELF (SHORT -ANSWER QUESTIONS)
1 Videos
- PERIMETER AND AREA OF PLANE FIGURES BOOK - RS AGGARWALCHAPTER - PERIMETER AND AREA OF PLANE FIGURES EXERCISE - Multiple Choice Questions (Mcq)
20 Videos
- MEAN,MEDAN,MODE OF GROUPED,DATA CUMULATIVE FREQUENCY GRAPH AND OGIVE BOOK - RS AGGARWALCHAPTER - MEAN,MEDAN,MODE OF GROUPED,DATA CUMULATIVE FREQUENCY GRAPH AND OGIVE EXERCISE - Test Yourself
18 Videos
- POLYNOMIALSBOOK - RS AGGARWALCHAPTER - POLYNOMIALSEXERCISE - Test Yourself
20 Videos
Similar Questions
In a four - sided field , the length of the longer diagonal is 120 m . The lengths of perpendicular from the opposite vertices on this diagonal are 20 m and 15 m .Find the area of the field .
View SolutionFind the area of a quadrilateral one of whose diagonals is 40 cm and the lengths of the perpendiculars drawn from the opposite vertices on the diagonal are 16 cm and 12 cm.
View Solution
Knowledge Check
In a four sider- field , the length of the longer diagonal is 128 m. the lengths of perpendiculars from the opposite vertices upon this diagonal are 22.7 m and 17.3 and Find the area of the field.
A128m2B2560m2C256m2DNone of theseThe diagonal of a quadrilateral is 20 cm in length and the lengths of perpendiculars on it from the opposite vertices are 8.5 cm and 11.5 cm. The area of the quadrilateral is
A400cm2B200cm2C300cm2D240cm2One side of a rectangular field is 9 m and one of its diagonal is 20 m. Find the area of the field.
A9√319sqmB7√319sqmC2√319sqmD5√319sqm
A diagonal of a quadrilateral is 30 cm in length and the lengths of perpendiculars to it from the opposite vertices are 6.8 cm and 9.6 cm. Find the area of the quadrilateral.
View SolutionA diagonal of a quadrilateral is 26 cm and the perpendiculars drawn to it from the opposite vertices are 12.8 cm and 11.2 cm. Find the area of the quadrilateral.
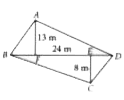
View SolutionThe diagonal of a quadrilateral shaped field is 24 m and the perpendiculars dropped on it from the remaining opposite vertices are 8 m and 13 m. Find the area of the field.
View SolutionFind the area of a square, the length of whose diagonal is 8√2 m.
View SolutionThe diagonal of a quadrilateral shaped field is 24 m and the perpendiculars dropped on it from the remaining opposite vertices are 8 m and 13 m. Find the area of the field.
View Solution
