A 3m long ladder weighing 20 kg leans on a frictionless wall. Its feet rest on the floor 1 m from the wall as shown in Fig.7.27. Find the reaction forces of the wall and the floor.Solution in Kannada
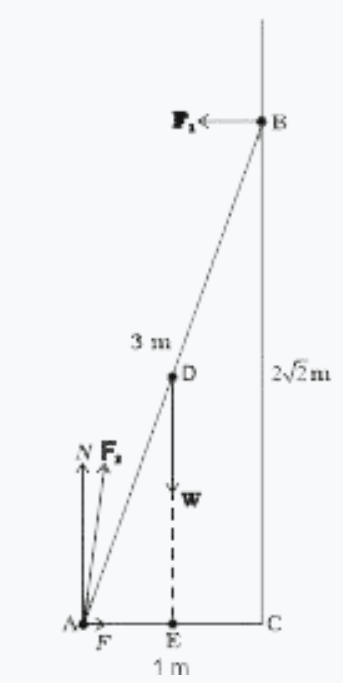
The ladder AB is 3 m long, its foot A is at distance AC=1m from the wall. From Pythagoras theorem, BC=2√2m. The forces on the ladder are its weight W acting at its centre of gravity D, reaction forces F1andF2 of the wall and the floor respectively. Force F1 is perpendicular to the wall, since the wall is frictionless. Force F2 is resolved into two components, the normal reaction N and the force of friction F. Note that F prevents the ladder from sliding away from the wall and is therefore directed toward the wall.
For translational equilibrium, taking the forces in the vertical direction,
N–W=0 (i)
Taking the forces in the horizontal direction, F–F1=0 (ii)
For rotational equilibrium, taking the moments of the forces about A,
2√2F1−(1/2)W=0 (iii)
Now W=20g=20×9.8N=196.0N
From (i) N=196.0N
From (iii) F1=W/4√2=196.0/4√2=34.6N
From (ii) F=F1=34.6N
F2=√F2+N2=199.0N
The force F2 makes an angle α with the horizontal,
tanα=N/F=4√2,α=tan−1(4/√2)≈80∘
Similar Questions
A 3m long ladder weighing 20 kg leans on a frictionless wall. Its feet rest on the floor 1 m from the wall as shown in Fig.7.27. Find the reaction forces of the wall and the floor.
View SolutionA 3m along ladder weighing 20 kg leans on a frictionless wall. Its feet rest on the floor 1 m from the wall. Find the rection forces of the wall and the floor.
View SolutionA uniform ladder of mass 10 kg leans against a smooth vertical wall making an angle of 530 with it. The other end rests on a rough horizontal floor. Find the normal force and the frictional force that the floor exerts on the ladder
View SolutionA uniform ladder of mass 10 kg leans against a smooth vertical wall making an angle 53∘ with it. The other end rests on a rough horizontal floor. Find the normal force and the frictional force that the floor exerts on the ladder.
View SolutionA 3m along ladder weighing 20kg leans on a frictionless wall. Its feet rest on the floor 1m from the wall. Find the rection forces of the wall and the floor.
View Solutionएक 3m लम्बी सीढ़ी जिसका द्रव्यमान 20 kg है, एक घर्षण रहित दीवार पर आनत है। इसका निचला सिरा फर्श पर दीवार से 1 m की दूरी पर टिका है। दीवार और फर्श के प्रतिक्रिया बलों का परिकलन कीजिए।
View Solution20 ফুট লম্বা একটি মই খাড়া দেওয়ালে হেলান দিয়ে আছে। মইটির শীর্ষবিন্দু সেকেন্ডে 2 ফুট হারে নিচের দিকে নামছে। মই -এর নিম্ন প্রান্তবিন্দু যখন দেওয়াল থেকে 12 ফুট দূরে তখন তার সরণের হার হল
View Solution
