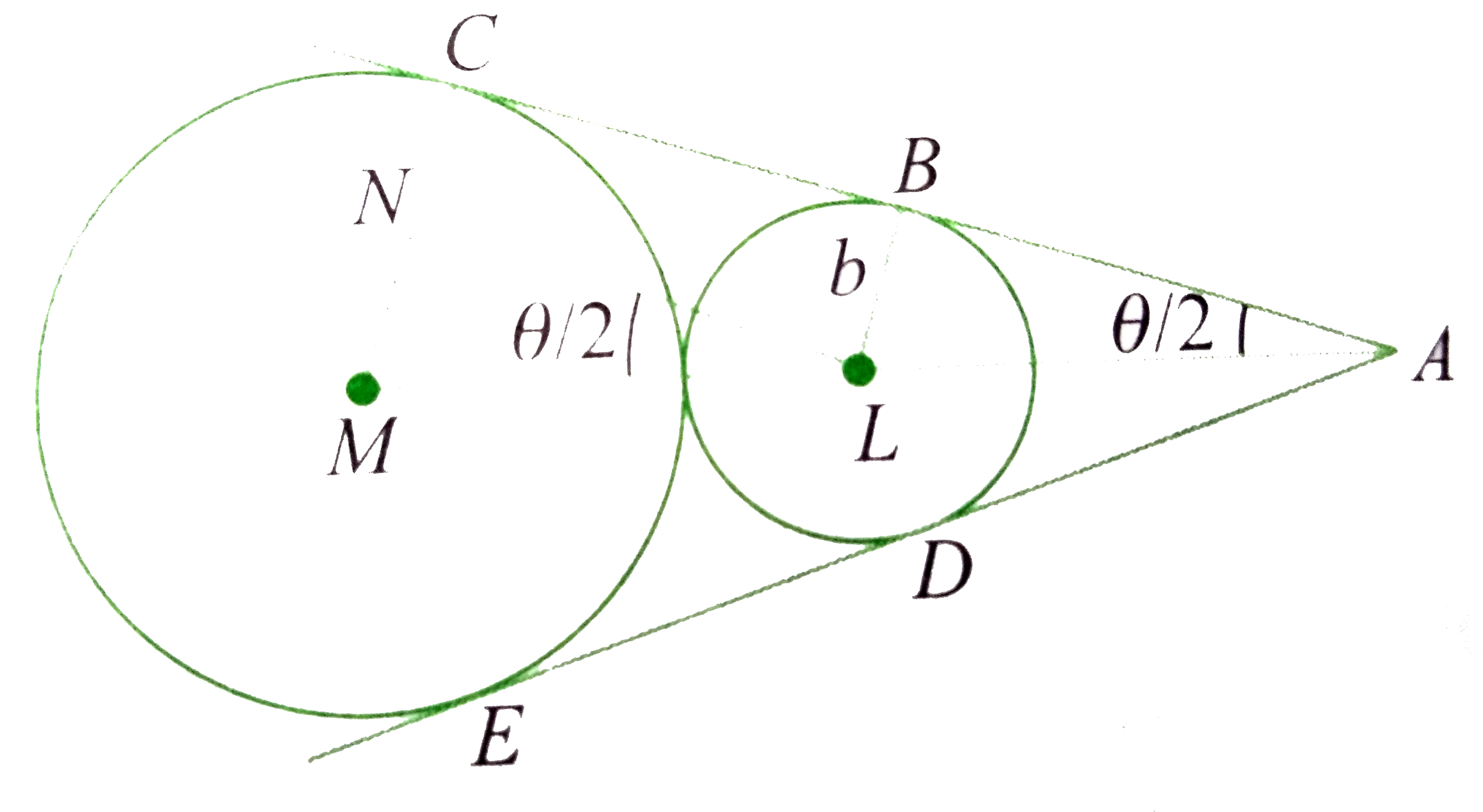
Two circles with radii $a a n d b$ touch each other externally such that $θ$ is the angle between the direct common tangents, $(a > b \geq 2)$ . Then prove that $θ = 2 {sin}^{- 1} (\frac{a - b}{a + b})$ .

Video Solution

Text Solution

Verified by Experts

From $Δ M L N$ ,
$sin . \frac{θ}{2} = \frac{a - b}{a + b}$
or $\frac{θ}{2} = {sin}^{- 1} (\frac{a - b}{a + b})$
Therefore, the angle between AB and AD is
$θ = 2 {sin}^{- 1} (\frac{a - b}{a + b})$

Updated on:21/07/2023

Two circles of radii $r_{1}$ and $r_{2}, r_{1} > r_{2} \geq 2$ touch each other externally. If $θ$ be the angle between the direct common tangents, then,
A $θ = {sin}^{- 1} (\frac{r_{1} + r_{2}}{r_{1} - r_{2}})$
B $θ = 2 {sin}^{- 1} (\frac{r_{1} - r_{2}}{r_{1} + r_{2}})$
C $θ = {sin}^{- 1} (\frac{r_{1} - r_{2}}{r_{1} + r_{2}})$
Dnone of these