Two lines through $(2, 3)$ from which the circle $x^{2} + y^{2} = 25$ intercepts chords of length $8$ units have equations
(A) $2 x + 3 y = 13$ , $x + 5 y = 17$
(B) $y = 3$ , $12 x + 5 y = 39$
(C) $x = 2$ , $9 x - 11 y = 51$
(D) $y = 0$ , $12 x + 5 y = 39$

A

$y = 3$

B

$12 x + 5 y = 39$

C

$x = 2$

D

$9 x - 11 y = 51$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:1,2

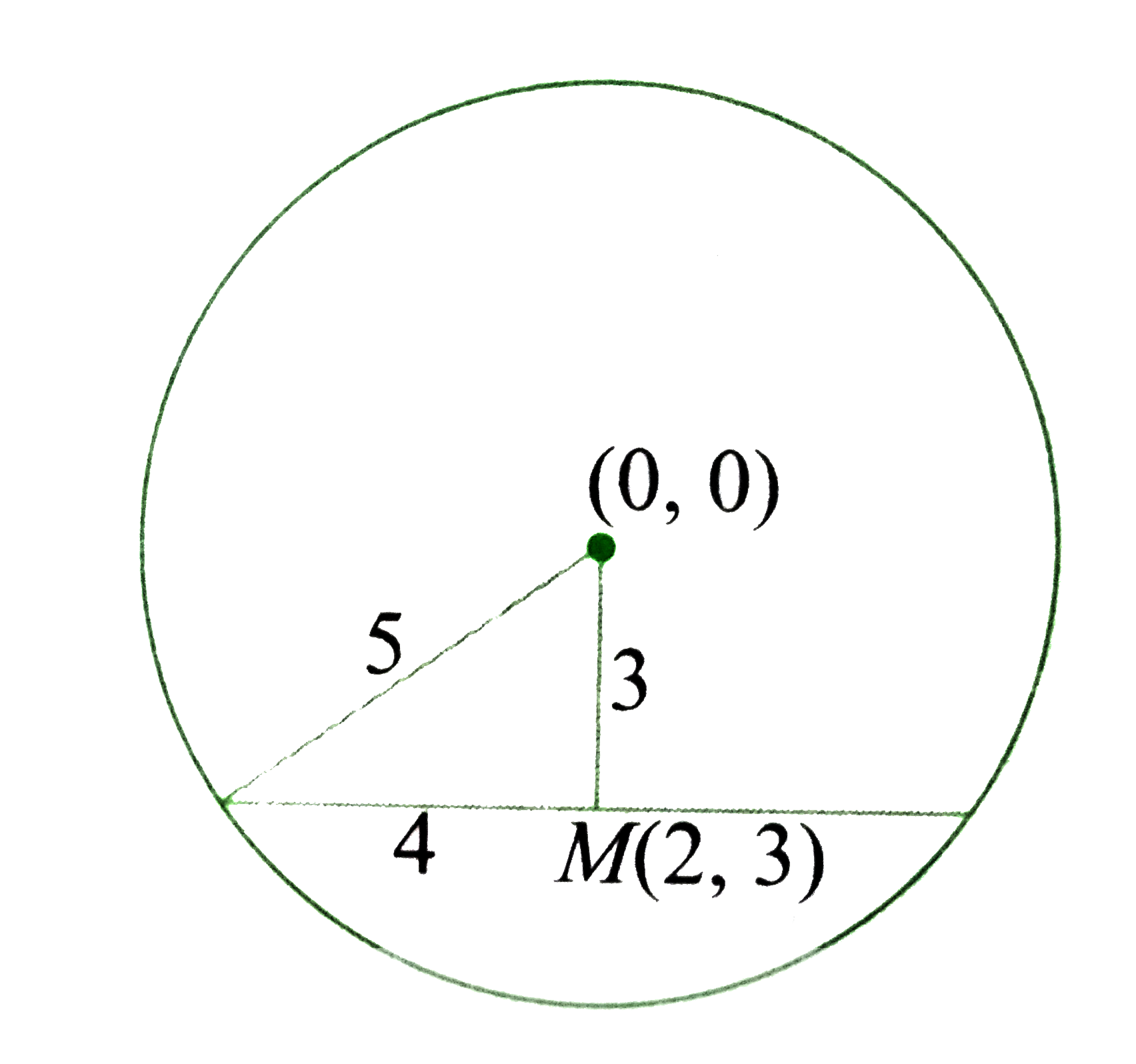
Let the slope the required line be m.
$y - 3 = m (x - 2)$
$\Rightarrow m x - y + (3 - 2 m) = 0$
Length of perpendicular from origin $= 3$
$\Rightarrow 9 + 4 m^{2} - 12 m = 9 + 9 m^{2}$
$\Rightarrow 5 m^{2} + 12 m = 0$
$\Rightarrow m = 0, - \frac{12}{5}$
Hence, lines are
$y - 3 = 0$ or $y = 3$
and $y - 3 = - \frac{12}{5} (x - 2)$ or $12 x + 5 y = 39$

|

Updated on:21/07/2023

Class 12 MATHS CIRCLE

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The two lines through (2,3) from which the circle $x^{2} + y^{2} = 25$ intercepts chords of length 8 units have equations
A $2 x + 3 y = 13, x + 5 y = 17$
B $y = 3, 12 x + 5 y = 39$
C $x = 2, 9 x - 11 y = 51$
Dnone of these
Question 2 - Select One
The equation $2 x + y = 5, x + 3 y = 5, x - 2 y = 0$ have :
Ano solution
Bone solution
Ctwo solutions
Dinfinitely many solutions
Question 3 - Select One
The equations $x + 2 y + 3 z = 1, 2 x + y + 3 x = 2, 5 x + 5 y + 9 z = 5$ have
Aunique solution
Binfinite many solution
Cinconsistent
DNone of these

CENGAGE-CIRCLE -Exercise (Multiple)

Home
Ask Doubt
Profile