The line x+2y=1 cuts the ellipse $x^{2} + 4 y^{2} = 1$ 1 at two distinct points A and B. Point C is on the ellipse such that area of triangle ABC is maximum, then find point C.

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $(- \frac{1}{\sqrt{2}}, - \frac{1}{2 \sqrt{2}})$

$\frac{9 x^{2}}{4} + 3 y^{2} = 1$ , which is required locus.

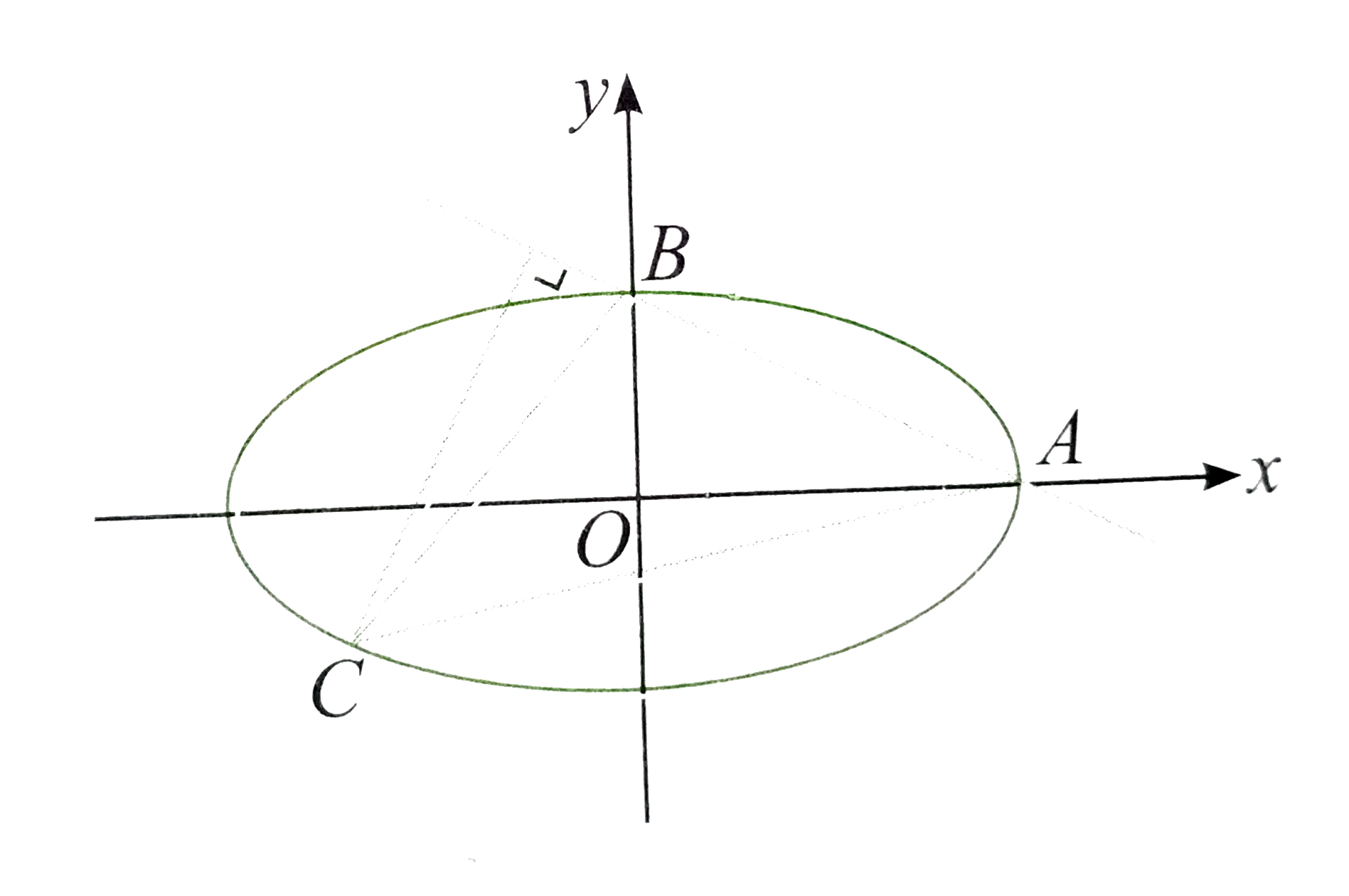
Clearly, line x+2y=1 cuts the ellipse $\frac{x^{2}}{1} + \frac{y^{2}}{1 / 4} = 1$ at $A (1, 0) and B (0, 2)$ .
Let point C on the ellipse be $(cos θ, (sin θ) / 2)$
Now, are of the triangle ABC is maximum, if distance of C from AB is maxiumm
Distance of C from AB, $d = ∣ ∣ ∣ \frac{cos θ + sin θ - 1}{\sqrt{5}} ∣ ∣ ∣$
Maximum value of d occur when $cos θ = sin θ = - \frac{1}{\sqrt{2}}$
$∴ C \equiv (- \frac{1}{\sqrt{2}}, - \frac{1}{2 \sqrt{2}})$

|

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Similar Questions

Knowledge Check

Question 1 - Select One
Two points A(1, 4) & B(3, 0) are given on the ellipse $2 x^{2} + y^{2} = 18$ . The co-ordinates of a point C on the ellipse such that the area of the triangle ABC is greatest is
A $(\sqrt{6}, \sqrt{6})$
B $(- \sqrt{6}, \sqrt{6})$
C $(\sqrt{6}, - \sqrt{6})$
D $(- \sqrt{6}, - \sqrt{6})$
Question 2 - Select One
The line $2 x + y = 3$ intersects the ellipse $4 x^{2} + y^{2} = 5$ at two points. The point of intersection of the tangents to the ellipse at these point is
A $(\frac{5}{6}, \frac{5}{6})$
B $(\frac{5}{3}, \frac{5}{6})$
C $(\frac{5}{6}, \frac{5}{3})$
D $(\frac{5}{3}, \frac{5}{3})$
Question 3 - Select One
The line $y = 2 t^{2}$ meets the ellipse $\frac{x^{2}}{9} + \frac{y^{2}}{4} = 1$ in real points if
A $| t | \leq 1$
B $| t | \geq 1$
C $| t | < 3$
DNone of these

Recommended Questions

Home
Ask Doubt
Profile