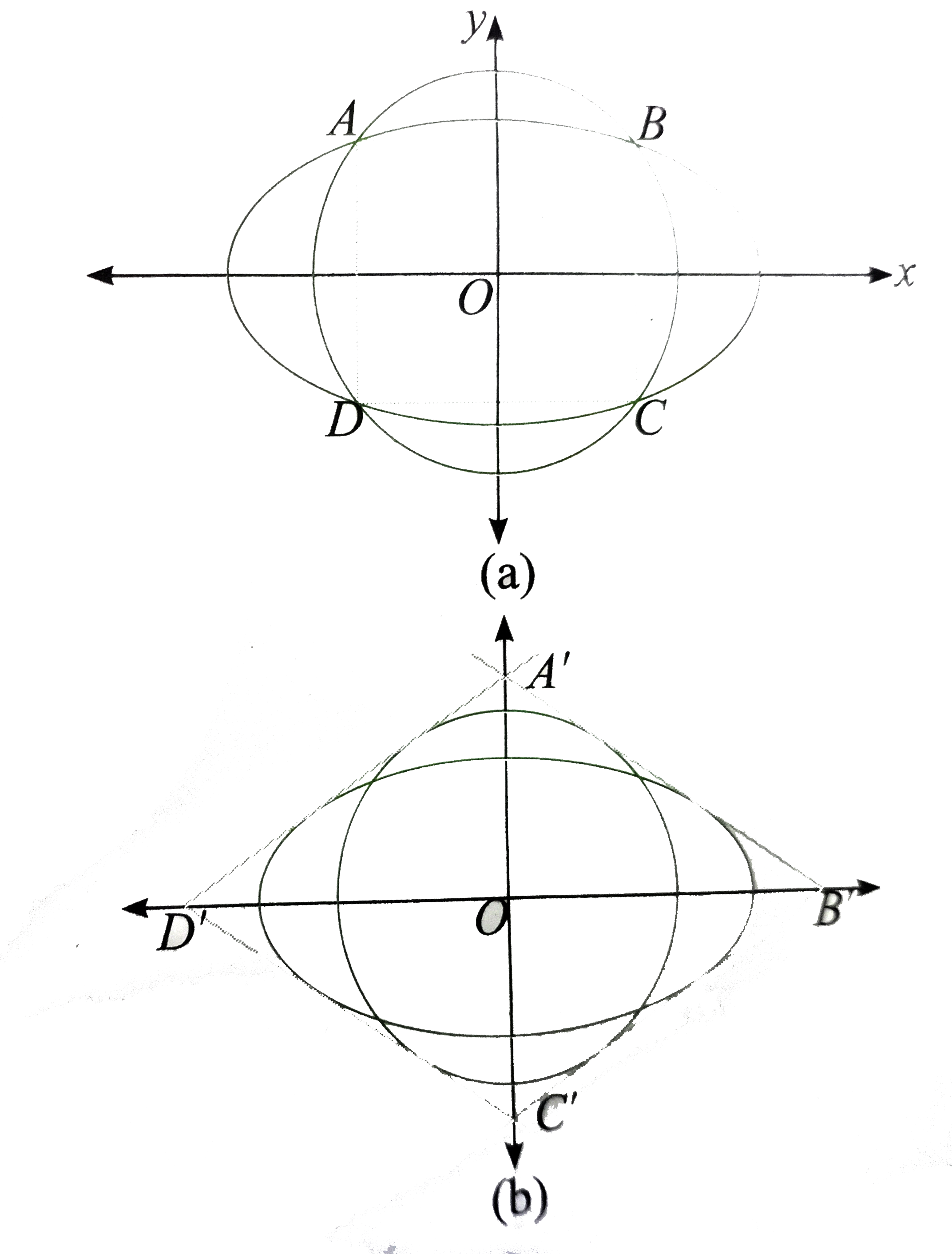
$C_{1} : x^{2} + y^{2} = r^{2} and C_{2} : \frac{x^{2}}{16} + \frac{y^{2}}{9} = 1$ interset at four distinct points A,B,C, and D. Their common tangents form a peaallelogram A'B'C'D'.
If A'B'C'D' is a square, then the ratio of the area of circle $C_{1}$ to the area of circumcircle of $Δ A' B' C'$ is

$9 / 16$

$3 / 4$

$1 / 2$

none of these

Text Solution

Verified by Experts

If A'B'C'D' is a square, then tangents are
$y = \pm x \pm 5$
for which diagonal length A'C' is 10.
Then the area of circumericle of $Δ A' B' C' is 25 π$
Also, the area of the circle $C_{1}$ is $25 / π$ . Hence the require ration is 1/2

$C_{1} : x^{2} + y^{2} = r^{2} and C_{2} : \frac{x^{2}}{16} + \frac{y^{2}}{9} = 1$ interset at four distinct points A,B,C, and D. Their common tangents form a parellelogram A'B'C'D'. if A'B'C'D' is a square, then r is equal to
A $\sqrt{120}$
B $\sqrt{12}$
C $\sqrt{15}$
Dnone of these
$C_{1} : x^{2} + y^{2} = r^{2} and C_{2} : \frac{x^{2}}{16} + \frac{y^{2}}{9} = 1$ interset at four distinct points A,B,C, and D. Their common tangents form a peaallelogram A'B'C'D'.
if A'B'C'D' is a square, then r is equal to
A $\sqrt{120}$
B $\sqrt{12}$
C $\sqrt{15}$
Dnone of these
If the points $A (- 2, - 1), B (1, 0), C (a, 3)$ and $D (1, b)$ form a parallelogram ABCD, Then the values of a and b are
A $a = - 4, b = - 2$
B $a = - 4, b = 2$
C $a = 4, b = 2$
D $a = 2, b = - 4$