The shape of a garden is rectangular in the middle and semi circular at the ends as shown in the diagram. Find the area and the perimeter of this garden [Length of rectangle is 20−(3.5+3.5) metres].
129.5,47
129.5,48
127.7,49
189.5,50
The correct Answer is:B
To solve the problem of finding the area and perimeter of the garden, which is rectangular in the middle and semi-circular at the ends, we can follow these steps:
Step 1: Understand the Dimensions
The total length of the garden is given as 20 meters. The ends of the garden are semi-circles with a combined diameter of 7 meters (3.5 meters on each side). Therefore, the length of the rectangle can be calculated as:
Length of Rectangle=20−(3.5+3.5)=20−7=13 meters
Step 2: Calculate the Area of the Rectangle
The area of a rectangle is given by the formula:
Area of Rectangle=Length×Breadth
Here, the length is 13 meters and the breadth (which is the diameter of the semi-circles) is 7 meters. Thus:
Area of Rectangle=13×7=91 square meters
Step 3: Calculate the Area of the Semi-Circles
The area of a semi-circle is given by:
Area of Semi-Circle=12πr2
The radius r of the semi-circle is half of the diameter:
r=72=3.5 meters
Now substituting the value of r:
Area of Semi-Circle=12×227×(3.5)2
Calculating (3.5)2:
(3.5)2=12.25
Now substituting back:
Area of Semi-Circle=12×227×12.25
Calculating this gives:
Area of Semi-Circle=22×12.2514=271.514=19.25 square meters
Since there are two semi-circles, the total area of the semi-circles is:
Total Area of Semi-Circles=2×19.25=38.5 square meters
Step 4: Calculate the Total Area of the Garden
Now, adding the area of the rectangle and the total area of the semi-circles:
Total Area=Area of Rectangle+Total Area of Semi-Circles
Total Area=91+38.5=129.5 square meters
Step 5: Calculate the Perimeter of the Garden
The perimeter of the garden consists of the two lengths of the rectangle and the circumference of the two semi-circles:
Perimeter=2×Length of Rectangle+Circumference of Semi-Circles
The circumference of a full circle is given by:
Circumference=2πr
Thus, for a semi-circle:
Circumference of Semi-Circle=πr
Substituting the radius:
Circumference of Semi-Circles=227×3.5=22 meters
Now substituting back into the perimeter formula:
Perimeter=2×13+22=26+22=48 meters
Final Results
- Total Area of the Garden: 129.5 square meters
- Total Perimeter of the Garden: 48 meters
The total length of the garden is given as 20 meters. The ends of the garden are semi-circles with a combined diameter of 7 meters (3.5 meters on each side). Therefore, the length of the rectangle can be calculated as:
Length of Rectangle=20−(3.5+3.5)=20−7=13 meters
The area of a rectangle is given by the formula:
Area of Rectangle=Length×Breadth
Here, the length is 13 meters and the breadth (which is the diameter of the semi-circles) is 7 meters. Thus:
Area of Rectangle=13×7=91 square meters
The area of a semi-circle is given by:
Area of Semi-Circle=12πr2
The radius r of the semi-circle is half of the diameter:
r=72=3.5 meters
Now substituting the value of r:
Area of Semi-Circle=12×227×(3.5)2
Calculating (3.5)2:
(3.5)2=12.25
Now substituting back:
Area of Semi-Circle=12×227×12.25
Calculating this gives:
Area of Semi-Circle=22×12.2514=271.514=19.25 square meters
Since there are two semi-circles, the total area of the semi-circles is:
Total Area of Semi-Circles=2×19.25=38.5 square meters
Now, adding the area of the rectangle and the total area of the semi-circles:
Total Area=Area of Rectangle+Total Area of Semi-Circles
Total Area=91+38.5=129.5 square meters
The perimeter of the garden consists of the two lengths of the rectangle and the circumference of the two semi-circles:
Perimeter=2×Length of Rectangle+Circumference of Semi-Circles
The circumference of a full circle is given by:
Circumference=2πr
Thus, for a semi-circle:
Circumference of Semi-Circle=πr
Substituting the radius:
Circumference of Semi-Circles=227×3.5=22 meters
Now substituting back into the perimeter formula:
Perimeter=2×13+22=26+22=48 meters
- Total Area of the Garden: 129.5 square meters
- Total Perimeter of the Garden: 48 meters
Topper's Solved these Questions
- MENSURATIONBOOK - NCERTCHAPTER - MENSURATIONEXERCISE - EXERCISE 11.4
8 Videos
- MENSURATIONBOOK - NCERTCHAPTER - MENSURATIONEXERCISE - EXERCISE 11.3
10 Videos
- LINEAR EQUATIONS IN ONE VARIABLEBOOK - NCERTCHAPTER - LINEAR EQUATIONS IN ONE VARIABLEEXERCISE - EXERCISE 2.3
9 Videos
- PLAYING WITH NUMBERSBOOK - NCERTCHAPTER - PLAYING WITH NUMBERSEXERCISE - SOLVED EXAMPLES
8 Videos
Similar Questions
A field has the shape of a rectangle having a semi-circle at its one end as shown in the figure. Find the area of the field.
View SolutionA rectangular' sheet is cut along its length into five equal squares as shown in the figure. The area of a square is 16cm2. Find the perimeter of the rectangle.

View Solution
Knowledge Check
The shape of a garden is rectangular in the middle and semi circular at the ends as shown in the diagram. Find the area and the perimeter of this garden [Length of rectangle is 20−(3.5+3.5) metres].
A129.5,47B129.5,48C127.7,49D189.5,50A garden is in the form of a rectangle with semi-circular ends on the either side as shown in the diagram below. The length and breadth of the rectangle are 20 m and 14 m, respectively. The cost of leveling the plot at 25 per m2 is
 ARs. 10850BRs. 434CRs. 25DNone of these
ARs. 10850BRs. 434CRs. 25DNone of these
Find area and perimeter of rectangle with length 5 cm and breadth 6 cm
View SolutionFind the area of the flower bed (with semi-circular ends) shown in figure

View SolutionA rectangular flower garden 20m by 15m has a 3m wide path running in the middle. Find the area of the path.
View SolutionFind the area of the flower bed (with semi-circular ends) shown in figure.
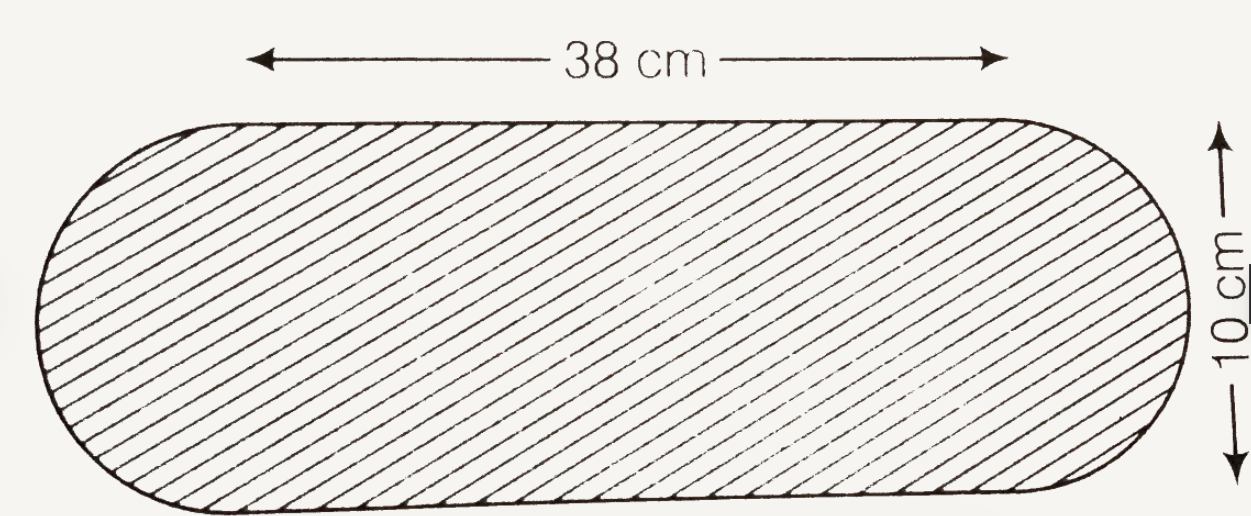
View SolutionThe perimeter of a rectangle is 40 m. Its length is four metres less than five times its breadth. Find the area of the rectangle.
View SolutionFind the perimeter of a rectangle in which Length = 10 cm and Breadth = 3.5 cm
View Solution
