Find the coefficient of $x^{5}$ in the expansioin of the product ${(1 + 2 x)}^{6} {(1 - x)}^{7} .$

Video Solution

Text Solution

Verified by Experts

We know that

${(a + b)}^{n} =^{n} C_{0} a^{n} +^{n} C_{1} a^{n - 1} b^{1} + ... ... . . +^{n} C_{n - 1} a^{1} b^{n - 1} +^{n} C_{n} b^{n}$

Hence,
${(a + b)}^{6} =^{6} C_{0} a^{6} +^{6} C_{1} a^{5} b^{1} +^{6} C_{2} a^{4} b^{2} +^{6} C_{3} a^{3} b^{3} +^{6} C_{4} a^{2} b^{4} +^{6} C_{5} a^{1} b^{5} +^{6} C_{6} b^{6}$

$= a^{6} + \frac{6!}{1! (6 - 1)!} a^{5} b^{1} + \frac{6!}{2! (6 - 2)!} a^{4} b^{2} + \frac{6!}{3! (6 - 3)!} a^{3} b^{3} + \frac{6!}{4! (6 - 4)!} a^{2} b^{4} + \frac{6!}{5! (6 - 5)!} a^{1} b^{5} + b^{6}$

$= a^{6} + \frac{6!}{1 \times 5!} a^{5} b^{1} + \frac{6!}{2! \times 4!} a^{4} b^{2} + \frac{6!}{3! 3!} a^{3} b^{3} + \frac{6!}{4! 2!} a^{2} b^{4} + \frac{6!}{5! \times 1} a^{1} b^{5} + b^{6}$

$= a^{6} + \frac{6 \times 5!}{5!} a^{5} b^{1} + \frac{6 \times 5 \times 4!}{2 \times 4!} a^{4} b^{2} + \frac{6 \times 5 \times 4 \times 3!}{3 \times 2 \times 1 \times 3!} a^{3} b^{3} + \frac{6 \times 5 \times 4!}{4! \times 2 \times 1} a^{2} b^{4} + \frac{6 \times 5!}{5!} a^{1} b^{5} + b^{6}$

$= a^{6} + 6 a^{5} b + 15 a^{4} b^{2} + 20 a^{3} b^{3} + 15 a^{2} b^{4} + 6 a b^{5} + b^{6}$

Putting $a = 1$ & $b = 2 x$

${(1 + 2 x)}^{6} = 1^{6} + 6 {(1)}^{5} (2 x) + 15 {(1)}^{4} {(2 x)}^{2} + 20 {(1)}^{3} {(2 x)}^{3} + 15 {(1)}^{2} {(2 x)}^{4} + 6 (1) {(2 x)}^{5} + {(2 x)}^{6}$

$= 1 + 6 (2 x) + 15 (4 x^{2}) + 20 (8 x^{3}) + 15 (16 x^{4}) + 6 (32 x^{5}) + 64 x^{6}$

$= 1 + 12 x + 60 x^{2} + 160 x^{3} + 240 x^{4} + 192 x^{5} + 64 x^{6}$

Similarly,

We know that

${(a + b)}^{n} =^{n} C_{0} a^{n} +^{n} C_{1} a^{n - 1} b^{1} + ... ... . . +^{n} C_{n - 1} a^{1} b^{n - 1} +^{n} C_{n} b^{n}$

Hence,
${(a + b)}^{7} =^{7} C_{0} a^{7} +^{7} C_{1} a^{6} b^{1} +^{7} C_{2} a^{5} b^{2} +^{7} C_{3} a^{4} b^{3} +^{7} C_{4} a^{3} b^{4} +^{7} C_{5} a^{2} b^{5} +^{7} C_{5} a^{1} b^{6} +^{7} C_{6} b^{7}$

$= a^{7} + \frac{7!}{1! (7 - 1)!} a^{6} b^{1} + \frac{7!}{2! (7 - 2)!} a^{5} b^{2} + \frac{7!}{3! (7 - 3)!} a^{4} b^{3} + \frac{7!}{4! (7 - 4)!} a^{3} b^{4} + \frac{7!}{5! (7 - 5)!} a^{2} b^{5} + \frac{7!}{5! (7 - 6)!} a^{1} b^{6} + b^{7}$

$= a^{7} + \frac{7!}{1 \times 6!} a^{6} b^{1} + \frac{7!}{2! \times 5!} a^{5} b^{2} + \frac{7!}{3! 4!} a^{4} b^{3} + \frac{7!}{4! 3!} a^{3} b^{4} + \frac{7!}{5! \times 2!} a^{2} b^{5} + \frac{7!}{6! \times 1!} a^{1} b^{6} + b^{7}$

$= a^{7} + \frac{7 \times 6!}{6!} a^{6} b^{1} + \frac{7 \times 6 \times 5!}{2 \times 5!} a^{5} b^{2} + \frac{7 \times 6 \times 5 \times 4!}{3 \times 2 \times 1 \times 4!} a^{4} b^{3} + \frac{7 \times 6 \times 5 \times 4!}{4! \times 3 \times 2 \times 1} a^{3} b^{4} + \frac{7 \times 6 \times 5!}{5! \times 2} a^{2} b^{5} + \frac{7 \times 6!}{6!} a^{1} b^{6} + b^{7}$

$= a^{7} + 7 a^{6} b + 21 a^{5} b^{2} + 35 a^{4} b^{3} + 35 a^{3} b^{4} + 21 a^{2} b^{5} + 7 a b^{6} + b^{7}$

Putting $a = 1$ & $b = - x$

${(1 - x)}^{7} = {(1)}^{7} + 7 {(1)}^{6} {(- x)}^{1} + 21 {(1)}^{5} {(- x)}^{2} + 35 {(1)}^{4} {(- x)}^{3} + 35 {(1)}^{3} {(- x)}^{4} + 21 {(1)}^{2} {(- x)}^{5} + 7 (1) {(- x)}^{6} + {(- x)}^{7}$

$= 1 - 7 x + 21 x^{2} - 35 x^{3} + 35 x^{4} - 21 x^{5} + 7 x^{6} - x^{7}$

Now,

${(1 + 2 x)}^{6} {(1 - x)}^{7} = (1 + 12 x + 60 x^{2} + 160 x^{3} + 240 x^{4} + 192 x^{5} + 64 x^{6}) \times (1 - 7 x + 21 x^{2} - 35 x^{3} + 35 x^{4} - 21 x^{5} + 7 x^{6} - x^{7})$

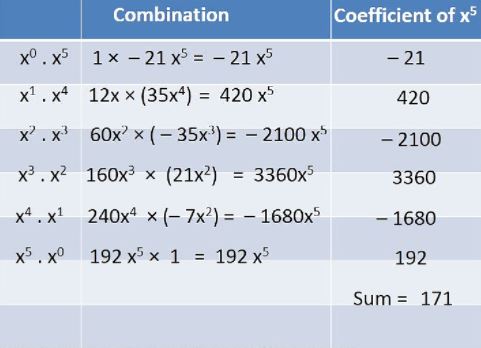
Coefficient of $x^{5}$ is $171$

Updated on:21/07/2023