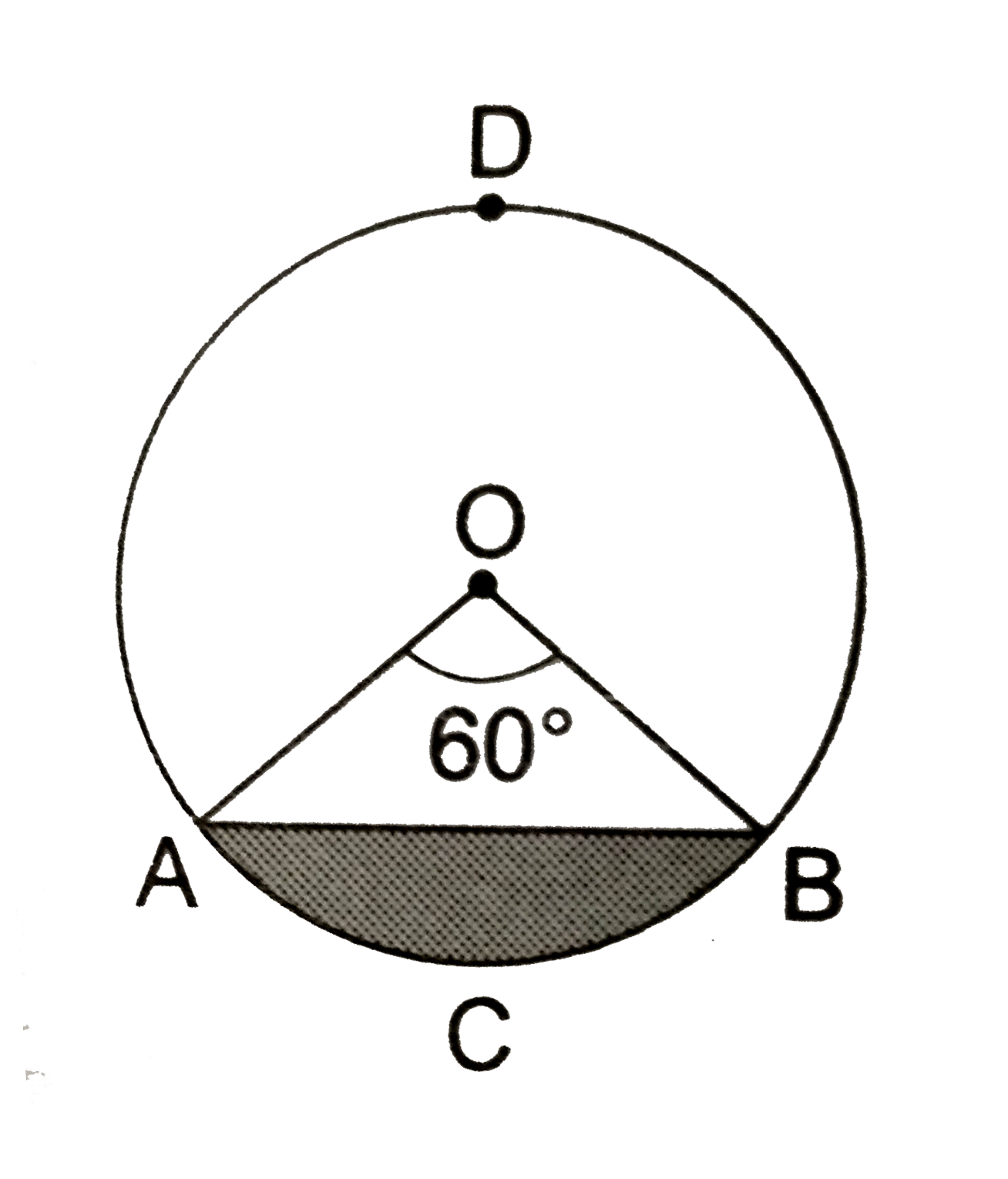
In a circle a radius 21 cm. An arc subtends an angle 60∘ at the centre (i) the length of an arc. (ii) are of the sector formed by the arc (iii) the area of the segment made by this arc.
Let ACB be th given are subtending an angle of 60∘ at the centre. Then r=21 cm and θ=60∘
(i) Length of the arc ACB=2πrθ360cm
=(2×227×21×60360)cm
=22cm
(ii) Area of the sector OACBO=πr2θ360cm2
=(227×21×21×60360)cm2
=231cm2
(iii) Area of the minor segment ACBA
= (area of the sector OACB) − (area of the ΔOAB)
=(231−12r2sinθ)cm2[231−(12×21×21×sin60∘)]cm2
=(231−12×21×21×√32)cm2
=(231−14×441×1.72)cm2
=(231−189.63)cm2=41.37cm2
(iv) Area of the major segment BDAB
= (area of the circle) − (area of the minor segment)
={(227×21×21)−41.37}cm2
(1386−41.37)cm2=1344.63cm2
Topper's Solved these Questions
- AREA OF CIRCLE, SECTOR AND SEGMENT BOOK - RS AGGARWALCHAPTER - AREA OF CIRCLE, SECTOR AND SEGMENT EXERCISE - SOLVED EXAMPLE_TYPE
1 Videos
- AREA OF CIRCLE, SECTOR AND SEGMENT BOOK - RS AGGARWALCHAPTER - AREA OF CIRCLE, SECTOR AND SEGMENT EXERCISE - Exercise 16A
65 Videos
- ARITHMETIC PROGRESSIONBOOK - RS AGGARWALCHAPTER - ARITHMETIC PROGRESSIONEXERCISE - Multiple Choice Questions (Mcq)
30 Videos
Similar Questions
In a circle a radius 21 cm. An arc subtends an angle 60∘ at the centre (i) the length of an arc. (ii) are of the sector formed by the arc (iii) the area of the segment made by this arc.
View SolutionIn a circle of radius 21 cm, an arc subtends an angle of 60∘ at the centre. Find:
(i) the length of the arc
(ii) area of the sector formed by the arc
(iii) area of the segment formed by the corresponding chord.
View Solution
Knowledge Check
In a circle of radius 21 cm, an arc subtends an angle of 60∘ at the centre. The length of the arc is
A21 cmB22 cmC18.16 cmD23.5cmIn a circle of radius 21 cm, an arc subtends an angle of 72 at the centre. The length of the arc is
A21.6 cmB26.4 cmC13.2 cmD19.8 cm
In a circle of radius 21 cm, an arc subtends an angle of 60o at the centre. Find: (i) length of the arc (ii) area of the sector formed by the arc (iii) area of the segment formed by the corresponding chord of the arc.
View SolutionIn a circle of radius 21 cm, an arc subtends an angle of 60∘ at the centre. Find the length of the arc.
View SolutionIn a circle of radius 4 cm, an arc subtends an angle of 60∘ at the centre. Find the length of the arc.
View SolutionIn a circle of radius 28 cm, an arc subtends an angle of 108∘ at the center. Find the length of the arc.
View SolutionIn a circle of radius 21cm, an arc subtends an angle of 600 at the centre. Find (i) the length of the arc (ii) area of the sector formed by the arc. (Useπ227)
View SolutionIn a circle of radius 35 cm, an arc subtends an angle of 72o at the centre. Find the length of the arc and area of the sector.
View Solution
