Question

Verify division algorithm for the polynomials
$p\left(x\right)=x^3+x^2+2x+3\text{and}g\left(x\right)=x+2$.
Find $p(-2)$. What do you observe ?

Explanation

By long division , we have

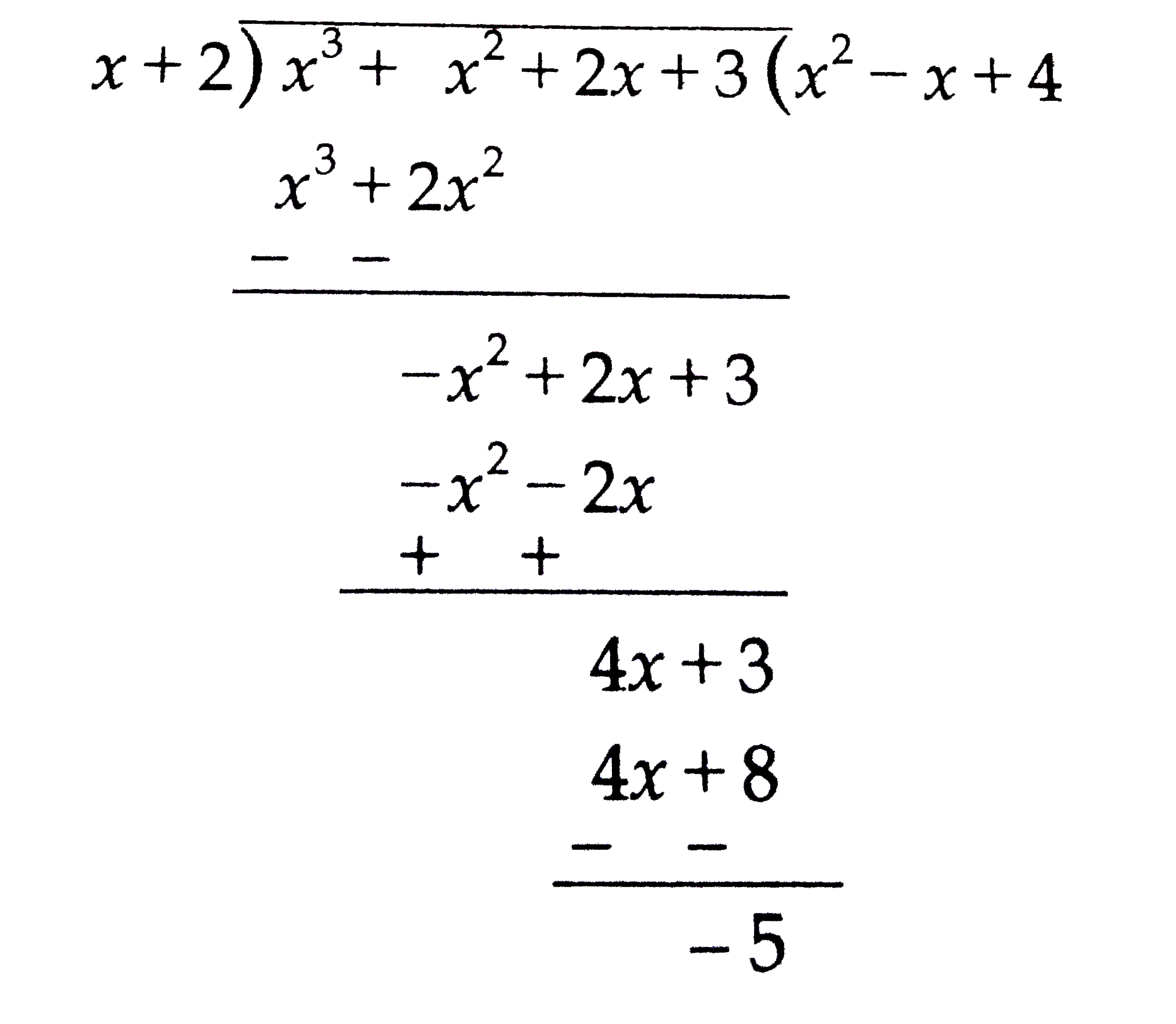
`therefore` quotient , `q(x)=x^(2)-x+4` and remainder , `r(x)=-5`.
Now `g(x)xxq(x)+r(x)=(x+2)(x^(2)-x+4)-5`
`=x^(3)+x^(2)+2x+8-5`
`=x^(3)+x^(2)+2x+3=p(x)`.
`thereforep(x)=g(x)xxq(x)+r(x)`, where `r(x)=-5` and degree `r(x)=0lt1=` degree g(x).
Thus , division algorithm is verified.
Now , `p(-2)=(-2)^(3)+(-2)^(2)+2xx(-2)+3=(-8+4-4+3)=-5`.
Observation Whan p (x) is divided by (x+2) , then the remainder is `p(-2)`.