By actual division, find the quotient and the remainder when the first polynomial is divided by the second polynomial x4+1andx−1 .
Video Solution
Text Solution
The correct Answer is:Quotient =(x3+x2+x+1) remainder =2
By actual division , we get
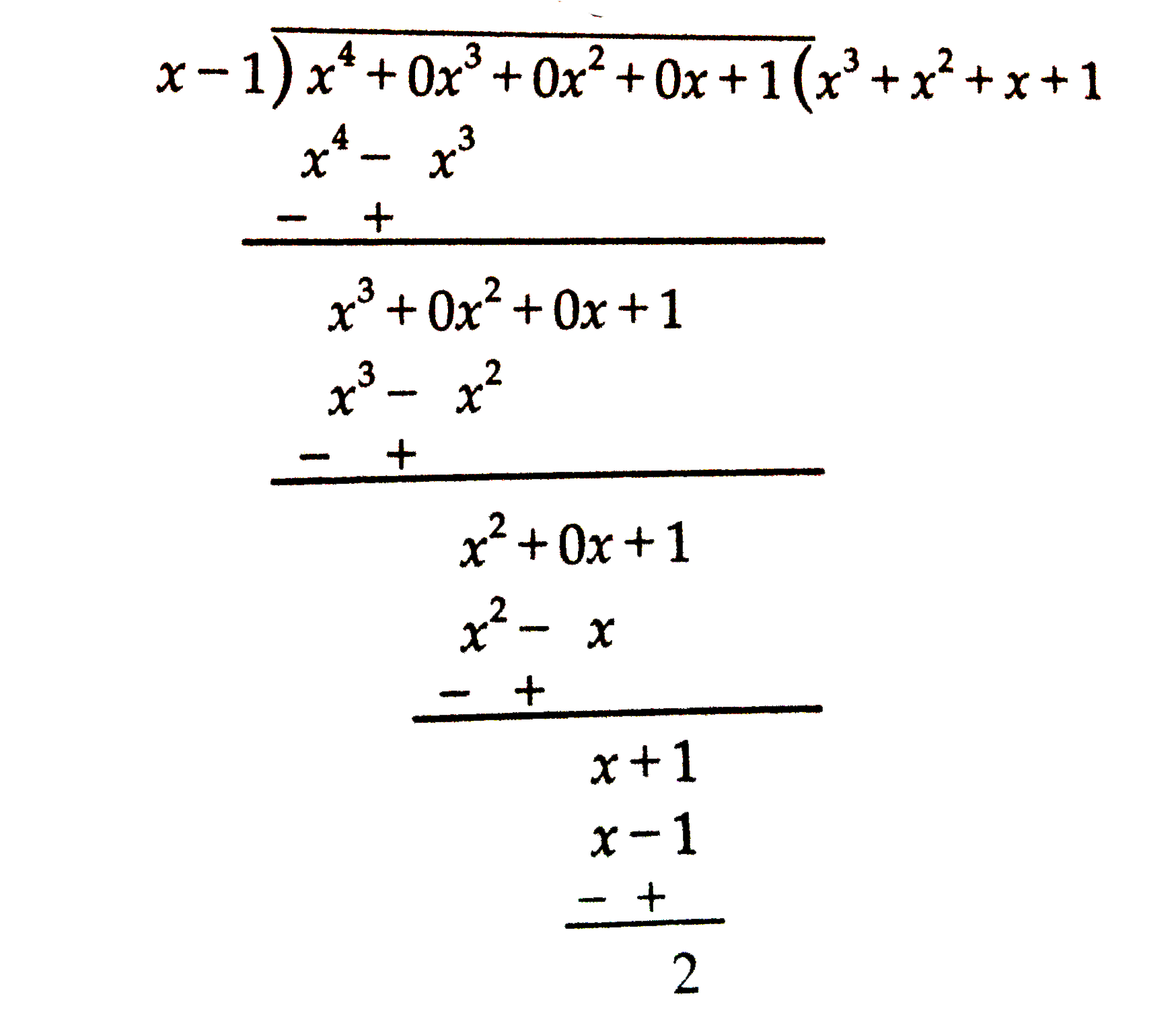
∴quotient=(x3+x2+x+1)and remainder=2.
|
Topper's Solved these Questions
- POLYNOMIALSBOOK - RS AGGARWALCHAPTER - POLYNOMIALSEXERCISE - Exercise 2D
26 Videos
- POLYNOMIALSBOOK - RS AGGARWALCHAPTER - POLYNOMIALSEXERCISE - Multiple Choice Questions (Mcq)
32 Videos
- POLYNOMIALSBOOK - RS AGGARWALCHAPTER - POLYNOMIALSEXERCISE - Exercise 2B
9 Videos
- NUMBER SYSTEMSBOOK - RS AGGARWALCHAPTER - NUMBER SYSTEMSEXERCISE - Very Short Answer Questions
23 Videos
- PRESENTATION OF DATA IN TABULAR FORMBOOK - RS AGGARWALCHAPTER - PRESENTATION OF DATA IN TABULAR FORMEXERCISE - Multiple Choice Questions (Mcq)
9 Videos
Similar Questions
Knowledge Check
Find the remainder when the polynomial f(x)=x3−3x2+4x+50 is divided by x+3 .
A−16B−12C−20D−10Find the remainder when the polynomial p(x)=x3−3x2+4x+50 is divided by (x+3).
A−13B−14C−15D−16
Find the remainder when the polynomial 4x4+3x2−5x+1 is divided by (2x-1).
View SolutionFind the remainder when the polynomial p(x)=x4−3x2+5x+1 is divided by (x-2).
View SolutionWrite the remainder when the polynomial f(x)=x3+x2−3x+2 is a divided by x+1
View SolutionFind the reminder when, p(x)=x4−3x2+2x+1 ,is divided by (x−1)
View SolutionFind the remainder when the polynomial p(x)=x100−x97+x3 is divided by x+1 .
View SolutionThe remainder obtained when the polynomial x64+x27+1is divided by x+1 is
View Solution