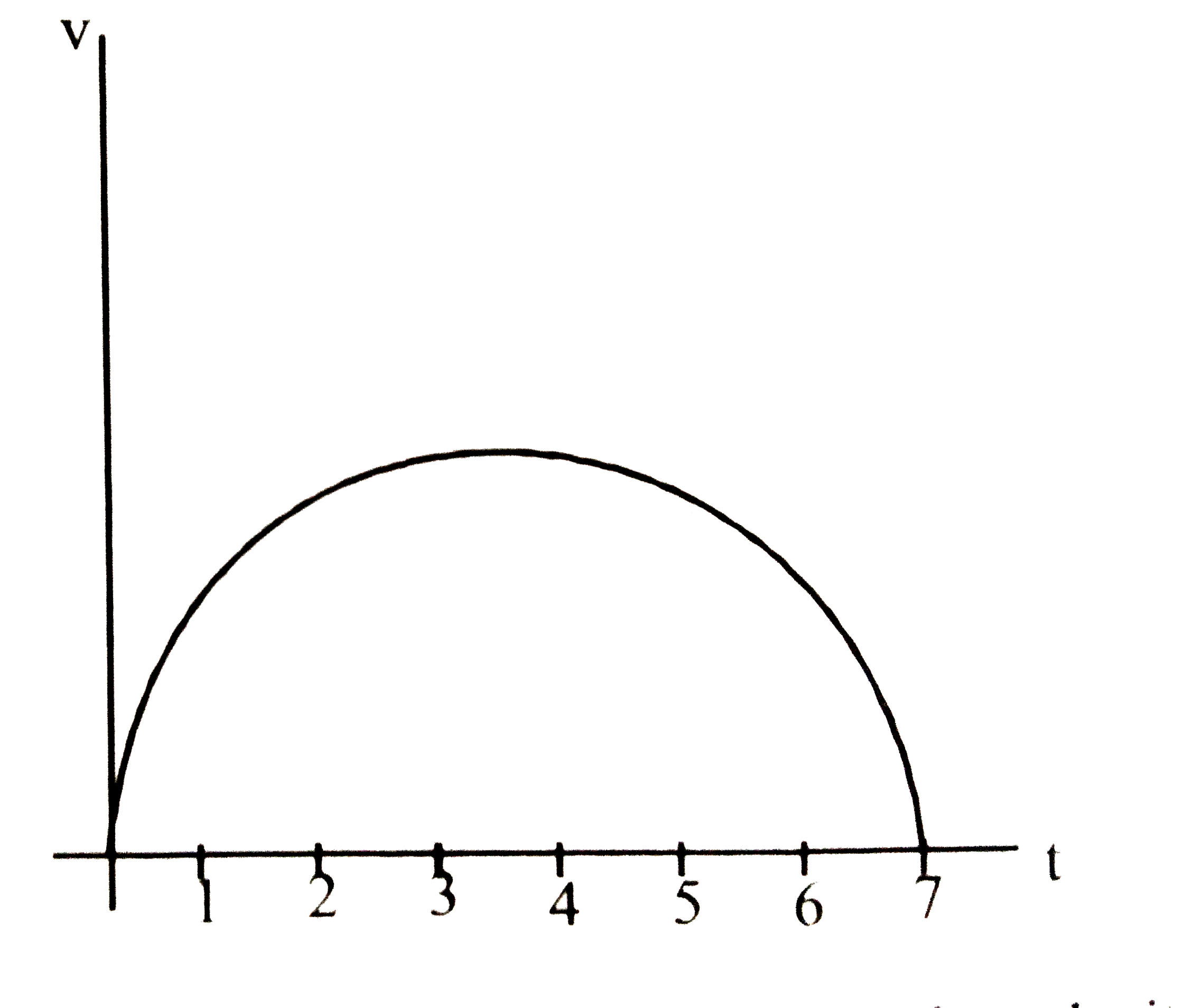
The plot given above represents the velocity of a particle (in mis) with time (in seconds). Assuming that the plot represents a semi-circle, distance traversed by the particle at the end of 7 seconds is approximately.
The plot given above represents the velocity of a particle (in mis) with time (in seconds). Assuming that the plot represents a semi-circle, distance traversed by the particle at the end of 7 seconds is approximately.

A

19 m

B

7 m

C

3.2 m

D

4.75 m

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:A

Area of the velocity-time plot (graph): represents the displacement of that object.
Radius of the semi=circle = 7 2 m
Area of the semi-circle = 1 2 π r 2
=displacement of the particle
= 1 2 × 22 7 × 7 2 × 7 2 m = 19.25 m

Updated on:21/07/2023
|
Class 12PHYSICSMechanics

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • The figure shows the velocity (v) of a particle plotted against time (t). ocr_image

    AThe particle changes its direction of motion at some point.
    BThe acceleration of the particle remains constant
    CThe displacement of the particle is zero
    DThe initial and final speeds of the particle are the same

  • The variation of velocity of a particle with time moving along a straight line is illustrated in the following figure. The distance travelled by the particle in four seconds is.
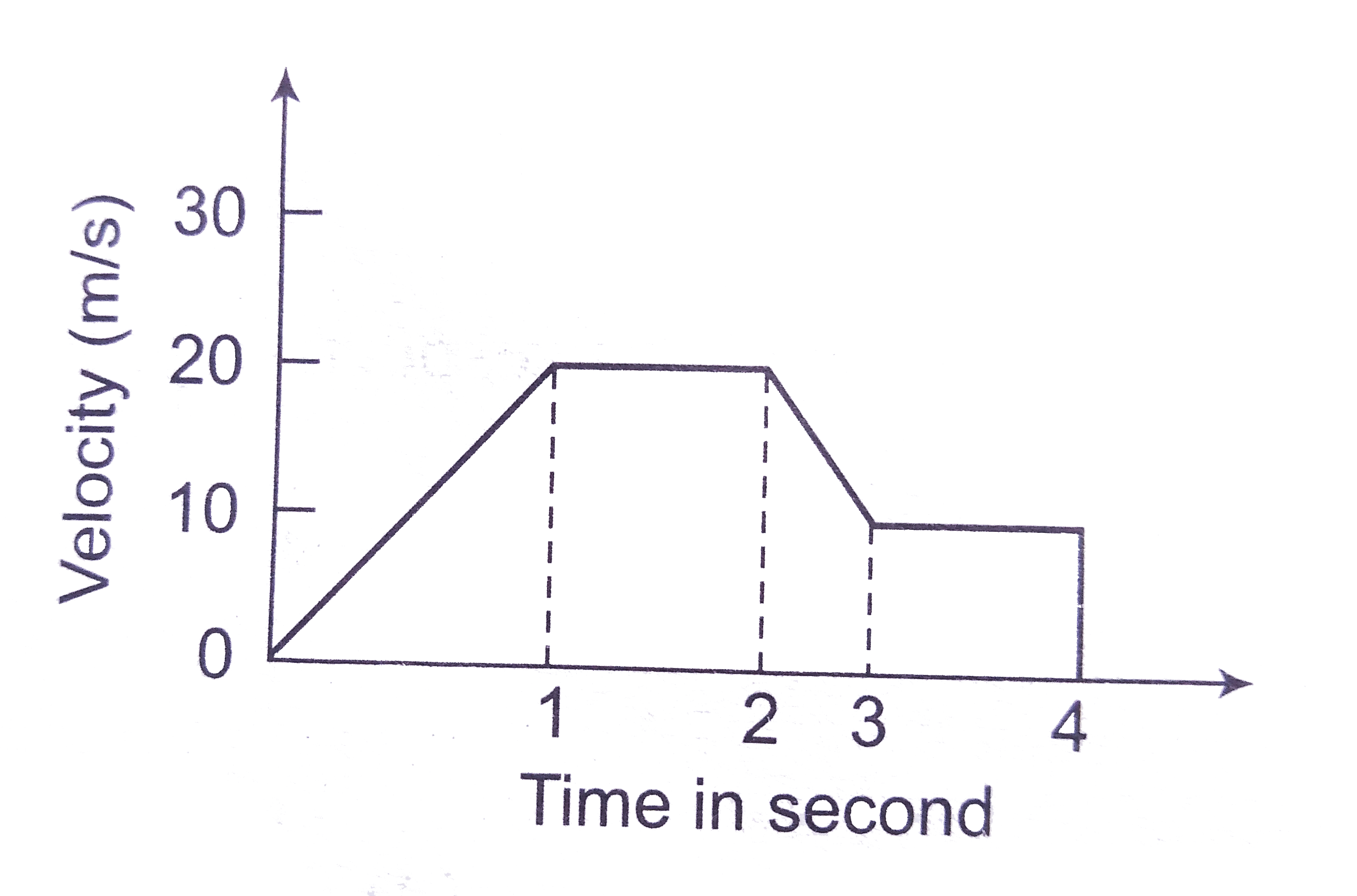    ocr_image

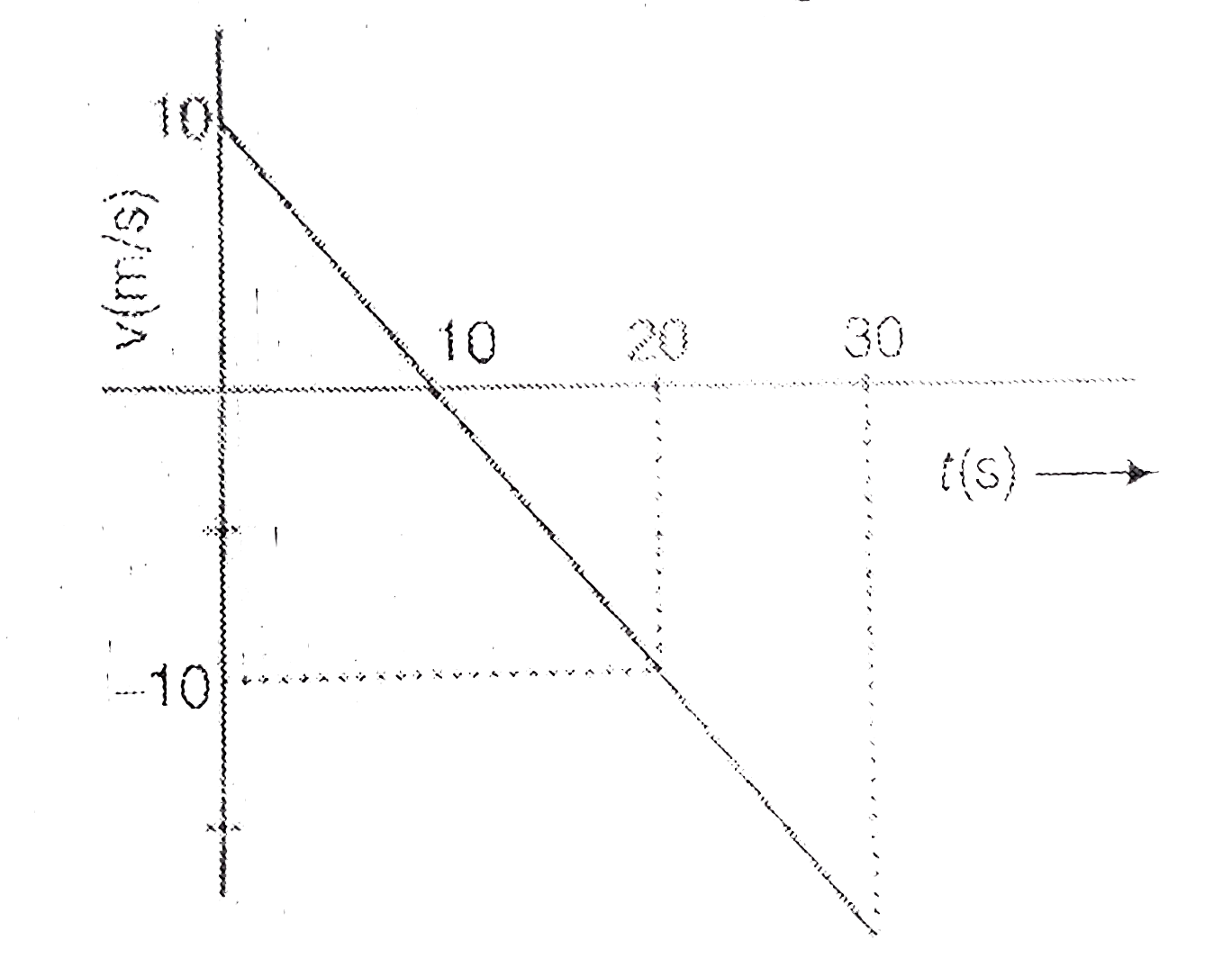
    A60 m
    B55 m
    C25 m
    D30 m

  • The velocity time plot for a particle moving on straight line is shown in the figure.
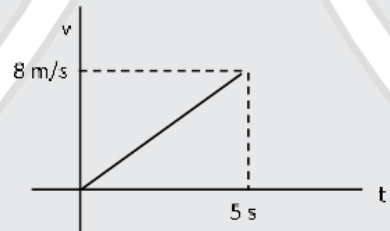
    ocr_image

    AThe particle has a constant acceleration
    BThe particle has nevedr turned around
    CThe paeticle has zero displacement.
    DThe average speed in the interval 0 to 10 s is the same as the average speed in the interval 10 s to 20s.