Doubtnut Promotions Sticky

Let f(x)=sinπx2,x>0
Let x1<x2<x3<..<xn<... be all the poitns of local maximum of f and y1<y2<y3<.....<yn<....... be all the poins of local minimum of f.
Then which of the following opions is/are correct?

A

|xn−yn|>1 for every n

B

xn+1−xn>2 for every n

C

x1<y1

D

xn∈(2n,2n+12) for every n

Text Solution
Verified by Experts

The correct Answer is:A, B, D

Given f(x)=sin(πx)x2,x>0
⇒f(x)=x2πcos(πx)−2xsin(πx)x4
=2xcos(πx)[xπ2−tan(πx)]x4
=2cos(πx)[xπ2−tan(πx)]x3
Since, for maxima and minima of f(x),f(x)=0
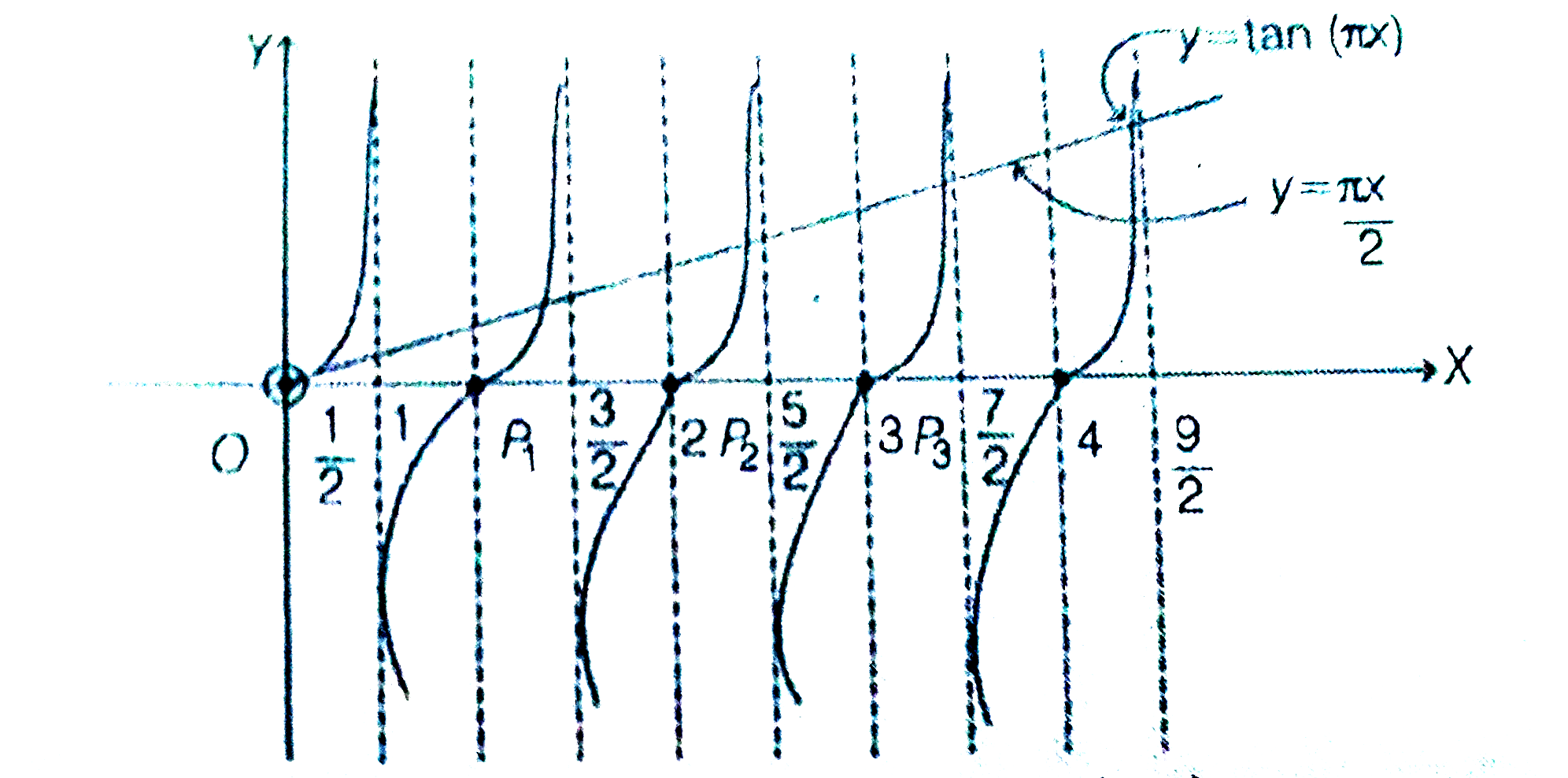
⇒cos(πx)=0 or tan(πx)=πx2, (as x>0)
∵cos(πx)≠0⇒tan(πx)=πx2
∵f'(P1')<0andf'(P1')<0⇒x=P2∈(2,52) is point of local maximum.
From the graph, for opition of maxima x1,x2,x3 . . . . it is clear that
52−x1>92−x2>132−x3>172−x4 . . .
impliexn+1−xn>2,∀n.
From the graph for point of minima y1,y2,y3. . . , it is clear
|xn−yn|>∀nandx1>(y1+1)
And x1∈(2,52),x2∈(4,92),x3∈(6,132)
⇒xn∈(2n,2n+12),∀n.
Hence, options (a), (b) and (d) is correct
ocr_image

Doubtnut Promotions Banner
|
Updated on:21/07/2023
Class 12MATHSQUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One or More

    Let f(x)=sinπxx2,x>0
    The x1<x2<x3...<xn<... be all points of local maximum of f(x) and y1<y2<y3...<yn<... be all the points of lcal minimum of f(x) then correct options is/are

    A|xn−yn|>1 for every n
    Bx1<y1
    Cxn∈(2n,2n+12) for every n
    Dxn+1−xn>2 for every n
  • Question 2 - Select One

    If 0<x<y<π ,then which one of the following is correct?

    Ax−cosx>y−cosy
    Bx−cosx<y−cosy
    Cx+cosx>ycosy
    Dx+cosx<y+cosy
  • Question 3 - Select One or More

    Let f(x)=⎧⎪⎨⎪⎩x20<x<22x−32≤x<3x+2x≥3 then the tuue equations:

    Af(f(f(32))=f(32)
    B1+f(f(f(52)))=f(52)
    Cf(f(f(2)))=f(1)
    Df(f(f(......f(4))...))=2012