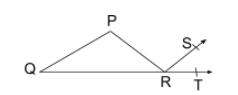
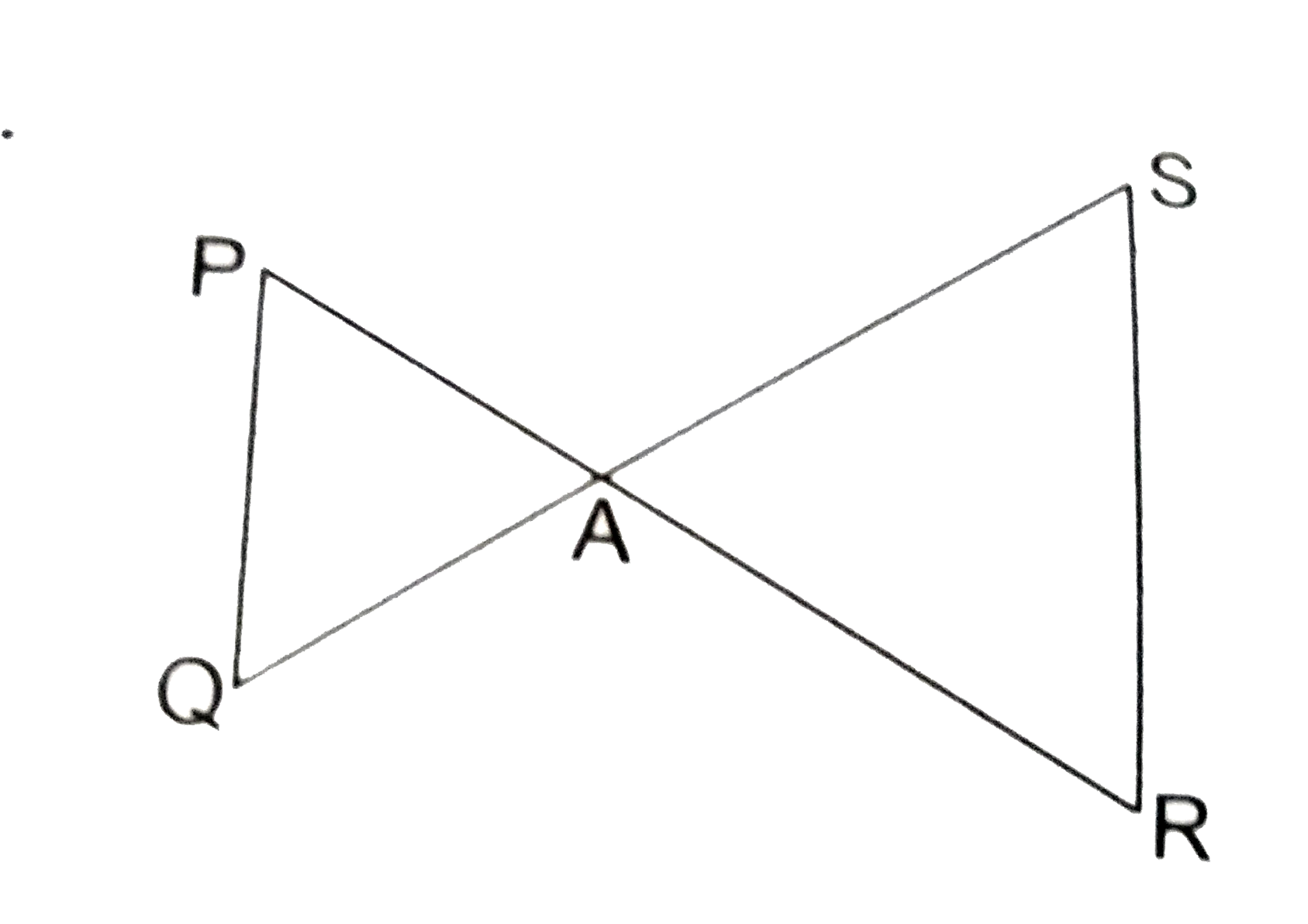
In the given figure, AP⋅AR=AS⋅AQ prove that ∠P=∠Sand∠Q=∠R.
Video Solution
Text Solution
We have,
∠PAQ=∠SAR ...(i) [ vertically opposite angles]
Also, AP⋅AR=AS⋅AQ (given)
⇒APAS=AQAR ...(ii)
From (i) and (ii) , we get
ΔPAQ~ΔSAR [ by SAS-similarity]
∴∠P=∠Sand∠Q∠R[ corresponding angles of similar triangles.]
|
Topper's Solved these Questions
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - SOLVED EXAMPLE
4 Videos
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - EXERCISE 7 A
1 Videos
- T-RATIOS OF SOME PARTICULAR ANGLESBOOK - RS AGGARWALCHAPTER - T-RATIOS OF SOME PARTICULAR ANGLESEXERCISE - Exercise 11
27 Videos
- TRIGONOMETRIC IDENTITIESBOOK - RS AGGARWALCHAPTER - TRIGONOMETRIC IDENTITIESEXERCISE - Test Yourself
20 Videos
Similar Questions
Knowledge Check
In the given figure, what is the value of ∠1+∠2+∠3+∠4+∠5+∠6+∠7+∠8+∠9+∠10 ?
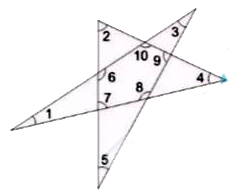 A600B720C900D1080
A600B720C900D1080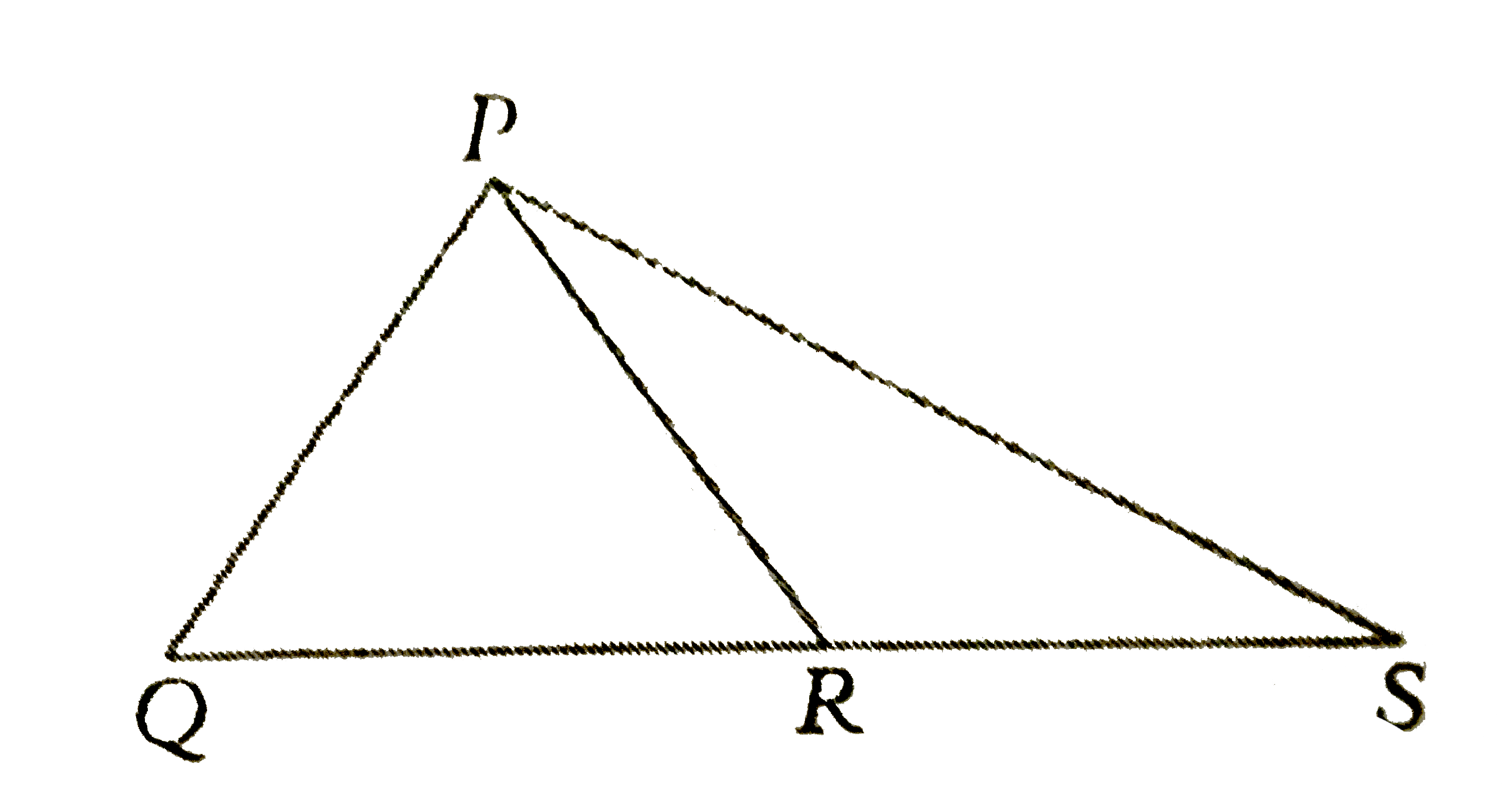
In the figure above, ∠Q=2∠Sand∠QPR=2∠RPS. Find ∠RPS+∠SA60∘B45∘C72∘D54∘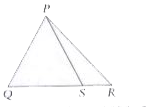
In the figure above ∠QPS=2∠SPR,∠Q=∠R+40∘and∠PSR=120∘. Find ∠QPRA50∘B55∘C65∘D60∘
In the given figure ∠1=∠2 and ∠2=∠3
then show that ∠1=∠3
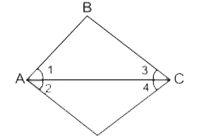
View SolutionIn the given figure we have ∠1=∠3,∠2=∠4and∠3=∠4 prove that ∠1=∠2
View SolutionIn the given figure, PR > PQ and PS bisects ∠ QPR. Prove that ∠PSR>∠PSQ.
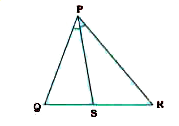
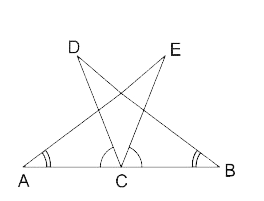
View SolutionIn the given figure , C is the midpoint of AB, if ∠DCA=∠ECBand∠DBC=∠EAC , Prove that DC=EC and BD=AE
View SolutionIn ΔDEFandΔPQR, it is given that ∠D=∠Qand∠R=∠E, then which of the following is not true?
View Solution