In the given figure, line the segment XY is parallel to side AC of ΔABC and it divides the triangles into two parts of equal area. Prove that AX:AB=(2−√2):2
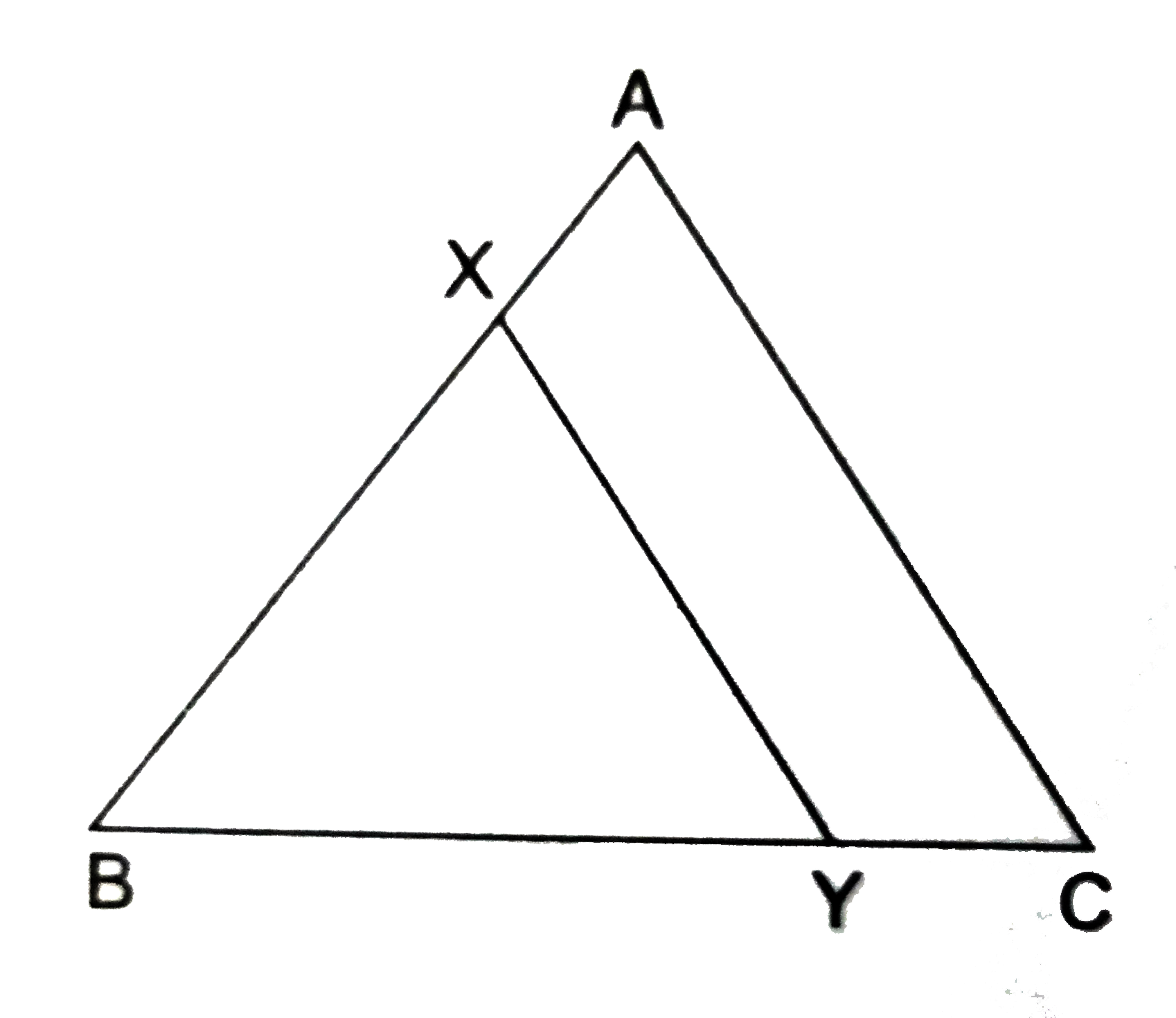
Since XY∣∣AC, we have,
∠A=∠BXYand∠C=∠BYX [ coress ∠]
∴ΔABC~ΔXBY
⇒ar(ΔABC)ar(ΔXBY)=AB2XB2....(i)
But, ar(ΔABC)~2×ar(ΔXBY) [ given]
⇒arΔABCar(ΔXYB)=2 ....(ii)
From (i) and (ii), we get
AB2XB2=2⇒(ABXB)2=2
⇒ABXB=√2AB=√2(XB)
⇒AB=√2(AB−AX)
⇒AXAB=√2(−1)√2×√2√2=(2−√2)2
Hence, AX:AB(2−√2):2
Topper's Solved these Questions
- BOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - SOLVED EXAMPLE4videos
TRIANGLES
- BOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - EXERCISE 7 A1videos
TRIANGLES
- BOOK - RS AGGARWALCHAPTER - T-RATIOS OF SOME PARTICULAR ANGLESEXERCISE - Exercise 1127videos
T-RATIOS OF SOME PARTICULAR ANGLES
- BOOK - RS AGGARWALCHAPTER - TRIGONOMETRIC IDENTITIESEXERCISE - Test Yourself20videos
TRIGONOMETRIC IDENTITIES
Similar Questions
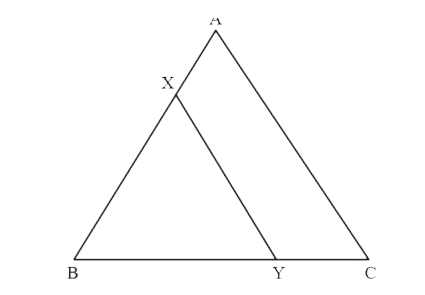
In the given figure, the line segment XY is Parallel to AC of ΔABC and it divides the triangle into two parts of equal areas. Prove that AXAB=√2−1√2
 View Solution
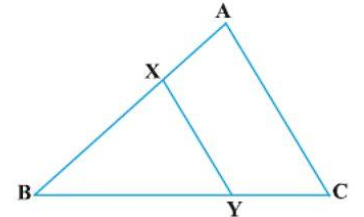
View SolutionIn Figure the line segment XY is parallel to side AC of ΔABCand it divides the triangle into two parts of equal areas. Find the ratio AXAB.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
In Figure the line segment XY is parallel to side AC of ΔABCand it divides the triangle into two parts of equal areas. Find the ratio AXAB.
A√2+1+√2B2−√2:2C1:√2D1:1 - Question 2 - Select One
In the figure given below, XY parallel to AC. If XY divides the triangle into equal Parts, then value of AX/ AB is equal to
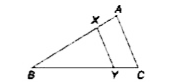 A12B1√2C√2+1√2D√2−1√2
A12B1√2C√2+1√2D√2−1√2 - Question 3 - Select One
In ΔABC, XY is parallel to BC and it divides the triangle into two equal areas (X lies on AB and Y lies on AC) then BX:AB equals
A√2:√2−1B1:1C2:1D√2−1:√2
In Fig. 4.176, XYAC and XY divides triangular region ABC into two parts equal in area. Determine AXAB.
View SolutionIn the given figure, AP is parallel to BC, BP is parallel to CQ. Prove that the areas of triangle ABC and BQP are equal
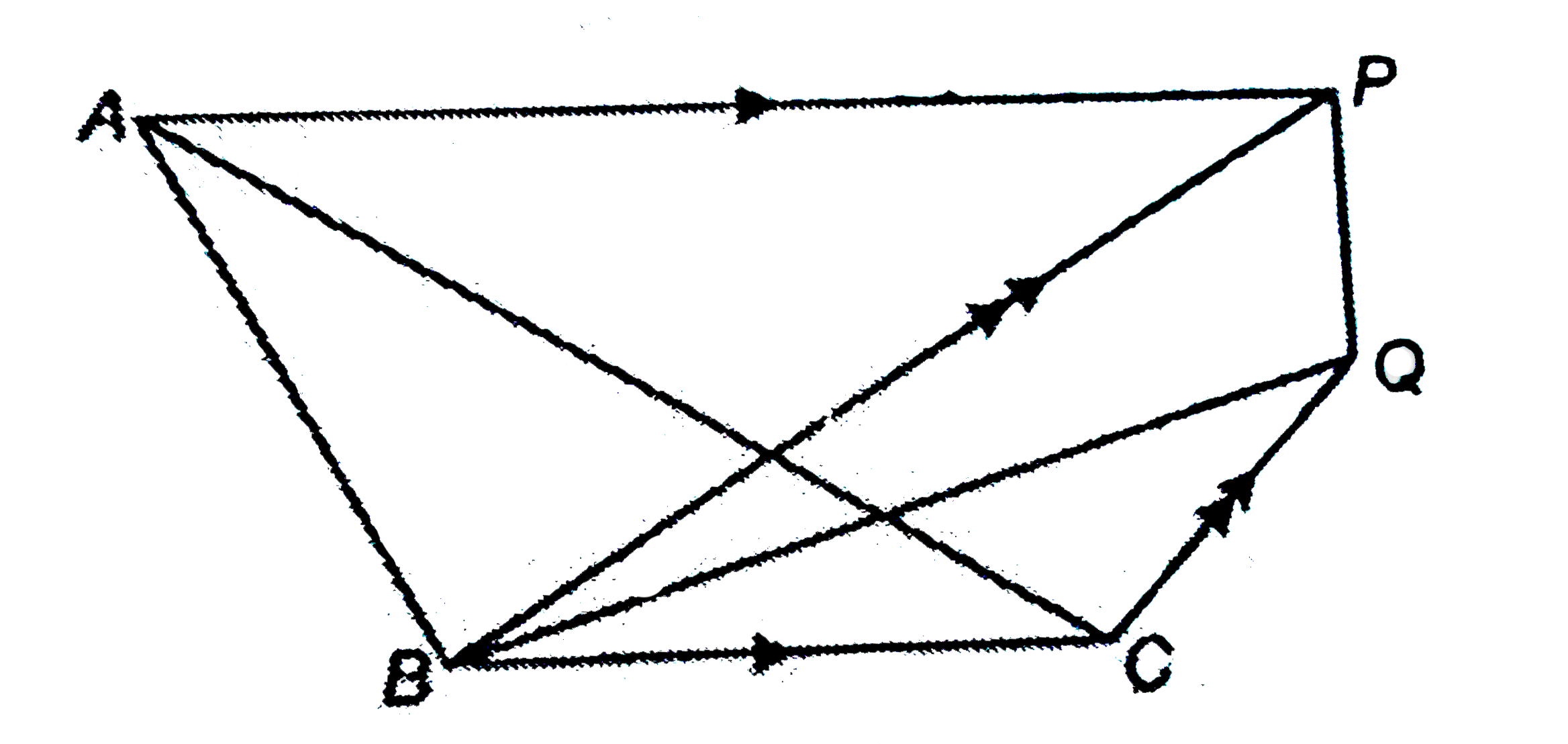 View Solution
View SolutionIn ABC , PQ is a line segment intersecting AB at P and AC at Q such that PQBC and PQ divides ABC into two parts equal in area. Find BPAB .
View SolutionX and Y are points on the sides AB and BC respectively of △ABC such that XY||AC and XY divides △ABC into two parts in area , find AXAB
View SolutionIn the given figure, XY∣∣ACandXY divides ΔABC into two regions, equal in area. Find the value of AXAB
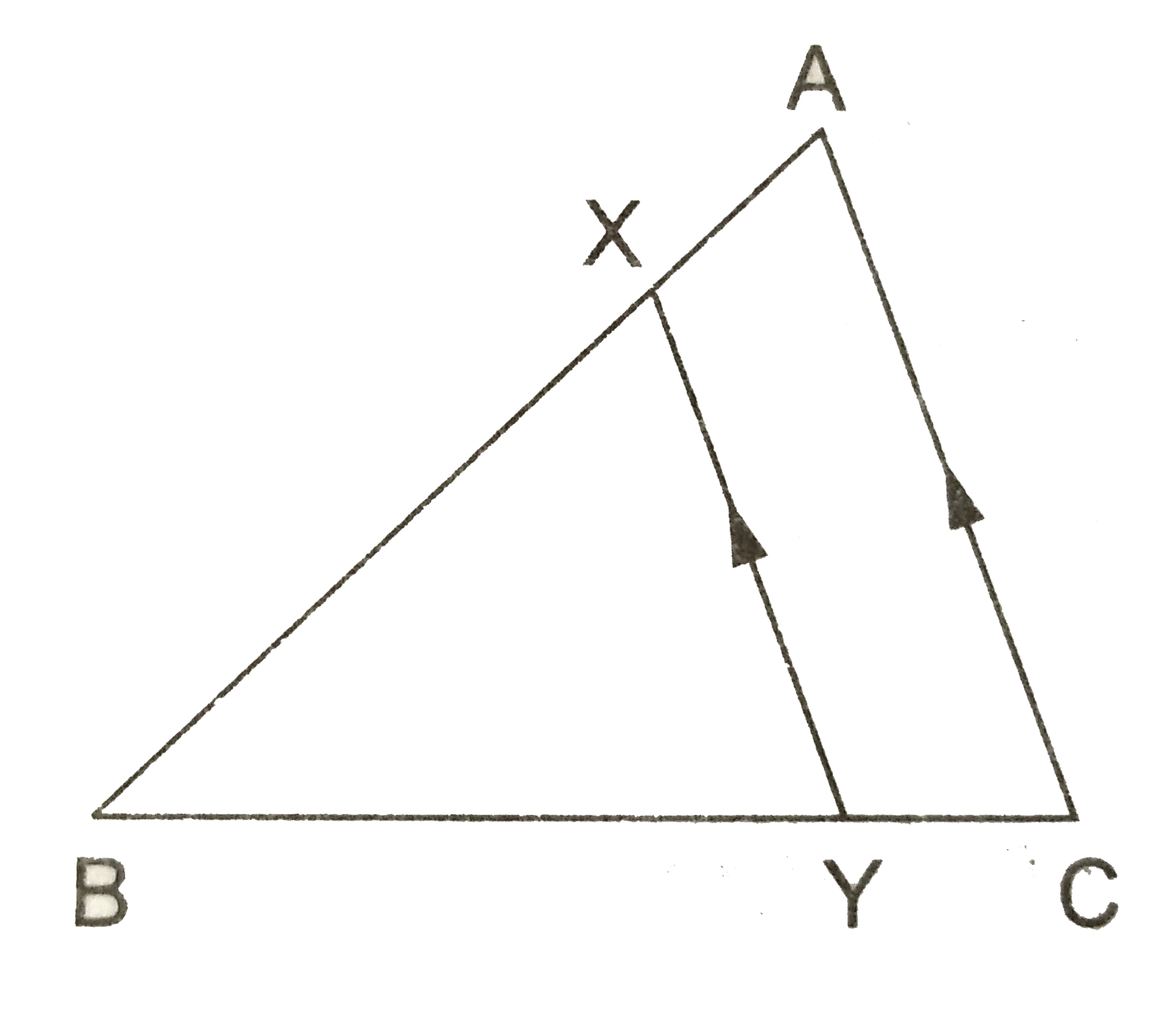 View Solution
View Solution

