Question

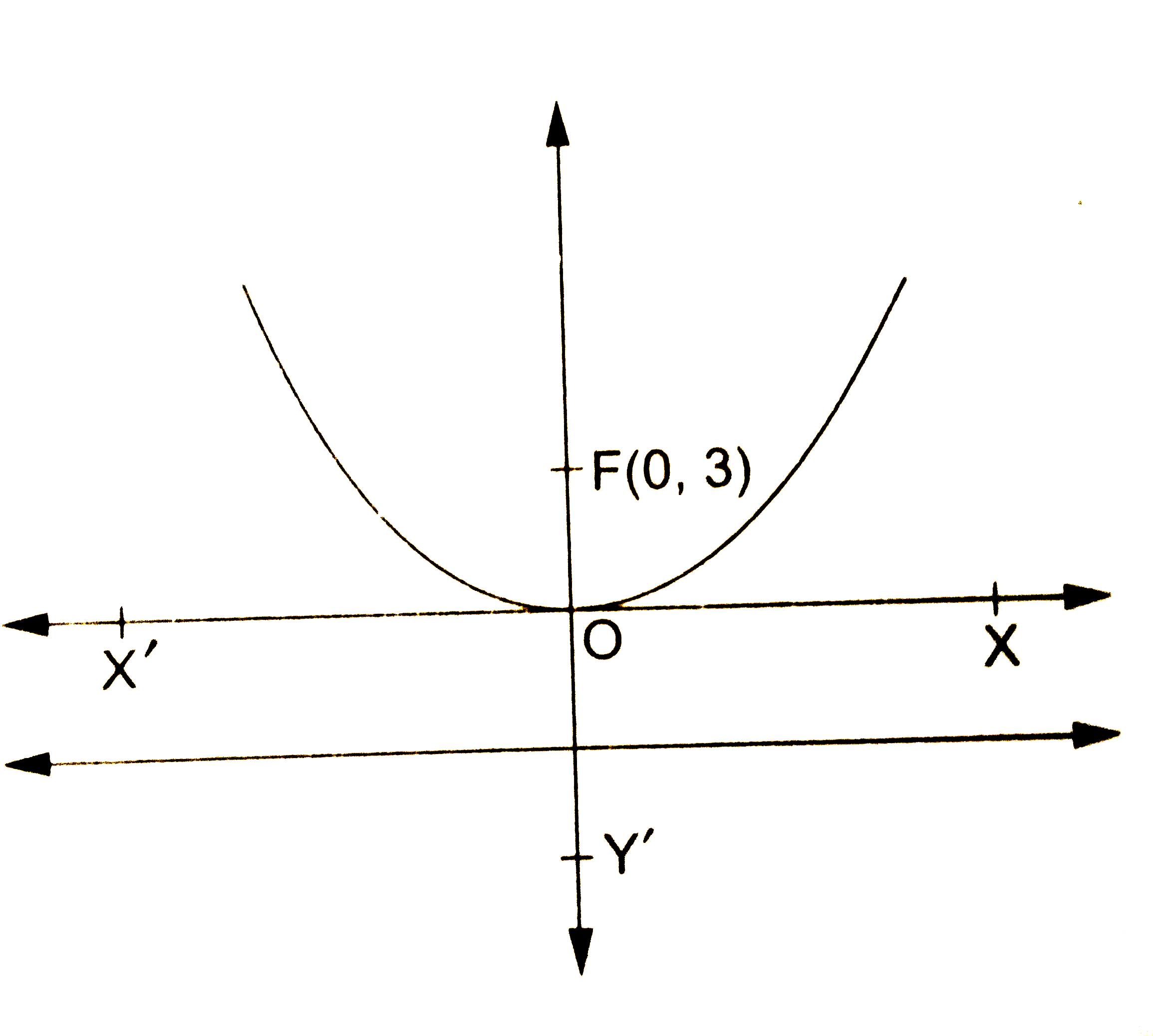
Find the equation of the parabola with vertex at the origin and y+3=0 as its directrix. Also, find its focus.

Explanation

Let the vertex of the parabola be O(0,0). Now, `y+3=0 Rightarrow y=-3`. Thus, the directix is a line parallel to the x-axis at a distance of 3 units below the x-axis. So, the focus of the parabola lies on the y-axis. Consequently, the focus is F(0,3). Hence the equations of the parabola is `x^(2)=4ay,"where"a=3`, i.e. `x^(2)=12y`