A uniform rod of length L, has a mass per unit length λ and area of cross section A. The elongation in the rod is l due to its own weight, if it suspended form the celing of a room. The Young's modulus of the rod is
2λgLAl
λgl2AL
λgL22Al
2λgL2Al
The correct Answer is:C
Increase in length of the rod due to its own weight (mg) acting at the centre of the rod will be
l=mg(L2)AY=mgL2AY or Y=mgL2Al=(λL)gL2Al=λgL22Al
Topper's Solved these Questions
- BULK PROPERTIES OF MATTERBOOK - MTG-WBJEECHAPTER - BULK PROPERTIES OF MATTEREXERCISE - WB JEE WORKOUT (ONE OR MORE THAN ONE OPTION CORRECT TYPE )
10 Videos
- BULK PROPERTIES OF MATTERBOOK - MTG-WBJEECHAPTER - BULK PROPERTIES OF MATTEREXERCISE - WB JEE PREVIOUS YEARS QUESTIONS (SINGLE OPTION CORRECT TYPE)
22 Videos
- ATOMS MOLECULES AND CHEMICAL ARITHEMETIC BOOK - MTG-WBJEECHAPTER - ATOMS MOLECULES AND CHEMICAL ARITHEMETIC EXERCISE - WB JEE PREVIOUS YEARS QUESTIONS CATEGORY 1: SINGLE OPTION CORRECT TYPE (1 MARK)
3 Videos
- CURRENT ELECTRICITY BOOK - MTG-WBJEECHAPTER - CURRENT ELECTRICITY EXERCISE - WB JEE PREVIOUS YEARS QUESTIONS
21 Videos
Similar Questions
A copper rod length L and radius r is suspended from the ceiling by one of its ends. What will be elongation of the rod due to its own weight when and Y are the density and Young's modulus of the copper respectively?
View SolutionA copper rod of length l is suspended from the ceiling by one of its ends. Find:
(a) the elongation Δl of the rod due to its own weight ,
(b) the relative increment of its volume ΔV/V.
View Solution
Knowledge Check
A uniform cylindrical rod of length L and cross-sectional area by forces as shown in figure.
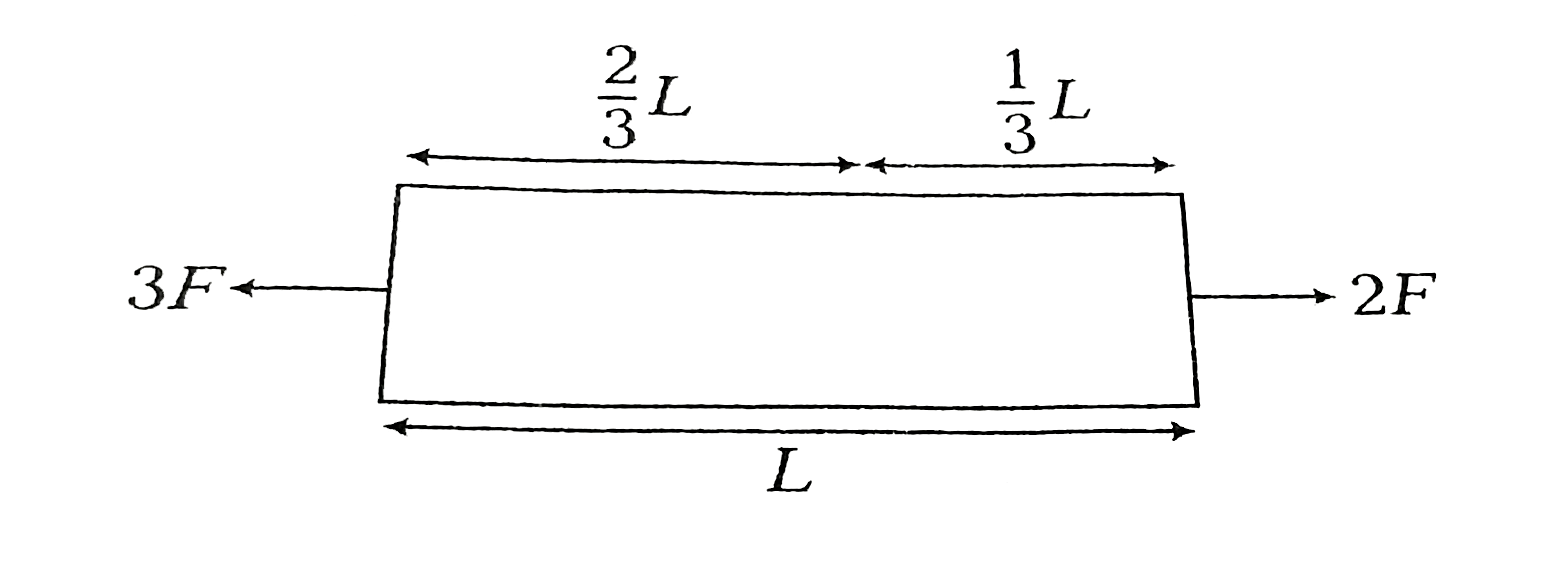
The elongation produced in the rod isA3FL8AYB3FL5AYC8FL3AYD5FL3AYYoung's modulus of a rod is AgL22l for which elongation is λ due to its own weight when suspended from the ceiling. L is the length of the rod and A is constant, which is:
AareaBmass per unit lengthCmass per unit length per unit areaDarea per unit massA uniform thin rod of length l is suspended from one of its ends and is rotated at f rotations per second. The rotational kinetic energy of the rod will be
A23π2f2ml2B43f2ml2C4π2f2ml2Dzero
wire of length I has a ear mass density λ and area of cross-section A and the Young's modulus y is suspended vertically from rigid support. The extension produced in wire due to its own weight
View SolutionA uniform thin rod of length l is suspended from one of its ends and is rotated at f rotations per second. The rotational kinetic energy of the rod will be
View SolutionA uniform rod of mass m. length L, area of cross- secticn A is rotated about an axis passing through one of its ends and perpendicular to its length with constant angular velocity o in a horizontal plane If Y is the Young's modulus of the material of rod, the increase in its length due to rotation of rod is
View SolutionA uniform wire of mass m, length L, area of cross-section A and Young's modulus Y hange from the ceiling. Its elongation under its own weight will be
View SolutionA wire of length L and area of cross-section A, is stretched by a load. The elongation produced in the wire is I. If Y is the Young's modulus of the material of the wire, then the torce corstant of the wire is
View Solution
