Let F(x) be an indefinite integral of sin2x
Statement-1: The function F(x) satisfies F(x+π)=F(x) for all real x. because
Statement-2: sin3(x+π)=sin2x for all real x.
A) Statement-1: True , statement-2 is true, Statement -2 is not a correct explanation for statement -1
c) Statement-1 is True, Statement -2 is False.
D) Statement-1 is False, Statement-2 is True.
Both the Statement are true and Statement 2 is the correct explanation of Statement 1
Both the Statement are true but Statement 2 is not the correct explanation of Statement 1
Statement 1 is true but Statement 2 is false
Statement 1 is false but Statement 2 is true
The correct Answer is:B
f(x)=∫sin2x.dx=∫1−cos2x2.dx
=12x−sin2x212+c=12x−14sin2x+c
(i) f(π+x)=12(π+x)−14sin2(π+x)
=12π+12x−12sin(2π+2x)+c
=x2−12sin2x+c=f(x)
So, statement 1 is true
(ii) sin2(π+x)=sin2x
(−sinx)2=sin2x
⇒sin2x=sin2x
So, statement 2 is true
Topper's Solved these Questions
Similar Questions
Let F(x) be an indefinite integral of sin2x.
Statement- 1: The function F(x) satisfies F(x+π)=F(x) for all real x.
Statement- 2: sin2(x+π)=sin2x for all real x.
(A) Statement I is true, Statement II is also true, Statement II is the correct explanation of Statement I.
(B)Statement I is true, Statement II is also true, Statement II is not the correct explanation of Statement I.
(C) Statement I is true, Statement II is false.
(D) Statement I is false, Statement II is ture.
View SolutionConsider the function F(x)=∫x(x−1)(x2+1)dxStatement-1: F(x) is discontinuous at x=1 ,Statement-2: Integrand of F(x) is discontinuous at x=1 (A) Statement-1 is True, Statement-2 is True, Statement-2 is a correct explanation for Statement-1. (B) Statement-1 is True, Statement-2 is True, Statement-2 is NOT a correct explanation for Statement-1. (C) Statement-1 is True, Statement-2 is False. (D) Statement-1 is False, Statement-2 is True.
View Solution
Knowledge Check
Let F(x) be an indefinite integral of sin2x Statement I The function F(x) satisfies F(x+π)=F(x) for all real x. Because Statement II sin2(x+π)=sin2x, for all real x. (A) Statement I is true, Statement II is also true,
Statement II is the correct explanation of Statement I. (B)Statement I is true, Statement II is also true,
Statement II is not the correct explanation of Statement I. (C) Statement I is true, Statement II is false. (D) Statement I is false, Statement II is ture.AStatement I is true, Statement II is also true,
Statement II is the correct explanation of Statement I.BStatement I is true, Statement II is also true,
Statement II is not the correct explanation of Statement I.CStatement I is true, Statement II is false.DStatement I is false, Statement II is ture.
Statement-1: The function F(x)=∫sin2xdx satisfies F(x+π)=F(x),∀ξnR ,Statement-2: sin2(x+π)=sin2x (A) Statement-1 is True, Statement-2 is True, Statement-2 is a correct explanation for Statement-1. (B) Statement-1 is True, Statement-2 is True, Statement-2 is NOT a correct explanation for Statement-1. (C) Statement-1 is True, Statement-2 is False. (D) Statement-1 is False, Statement-2 is True.
View SolutionLet f: R R be a continuous function defined by f(x)=1ex+2e−x . Statement-1: f(c)=13, for some c∈R . Statement-2: 0<f(x)≤12√2, for all x∈R . (1) Statement-1 is true, Statement-2 is true; Statement-2 is not the correct explanation for Statement-1 (2) Statement-1 is true, Statement-2 is false (3) Statement-1 is false, Statement-2 is true (4) Statement-1 is true, Statement-2 is true; Statement-2 is the correct explanation for Statement-1
View SolutionStatement-1: ∫sin−1xdx+∫sin−1√1−x2dx=π2x+cStatement-2: sin−1x+cos−1x=π2 (A) Statement-1 is True, Statement-2 is True, Statement-2 is a correct explanation for Statement-1. (B) Statement-1 is True, Statement-2 is True, Statement-2 is NOT a correct explanation for Statement-1. (C) Statement-1 is True, Statement-2 is False. (D) Statement-1 is False, Statement-2 is True.
View SolutionEach question has 5 choices (a) ,(b) ,(c ) (d) and (e ) out of which ONLY ONE is correct.
(a) Statement -1 is True, Statement -2 is True , Statement -2 is a correct explanation for Statement -1.
(b) Statement -1 is True, Statement -2 is True, Statement -2 is NOT a correct explanation for Statement -1.
(C ) Statement -1 is True, Statement -2 is False.
(d) Statement -1 is False, Statement -2 is True.
(e) Statement -1 and Statement -2 both are False.
Statement -1: Yield of R-Br follows the order 1∘>2∘>3∘ in Hundsdicker reaction
Statement -2: Hundsdicker reaction follows free redical mechanism.
View SolutionEach question has 5 choices (a) ,(b) ,(c ) (d) and (e ) out of which ONLY ONE is correct.
(a) Statement -1 is True, Statement -2 is True , Statement -2 is a correct explanation for Statement -1.
(b) Statement -1 is True, Statement -2 is True, Statement -2 is NOT a correct explanation for Statement -1.
(C ) Statement -1 is True, Statement -2 is False.
(d) Statement -1 is False, Statement -2 is True.
(e) Statement -1 and Statement -2 both are False.
Statement -1: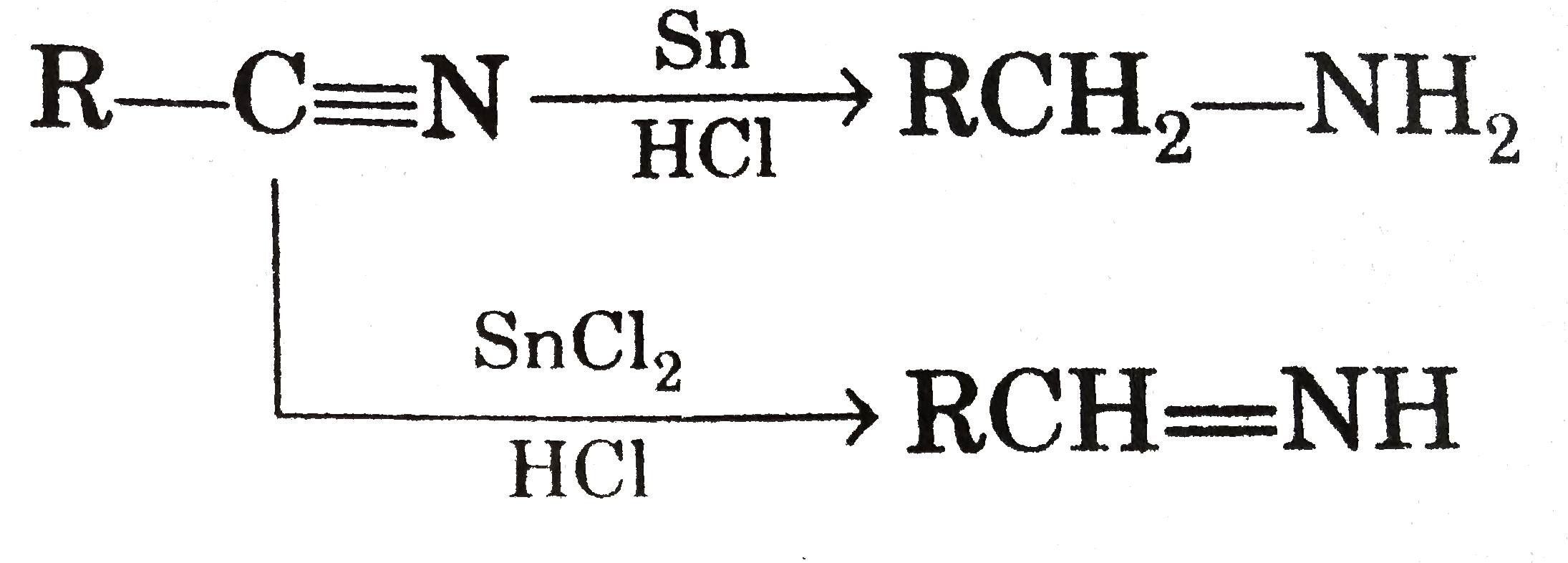
Statement-2: Sn can give maximum of 4 electron during reduction, whereas SnCl2 can give only 2.
View SolutionEach question has 5 choices (a) ,(b) ,(c ) (d) and (e ) out of which ONLY ONE is correct.
(a) Statement -1 is True, Statement -2 is True , Statement -2 is a correct explanation for Statement -1.
(b) Statement -1 is True, Statement -2 is True, Statement -2 is NOT a correct explanation for Statement -1.
(C ) Statement -1 is True, Statement -2 is False.
(d) Statement -1 is False, Statement -2 is True.
(e) Statement -1 and Statement -2 both are False.
Statement -1: Effective molecular mass of acetic acid is 120.
Statement -2: It forms dimer due to intramolecular H-bonding.
View SolutionEach question has 5 choices (a) ,(b) ,(c ) (d) and (e ) out of which ONLY ONE is correct.
(a) Statement -1 is True, Statement -2 is True , Statement -2 is a correct explanation for Statement -1.
(b) Statement -1 is True, Statement -2 is True, Statement -2 is NOT a correct explanation for Statement -1.
(C ) Statement -1 is True, Statement -2 is False.
(d) Statement -1 is False, Statement -2 is True.
(e) Statement -1 and Statement -2 both are False.
Statement -1: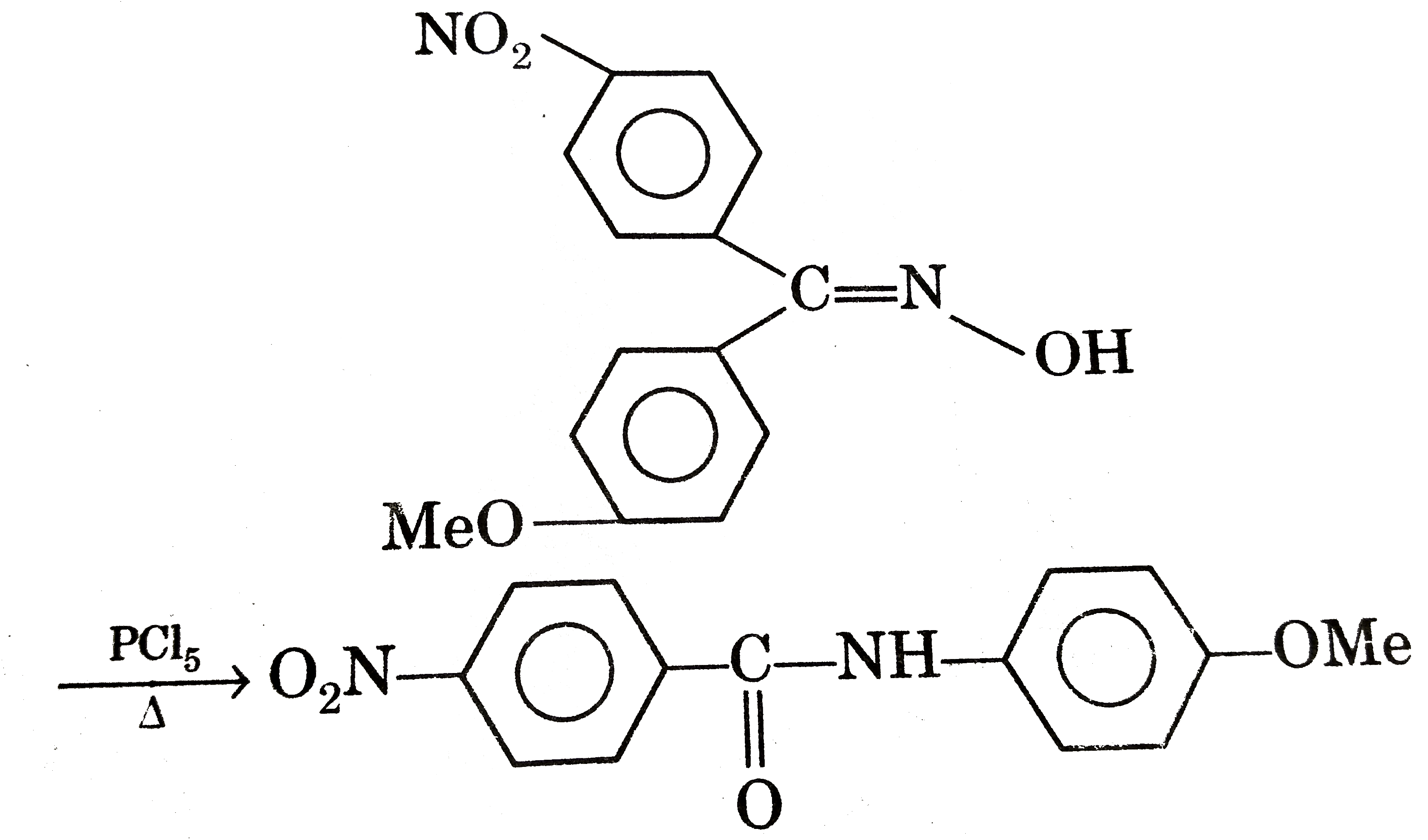
Statement -2: Migratory aptitude of p-methoxyphenyl group is greater than migratory aptitude p-nitrophenyl of group during carbrocation rearrangements.
View Solution
