In Q,NO. 111, f2orf1 is
onto but not one-one
neither continuous nor one-one
differentiable but not one-one
continuous and one-one
The correct Answer is:B
We have
f1(x)={|x|if x<0exif x≥0andf2(x)=x2
∴f20f1(x)={f2(|x|)ifx<0f2(ex)if x≥0
⇒f2of1(x)={|x|2if x<0(ex)2if x≥0
⇒f20f(1)(x)={x2if x<0e2xif x≥0

The graph, of f1 x is shown in Fig. 17. Clearly, it is not continuous at the origin and it is not one-one
Topper's Solved these Questions
- CONTINUITY AND DIFFERENTIABILITYBOOK - OBJECTIVE RD SHARMACHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Section II - Assertion Reason Type
13 Videos
- CONTINUITY AND DIFFERENTIABILITYBOOK - OBJECTIVE RD SHARMACHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Exercise
86 Videos
- CONTINUITY AND DIFFERENTIABILITYBOOK - OBJECTIVE RD SHARMACHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Exercise
86 Videos
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMACHAPTER - COMPLEX NUMBERS EXERCISE - Chapter Test
59 Videos
- DEFINITE INTEGRALSBOOK - OBJECTIVE RD SHARMACHAPTER - DEFINITE INTEGRALSEXERCISE - Chapter Test 2
60 Videos
Similar Questions
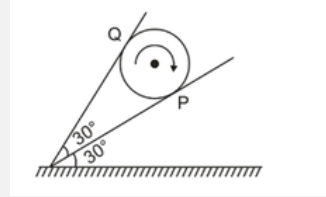
A sphere is rotating between two rough inclined walls as shown in figure. Coefficient of friction between each wall and the sphere is 1/3. If f1andf2 be the frictional forces at P and Q. Then f1f2 is
View SolutionLet f1(x)andf2(x) be twice differentiable functions where F(x)=f1(x)+f2(x)andG(x)=f1(x)−f2(x),∀x∈R,f1(0)=2andf2(0)=1.Iff'1(x)=f2(x)andf'2(x)=f1(x),∀x∈R. then the number of solutions of the equation (F(x))2=9x4G(x)is...... .
View Solution
Knowledge Check
In Q,NO, 111, f2 is
Aonto but not one-oneBneither continuous nor one-oneCdifferentiable but not one-oneDcontinuous and one-oneIn Q,NO, 111, f3 is
Aonto but not one-oneBneither continuous nor one-oneCdifferentiable but not one-oneDcontinuous and one-oneA sphere is rotating between two rough inclined walls as shoen in fiogure. Cofficent of friction between each wall and the sphere is 13. If f1 and f2 be the ffriction forces at PO and Q. Then f1f2 is
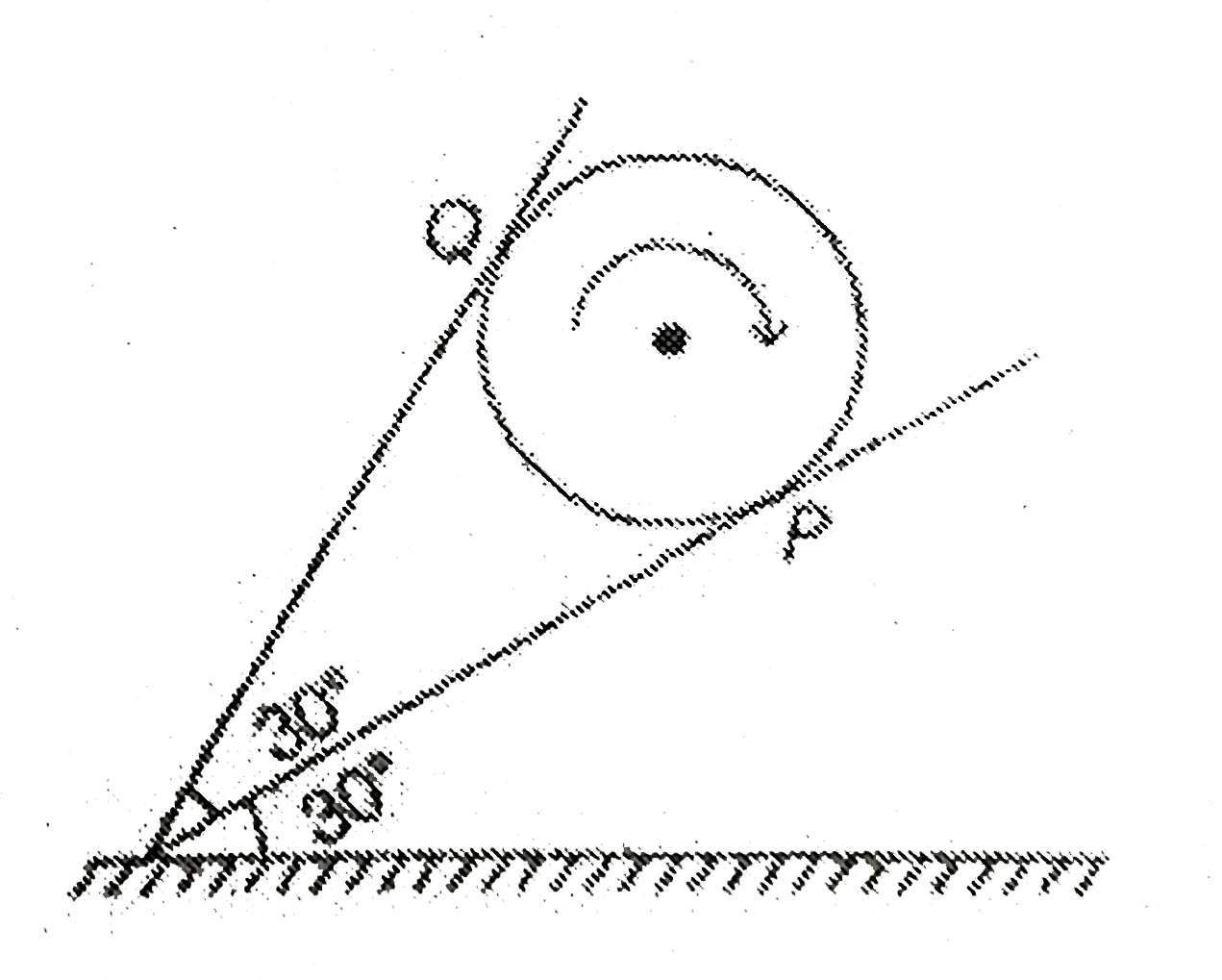 A4√3+1B1√3+2C12+√3D1+2√3
A4√3+1B1√3+2C12+√3D1+2√3
Derive the relation : 1F=1f1+1f2,where f1 and f2 are focal length of two thin lenses and F is the focal length of the combination in contact.
View SolutionFor the following table, the values of f1, f0 and f2 are respectively______.
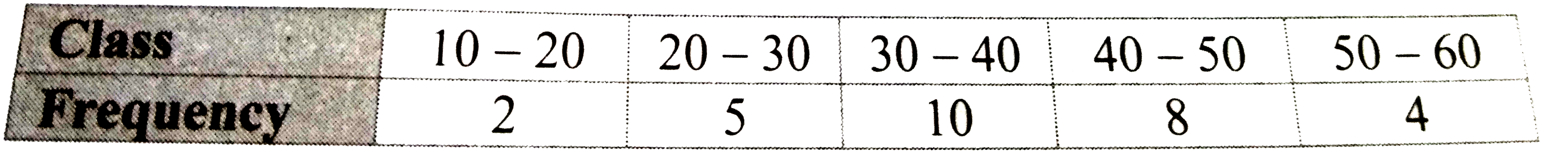
View SolutionTwo charge Q1 and Q2 are placed at the points A and B having separation r lying inside and outside the unchanged conducting shell. The force on Q1 is F and that on Q2 is f.Then
View SolutionThe value of ∫21[f1{f2(x)}]−1f'1[f2(x)]f'2(x)dx where f2(1)=f2(2), is equal to
View SolutionIf f is a function such that f(0) = 2, f(1) = 3, f(x+2) = 2f(x) - f(x + 1), then f(5) is
View Solution
