Area bounded by |x−1|≤2andx2−y2=1, is
A
6√2+12ln∣∣3+2√2∣∣
B
6√2+12ln∣∣3−2√2∣∣
C
6√2−ln∣∣3+2√2∣∣
D
none of these
Video Solution
Text Solution
The correct Answer is:C
We have
∣x−1≤2⇔−1≤3x≤3
∴ Required area A is the area of the shadded region and is given by
A=23∫1√x2−1dx
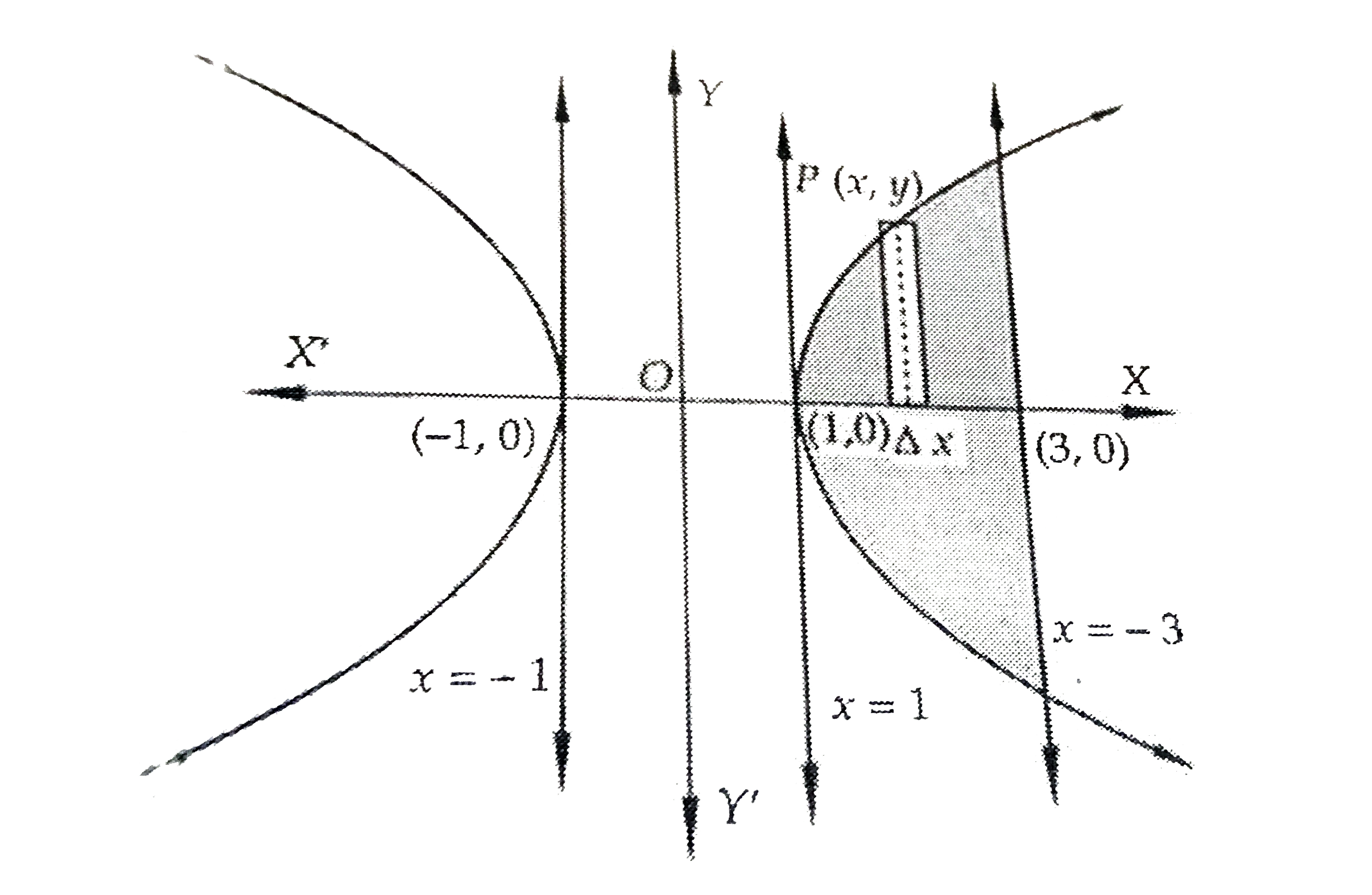
⇒A=[x√x2−a−loge(x+√x2−1)]31
⇒A={3√8−loge(3+√8)}=6√2−loge(3+2√2)
|
Topper's Solved these Questions
- AREAS OF BOUNDED REGIONSBOOK - OBJECTIVE RD SHARMACHAPTER - AREAS OF BOUNDED REGIONSEXERCISE - Exercise
62 Videos
- AREAS OF BOUNDED REGIONSBOOK - OBJECTIVE RD SHARMACHAPTER - AREAS OF BOUNDED REGIONSEXERCISE - Chapter Test
60 Videos
- APPLICATION OF DERIVATIVESBOOK - OBJECTIVE RD SHARMACHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Illustration
6 Videos
- CARTESIAN PRODUCT OF SETS AND RELATIONS BOOK - OBJECTIVE RD SHARMACHAPTER - CARTESIAN PRODUCT OF SETS AND RELATIONS EXERCISE - Chapter Test
31 Videos
Similar Questions
Knowledge Check
The area of the region bounded by y = x - 1 and x=3−y2 is
A52B72C92D4Area bounded by the circle x2+y2=1 and the curve |x|+|y|=1 is
A2πBπ−2CπDπ+3Area bounded by y=x2−2|x| and y=−1 is equal to
A21∫0(2x−x2)dxB23 sq. unitsC23 (Area of rectangle ABCD) where points A,B,C,D are (−1,−1),(−1,0),(1,0)&(1,−1)D23 (Area of rectangle ABCD) where points A,B,C,D are (−1,−1),(−1,0),(1,0)&(1,−1)
The ratio in which the area bounded by the curves y2=xandx2=y is divided by the line x=12 is
View SolutionThe area of region bounded by circles x2+y2=1 and (x−1)2+y2=1 is :
View SolutionThe area bounded by the ellipse x24+y225=1 is
View SolutionThe area of the smaller region bounded by the circle x2+y2=1 and the lines |y|=x+1 is
View SolutionThe area bounded by |x|=1−y2and|x|+|y|=1 is:
View Solution
