If the line x=a bisects the area under the curve y=1x2,1≤x≤9 , then a is equal to
A
49
B
95
C
59
D
94
Video Solution
Text Solution
The correct Answer is:B
It is given that
2a∫11x2dx=9∫11x2dx
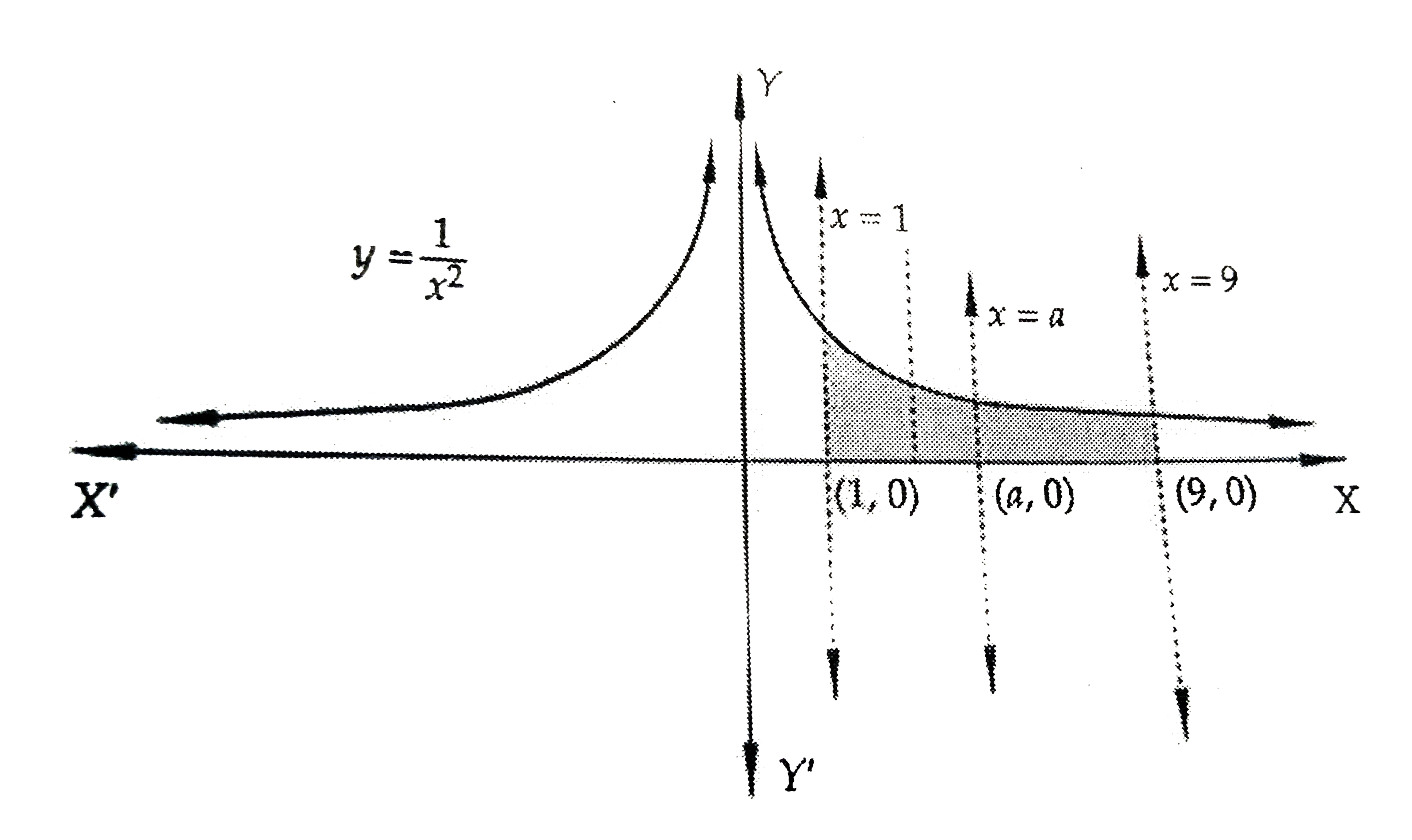
⇒2[−1x]a1=[−1x]91
⇒2(−1a+1)=(−19+1)
⇒−2a+2=−19+1⇒−2a=−109⇒a=95
|
Topper's Solved these Questions
- AREAS OF BOUNDED REGIONSBOOK - OBJECTIVE RD SHARMACHAPTER - AREAS OF BOUNDED REGIONSEXERCISE - Exercise
62 Videos
- AREAS OF BOUNDED REGIONSBOOK - OBJECTIVE RD SHARMACHAPTER - AREAS OF BOUNDED REGIONSEXERCISE - Chapter Test
60 Videos
- APPLICATION OF DERIVATIVESBOOK - OBJECTIVE RD SHARMACHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Illustration
6 Videos
- CARTESIAN PRODUCT OF SETS AND RELATIONS BOOK - OBJECTIVE RD SHARMACHAPTER - CARTESIAN PRODUCT OF SETS AND RELATIONS EXERCISE - Chapter Test
31 Videos
Similar Questions
Knowledge Check
Find the area bounded by the curves x2≤y≤x+2:
A112B72C92D52The area between the curves y=x2andy=x1/3,−1≤x≤1 is
A12B2C34D32The area bounded by the curve y=sin2x,0≤x≤π2, X axis and the line x=π2 is
Aπ4sq.unitsBπ2sq.unitsC3π2sq.unitsD3π4sq.units
Find the area between the x-axis and the curve y=√1+cos4x,0≤x≤π.
View SolutionThe area {(x,y) x2≤y≤√2} is equal to
View SolutionIf the line, y=mx bisects the area of the region {(x,y):0≤x≤32,0≤y≤1+4x−x2}, then m equals:
View SolutionThe area of the region {(x,y):x2+y2≤1≤x+y} , is
View SolutionIf the line x=α divides the area of region R={(x,y)∈R2:x3≤y≤x,0≤x≤1} into two equal parts, then
View Solution
