The area (in sqaure units) of the region ${(x, y) : x \geq 0, x + y \leq 3, x^{2} \leq 4 y and y \leq 1 + \sqrt{x}}$ is

A

$\frac{59}{12}$

B

$\frac{3}{2}$

C

$\frac{7}{3}$

D

$\frac{5}{2}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:d

Let A be the area of the shaded region. Then,

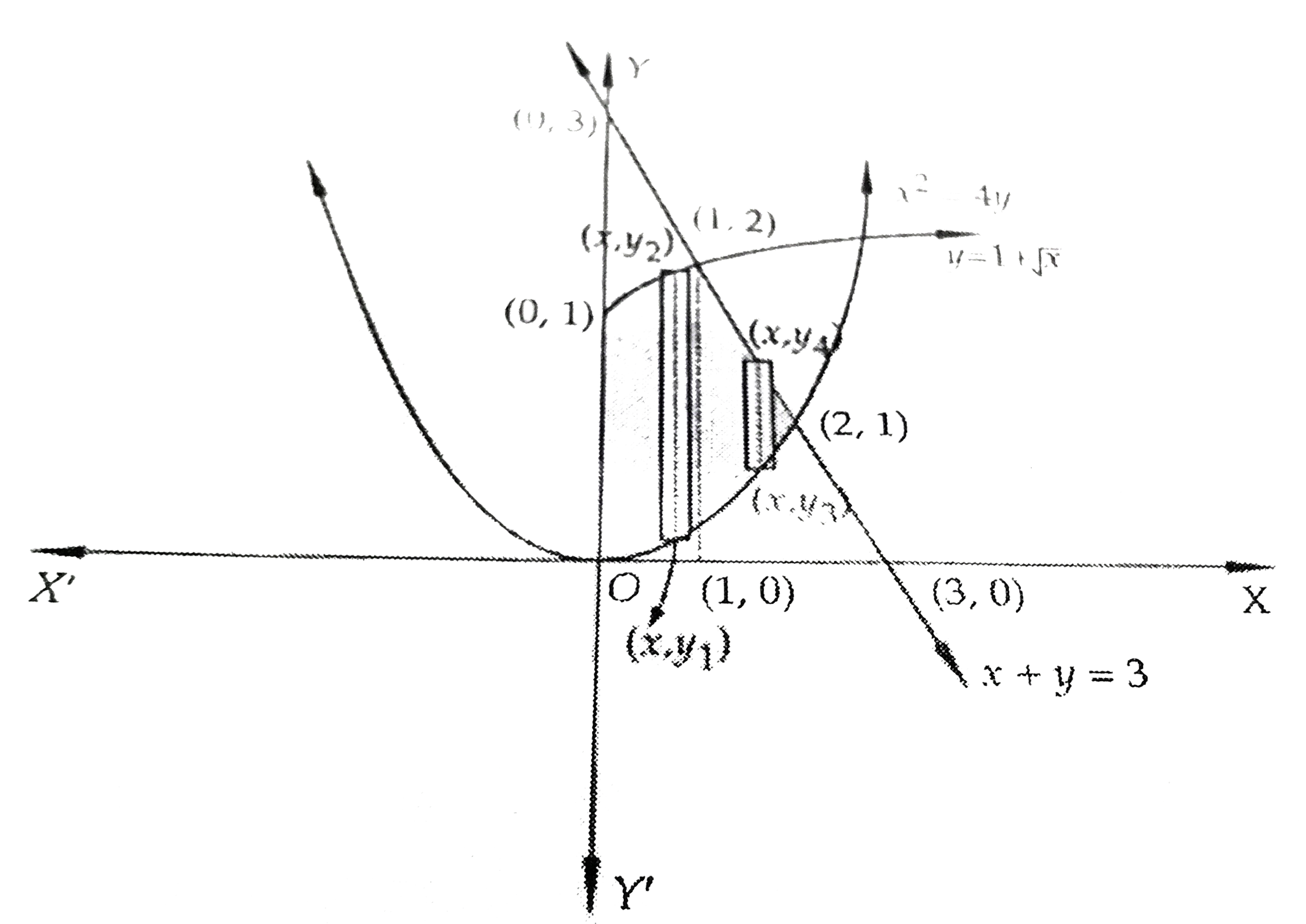
$A = 1 \int 0 (y_{2} - y_{1}) d x + 2 y_{4} - y_{3} 1 d x$
$\Rightarrow A = 1 \int 0 (1 + \sqrt{x} - \frac{x^{2}}{4}) d x + 2 \int 1 (3 - x - \frac{x^{2}}{4}) d x$
$\Rightarrow A = {[x + \frac{2}{3} x^{3 / 2} - \frac{x^{3}}{12}]}_{0}^{3 / 2} + {[3 x - \frac{x^{2}}{2} - \frac{x^{3}}{12}]}_{1}^{2}$
$A = \frac{19}{12} + \frac{10}{3} - \frac{29}{12} = \frac{5}{2}$

|

Updated on:21/07/2023

Class 12 MATHS APPLICATION OF INTEGRALS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The area ( in sq. units) of the region ${(x, y) : x \geq 0, x + y \leq 3, x^{2} \leq 4 y$ and $y \leq 1 + \sqrt{x}}$ is :
A $\frac{59}{12}$
B $\frac{3}{2}$
C $\frac{7}{3}$
D $\frac{5}{2}$
Question 2 - Select One
The area (in sq units) of the region $A = {(x, y) : x^{2} \leq y \leq x + 2}$ is
A $\frac{13}{6}$
B $\frac{9}{2}$
C $\frac{31}{6}$
D $\frac{10}{3}$
Question 3 - Select One
The area (in sq. units) of the region ${(x, y), x \geq 0, y \geq 0, y \geq x - 2, and y \leq \sqrt{x}}$ is
A $\frac{10}{3}$
B $\frac{13}{3}$
C $\frac{5}{3}$
D $\frac{8}{3}$

OBJECTIVE RD SHARMA-AREAS OF BOUNDED REGIONS-Chapter Test

Home
Ask Doubt
Profile