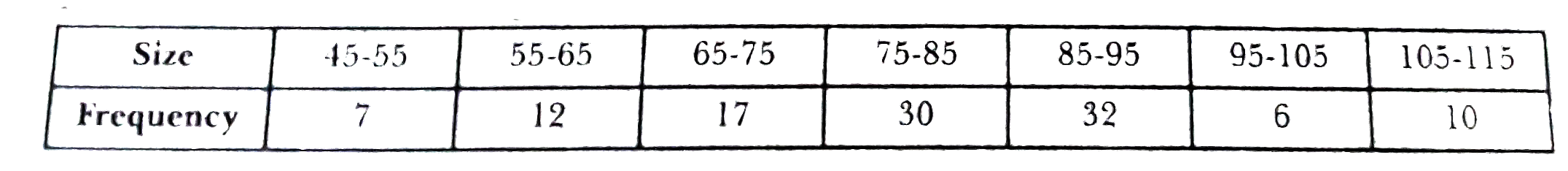
Mode of a certain series is x. If each score is decreased by 3, then mode of the new series is
x
x−3
x+3
3x
The correct Answer is:B
Step by step video, text & image solution for Mode of a certain series is x. If each score is decreased by 3, then mode of the new series is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MEASURES OF CENTRAL TENDENCYBOOK - OBJECTIVE RD SHARMACHAPTER - MEASURES OF CENTRAL TENDENCYEXERCISE - Exercise
40 Videos
- MAXIMA AND MINIMA BOOK - OBJECTIVE RD SHARMACHAPTER - MAXIMA AND MINIMA EXERCISE - Chapter Test
30 Videos
- MISCELLANEOUS EQUATIONS AND INEQUATIONS BOOK - OBJECTIVE RD SHARMACHAPTER - MISCELLANEOUS EQUATIONS AND INEQUATIONS EXERCISE - Chapter Test
30 Videos
Similar Questions
Knowledge Check
The arithmetic mean of a set of observations is ¯¯¯X. If each observation is divided by α and then is increased by 10, then the mean of the new series is
A→XαB→X+10αC→X+10ααDα→X+10The arithmetic mean of a set of observations is ¯¯¯X. If each observation is divided by α and then is increased by 10, then the mean of the new series is
A¯¯¯XαB¯¯¯X+10αC¯¯¯X+10ααDα¯¯¯X+10The number of letters skipped in between adjacent letters in the series decreases by one each time. Which of the following series observes the rule?
ACGKOSBDHLPTCEJNQSDBFJNR
Find the mode of the following series by grouping
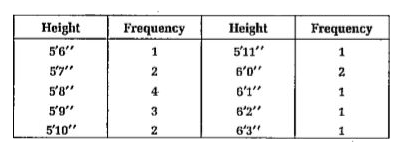
View SolutionThere can be more than one mode in a series.
View SolutionIf the sum of mode and mean of certain observations is 129 and the median of the observations is 63, then find mode and mean.
View SolutionIf the sum of the mode and mean of a certain frequency distribution is 129 and the median of the observations is 63, mode and median are respectively
View SolutionAn arithmetic series consists of 2n terms, and the first term equals the value of the common difference. If a new series is formed taking the 1st, 3rd, 5th,... (2n – 1) th term of the old series, find the ratio of the sum of the new series to that of the sum of the terms of the old series.
View Solution