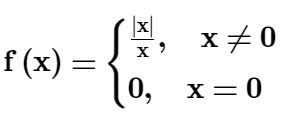If f(x)=xe[x]+|x|−2[x]+|x|, then limx→0f(x) is.
A
-1
B
0
C
1
D
non-existent
Video Solution
Text Solution
The correct Answer is:D
We have,
limx→0−f=(x)limx→0−xe−1−x−2−1−x[∵[x]=−1and|x|=−x for−1<x<0]
⇒limx→0−f(x)=0×e−1−2−1=0
and , limx→0+f(x)=limx→0x(e−x−2x)[∵[x]=0and|x|=xfor−1<x<0]
⇒limx→0+f(x)=limx→0ex−2=1−2=−1
∴limx→0f(x) does not exist.
|
Topper's Solved these Questions
- LIMITSBOOK - OBJECTIVE RD SHARMACHAPTER - LIMITSEXERCISE - Section I - Solved Mcqs
101 Videos
- LIMITSBOOK - OBJECTIVE RD SHARMACHAPTER - LIMITSEXERCISE - Section II - Assertion Reason Type
5 Videos
- INVERSE TRIGONOMETRIC FUNCTIONS BOOK - OBJECTIVE RD SHARMACHAPTER - INVERSE TRIGONOMETRIC FUNCTIONS EXERCISE - Chapter Test
30 Videos
- MATHEMATICAL INDUCTION BOOK - OBJECTIVE RD SHARMACHAPTER - MATHEMATICAL INDUCTION EXERCISE - Exercise
30 Videos
Similar Questions
Knowledge Check
If f(x)=sin(ex−2−)log(x−1) then limx→2f(x) is given by
A−2B−1C0D1If f(x)=tanxx−π, then limx→(π)f(x)=……………….
A1B0C−1Dnone of the aboveIf f(x)={xsin(1x)x≠00x=0 Then, limx→0f(x)
Ais equal to 1Bis equal to−1Cis equal to 0Ddoes not exist