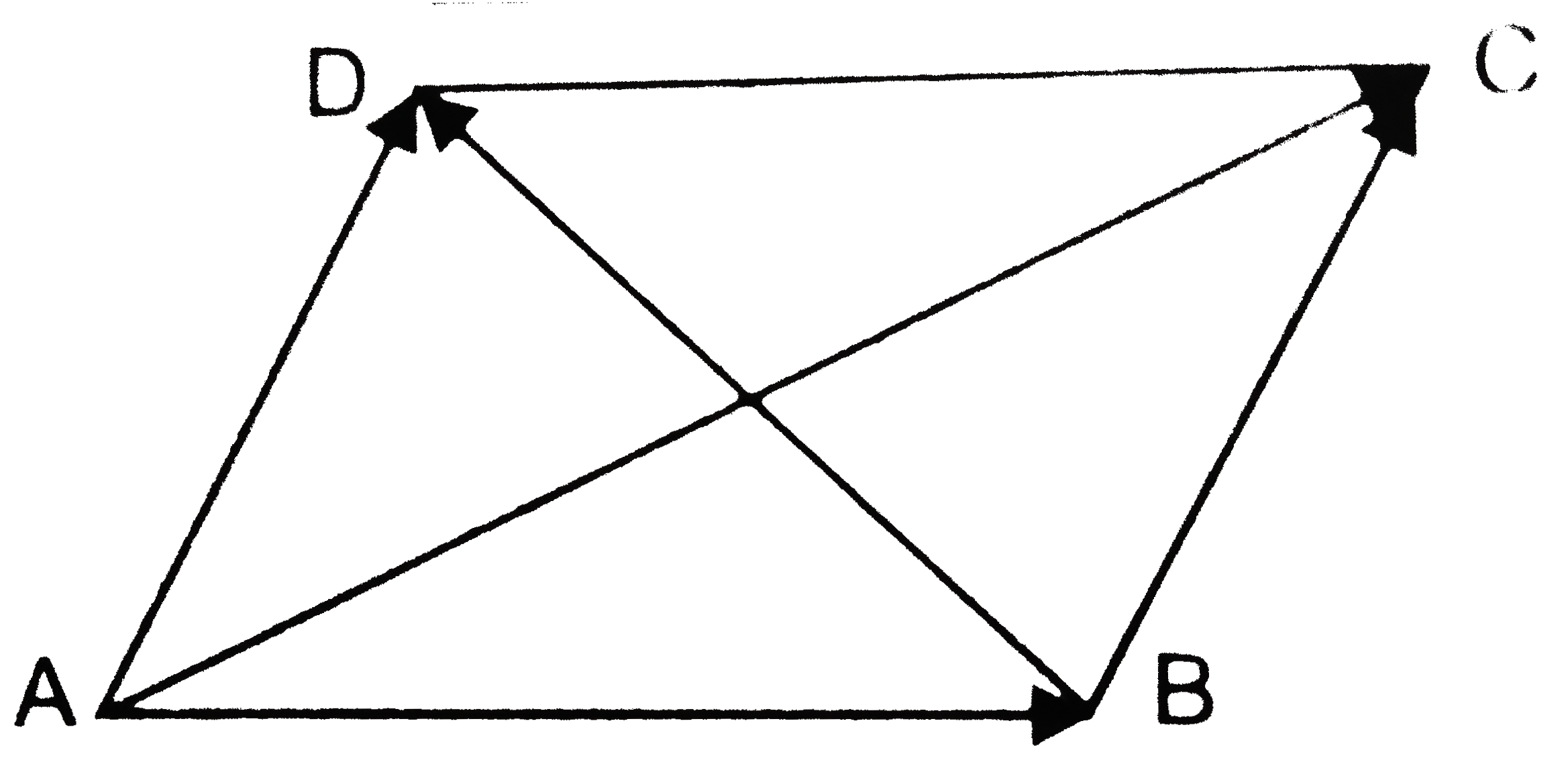
ABCD is a parallelogram with AC and BD as diagonals. Then, A→C−B→D=
4A→B
3A→B
2A→B
A→B
The correct Answer is:C
To solve the problem, we need to find the expression for →AC−→BD in the context of a parallelogram ABCD.
1. Identify the Vectors:
In a parallelogram, the diagonals bisect each other. We can express the vectors →AC and →BD in terms of the sides of the parallelogram.
- Let →A be the position vector of point A, →B for point B, →C for point C, and →D for point D.
- The vector →AC can be expressed as:
→AC=→C−→A
- The vector →BD can be expressed as:
→BD=→D−→B
2. Using the Properties of a Parallelogram:
In a parallelogram, we have:
→C=→A+→AB+→AD
→D=→B+→AD−→AB
However, we can simplify our approach by using the fact that →C=→A+→AB+→AD and →D=→A+→AD.
3. Express →AC−→BD:
Now substituting the expressions for →AC and →BD:
→AC−→BD=(→C−→A)−(→D−→B)
This simplifies to:
→AC−→BD=→C−→A−→D+→B
4. Substituting for →C and →D:
We know that →C=→A+→AB+→AD and →D=→B+→AD. Thus:
→AC−→BD=(→A+→AB+→AD−→A)−(→B+→AD−→B)
This leads to:
→AC−→BD=→AB−→AD
5. Final Simplification:
Since →AD=→BC in a parallelogram, we can conclude:
→AC−→BD=→AB+→BC−→BC=→AB
Thus, we find that:
→AC−→BD=2→AB
Final Answer:
→AC−→BD=2→AB
In a parallelogram, the diagonals bisect each other. We can express the vectors →AC and →BD in terms of the sides of the parallelogram.
- Let →A be the position vector of point A, →B for point B, →C for point C, and →D for point D.
- The vector →AC can be expressed as:
→AC=→C−→A
- The vector →BD can be expressed as:
→BD=→D−→B
In a parallelogram, we have:
→C=→A+→AB+→AD
→D=→B+→AD−→AB
However, we can simplify our approach by using the fact that →C=→A+→AB+→AD and →D=→A+→AD.
Now substituting the expressions for →AC and →BD:
→AC−→BD=(→C−→A)−(→D−→B)
This simplifies to:
→AC−→BD=→C−→A−→D+→B
We know that →C=→A+→AB+→AD and →D=→B+→AD. Thus:
→AC−→BD=(→A+→AB+→AD−→A)−(→B+→AD−→B)
This leads to:
→AC−→BD=→AB−→AD
Since →AD=→BC in a parallelogram, we can conclude:
→AC−→BD=→AB+→BC−→BC=→AB
→AC−→BD=2→AB
→AC−→BD=2→AB
Topper's Solved these Questions
- ALGEBRA OF VECTORSBOOK - OBJECTIVE RD SHARMACHAPTER - ALGEBRA OF VECTORSEXERCISE - Exercise
30 Videos
- ALGEBRA OF VECTORSBOOK - OBJECTIVE RD SHARMACHAPTER - ALGEBRA OF VECTORSEXERCISE - Chapter Test
30 Videos
- ALGEBRAIC INEQUATIONSBOOK - OBJECTIVE RD SHARMACHAPTER - ALGEBRAIC INEQUATIONSEXERCISE - Exercise
39 Videos
Similar Questions
Knowledge Check
ABCD is a quadrlateral in which BD and AC are diagonals then
AAB+BC+CD+AD<AC+BDBAB+BC+CD+DA>AC+BDCAB+BC+CD+DA=AC+BDDAB+BC+CD+DA>2(AC+BD)If ABCD is a parallelogram, then −−→AC−−−→BD=
A4−−→ABB3−−→ABC2−−→ABD−−→ABIf G is the intersection of diagonals of a parallelogram ABCD and O is any point, then O→A+O→B+O→C+−−→OD=
A2−−→OGB4−−→OGC5−−→OGD3−−→OG
ABCD is a parallelogram where AC and BD are the diagonals. If ∠BAD=60∘,∠ABD=90∘, then what is BD2 equal to ?
View SolutionABCD is a parallelogram and AC, BD are its diagonals. Show that :
−−→AC+−−→BD=2−−→BC,−−→AC−−−→BD=2−−→AB.
View SolutionABCD is a parallelogram Fig. 2 (c ) .64. ACand(BD)areitsdiagonals.Showtˆ<br> (a) vec (AC) +vec (BD) =2 vec (BC)<br>(b)vec (AC) - vec (BD) =2 vec (AB)
 .
.
View SolutionIf →aand→b represent two adjacent sides →ABand→BC respectively of a parallelogram ABCD, then show that its diagonals →ACand→DB are equal to →a+→b and →a−→b respectively.
View SolutionIf G is the intersection of diagonals of a parallelogram ABCD and O is any point, then O→A+O→B+O→C+−−→OD=
View Solution
